A particle moves along the straight line y=3x+5. Which coordinate changes at faster rate?
PV
Pb
aV2
abV2
The correct Answer is:C
To solve the problem of which coordinate changes at a faster rate for a particle moving along the straight line given by the equation y=3x+5, we will follow these steps:
Step 1: Understand the relationship between x and y
The equation of the line is given as:
y=3x+5
This indicates that y is a linear function of x.
Step 2: Differentiate both sides with respect to time t
Assuming both x and y are functions of time t, we differentiate the equation with respect to t:
dydt=ddt(3x+5)
Using the chain rule, we differentiate the right-hand side:
dydt=3dxdt+0
The term 0 comes from the derivative of the constant 5.
Step 3: Simplify the equation
From the differentiation, we have:
dydt=3dxdt
Step 4: Analyze the rates of change
This equation shows that the rate of change of y with respect to t is three times the rate of change of x with respect to t:
dydt=3dxdt
This means that for every unit change in x, y changes three units.
Step 5: Conclusion
Since dydt is greater than dxdt, we conclude that the y coordinate changes at a faster rate than the x coordinate.
Final Answer:
The y coordinate changes at a faster rate.
---
The equation of the line is given as:
y=3x+5
This indicates that y is a linear function of x.
Assuming both x and y are functions of time t, we differentiate the equation with respect to t:
dydt=ddt(3x+5)
Using the chain rule, we differentiate the right-hand side:
dydt=3dxdt+0
The term 0 comes from the derivative of the constant 5.
From the differentiation, we have:
dydt=3dxdt
This equation shows that the rate of change of y with respect to t is three times the rate of change of x with respect to t:
dydt=3dxdt
This means that for every unit change in x, y changes three units.
Since dydt is greater than dxdt, we conclude that the y coordinate changes at a faster rate than the x coordinate.
The y coordinate changes at a faster rate.
Topper's Solved these Questions
- BASIC MATHSBook:ALLENChapter:BASIC MATHSExercise:Comprehension 2
Explore 3 Videos
- BASIC MATHSBook:ALLENChapter:BASIC MATHSExercise:Comprehension 3
Explore 3 Videos
- BASIC MATHSBook:ALLENChapter:BASIC MATHSExercise:ASSERTION-REASON
Explore 11 Videos
- AIIMS 2019Book:ALLENChapter:AIIMS 2019Exercise:PHYSICS
Explore 40 Videos
- CIRCULAR MOTIONBook:ALLENChapter:CIRCULAR MOTIONExercise:EXERCISE (J-A)
Explore 6 Videos
Similar Questions
A particle moves along the curve 12y=x3. . Which coordinate changes at faster rate at x=10?
View SolutionA particle moves along a straight line on y-axis. The distance of the particle from O varies with time and is given by : y=20t−t2. The distance travelled by the particle before it momentarily comes to rest is
View SolutionA particle moves along the curve x2=2y. At what point, ordinate increases at the same rate as abscissa increases ?
View SolutionA particle moves along a straight line OX. At a time t (in seconds) the distance x (in metre) of the particle is given by x=40+12t−t3. How long would the particle travel before coming to rest ?
View SolutionA particle moves along a straight line OX. At a time t(in second), the distance x (in metre) of the particle from O is given by
x=40+12t−t3
How long would the particle travel before coming to rest ?
View SolutionA particle moves along a straight line such that its displacement at any time t is given by s=3t3+7t2+14t+5. The acceleration of the particle at t=1s is
View SolutionThe figure shows velocity-time graph of a particle moving along a straight line. Identify the correct statement.
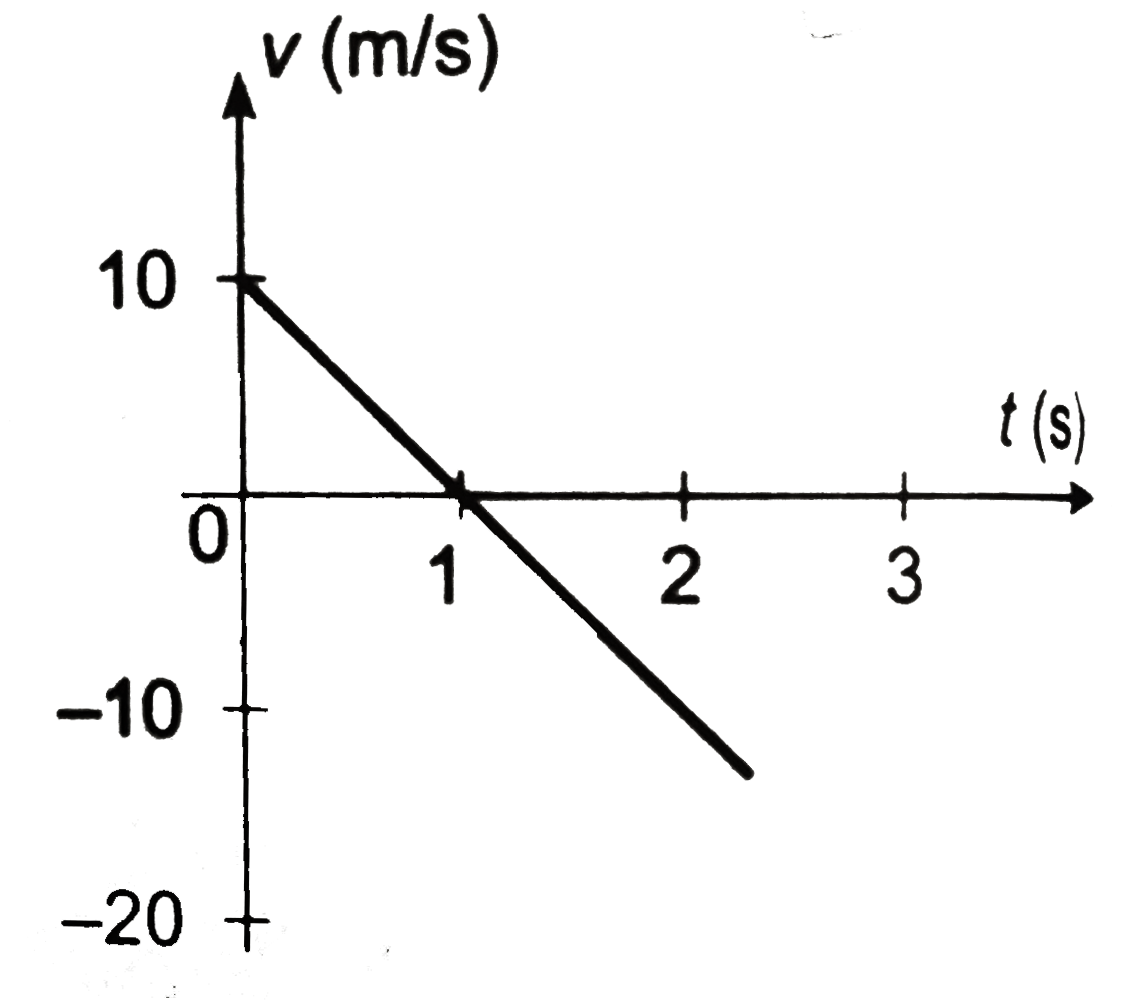
View SolutionIf a particle is moving along straight line with increasing speed, then
View SolutionA particle moves in straight line. Acceleration of particle changes with velocity as showns in graph:
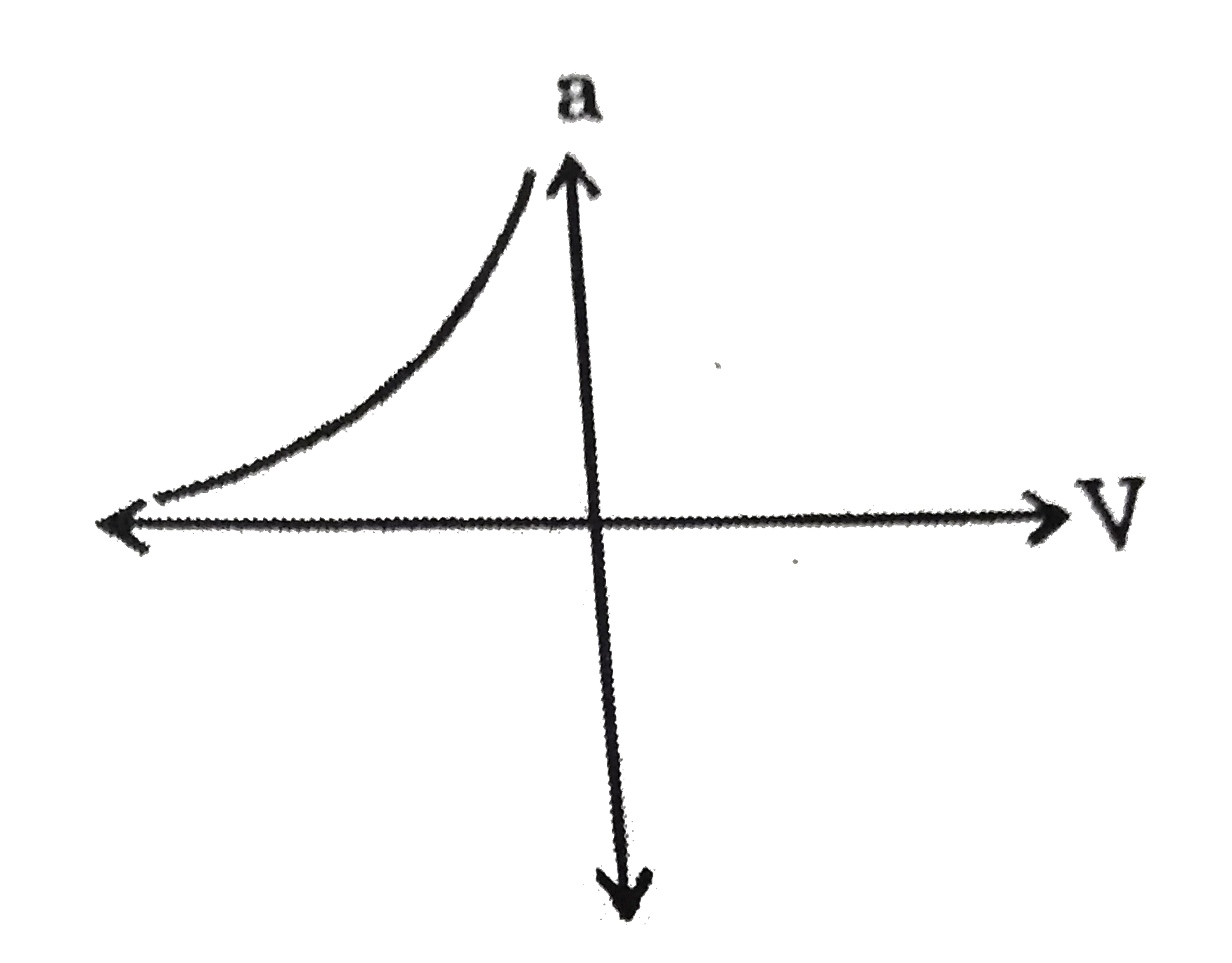
View SolutionA particle moves along a straight line and its position as a function of time is given by x=t6(3)−3t6(2)+3t+3, then particle
View Solution
