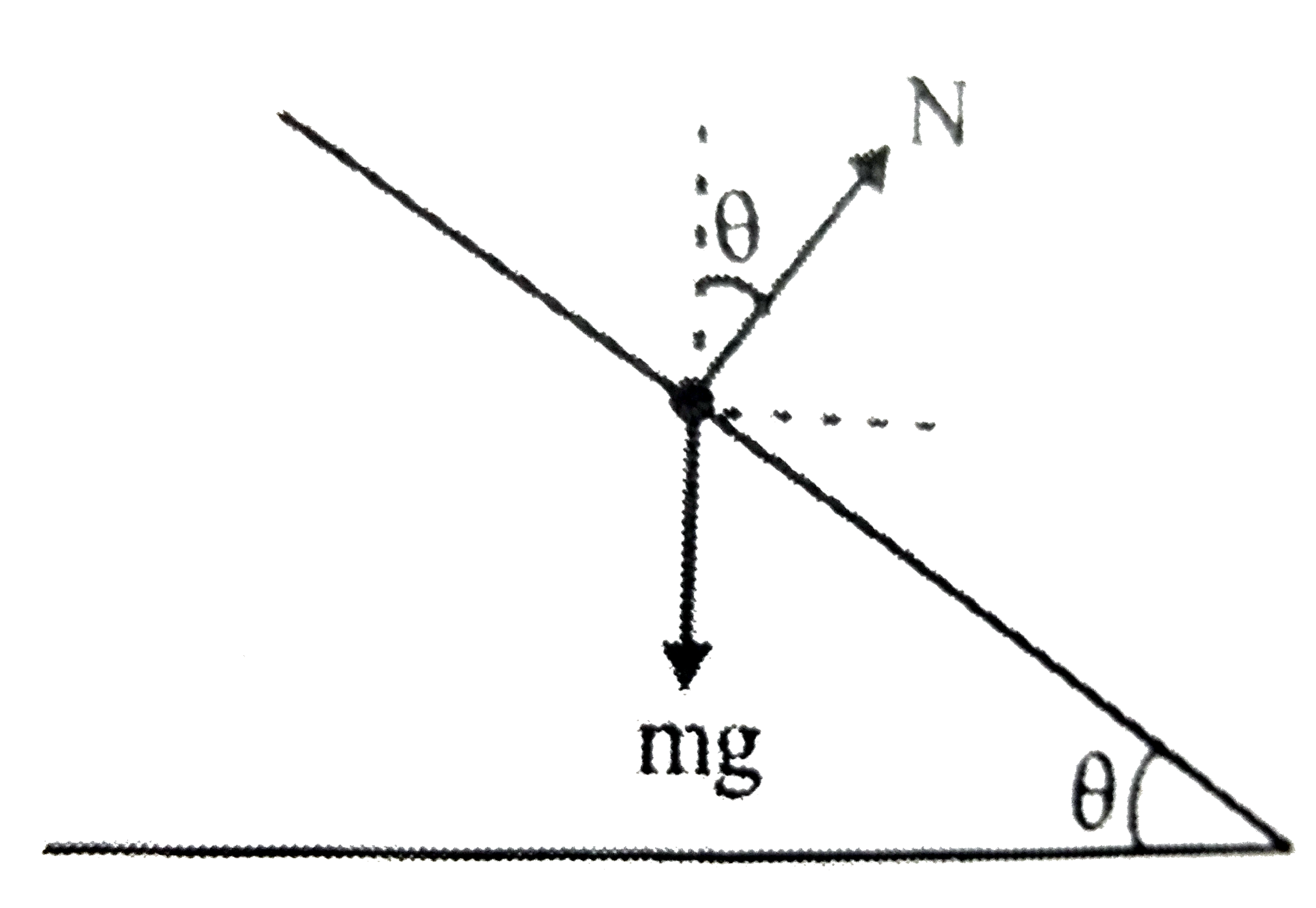
A car is moving along a banked road laid out as a circle of radius r . (a) What should be the banking angle $θ$ so that the car travelling at speed v needs no frictional force from tyres to negotiate the turn ? (b) The coefficient of friction between tyres and road are $μ_{s} = 0.9$ and $μ_{k} = 0.8$ . At what maximum speed can a car enter the curve without sliding toward the top edge of the banked turn ?

Video Solution

Text Solution

Verified by Experts

$N sin θ = \frac{m v^{2}}{r} and N cos θ = m g \Rightarrow tan θ = \frac{v^{2}}{r g}$
NOTE: In above case friction does not play any role in negotiating the turn.
(b) If the driver moves faster than the speed mentioned above, a friction force myst act parallel to the road, inward towards center of the turn.
$\Rightarrow F cos θ + N sin θ = \frac{m v^{2}}{r} and N cos θ = m g + f sin θ$
For maximum speed of f= $μ N$
$N (μ cos θ + sin θ) = \frac{m v^{2}}{r} and N (cos θ - μ sin θ) = m g$
$= \frac{v^{2}}{r g} = \frac{sin θ + μ cos θ}{cos θ - μ sin θ} \Rightarrow v = \sqrt{(\frac{sin θ + μ cos θ}{cos θ - μ sin t h e a})} r g$

Updated on:21/07/2023