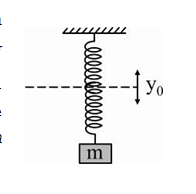
A mass m is undergoing SHM in the vertical direction about the mean position Y0 with amplitude A and angular frequency omega. At a distance y* from the mean position, the mass detaches from the spring. Assume that the spring contracts and does not obstruct the motion of m. Find the distance y⋅ (measured from the mean position) such that the height h attained by the block is maximum. Aω2>g__________
The correct Answer is:mgk=gω2<a
A small body of mass attached to one end of a vertically hanging spring performs SHM.
Angular frequency =ω
Amplitude =a
Under SHM, velocity
v=ω√a20−y2
After detaching from spring net downward accelration of the block =g.
∴ Height attained by the block =h
∴h=y+v22g⇒h=y+ω2(a2−y2)2g
For h tq be maximum
dhdy=0,y=y∗
∴dhdy=1+ω22g(−2y∗)⇒0=1−2ω2y∗2g
⇒ω2y∗g=1⇒y∗=gω2
Since aω2>g (given)
∴a>gω2∴a>y∗.y∗
from mean position <a
Hence y∗=gω2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Solved Example
3 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - X Rays : Solved Example
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Paragraph
3 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A mass m is undergoing SHM in the verticl direction about the mean position y0 with amplitude A and anglular frequency ω. At a distance y form the mean position, the mass detached from the spring. Assume that th spring contracts and does not obstruct the motion of m. Find the distance y. (measured from the mean position). such that height h attained by the block is maximum (Aω2>g).
_E01_175_Q01.png)
View SolutionA block is performin SHM of amplitude 'A' in vertical direction. When block i8s at 'y' (measured from mean position), it detaches froms spring, so that spring contracts and does not effect the motion of the block. Find 'y⋅' such that block attains maximum height from the mean position. (given Aω2>g)
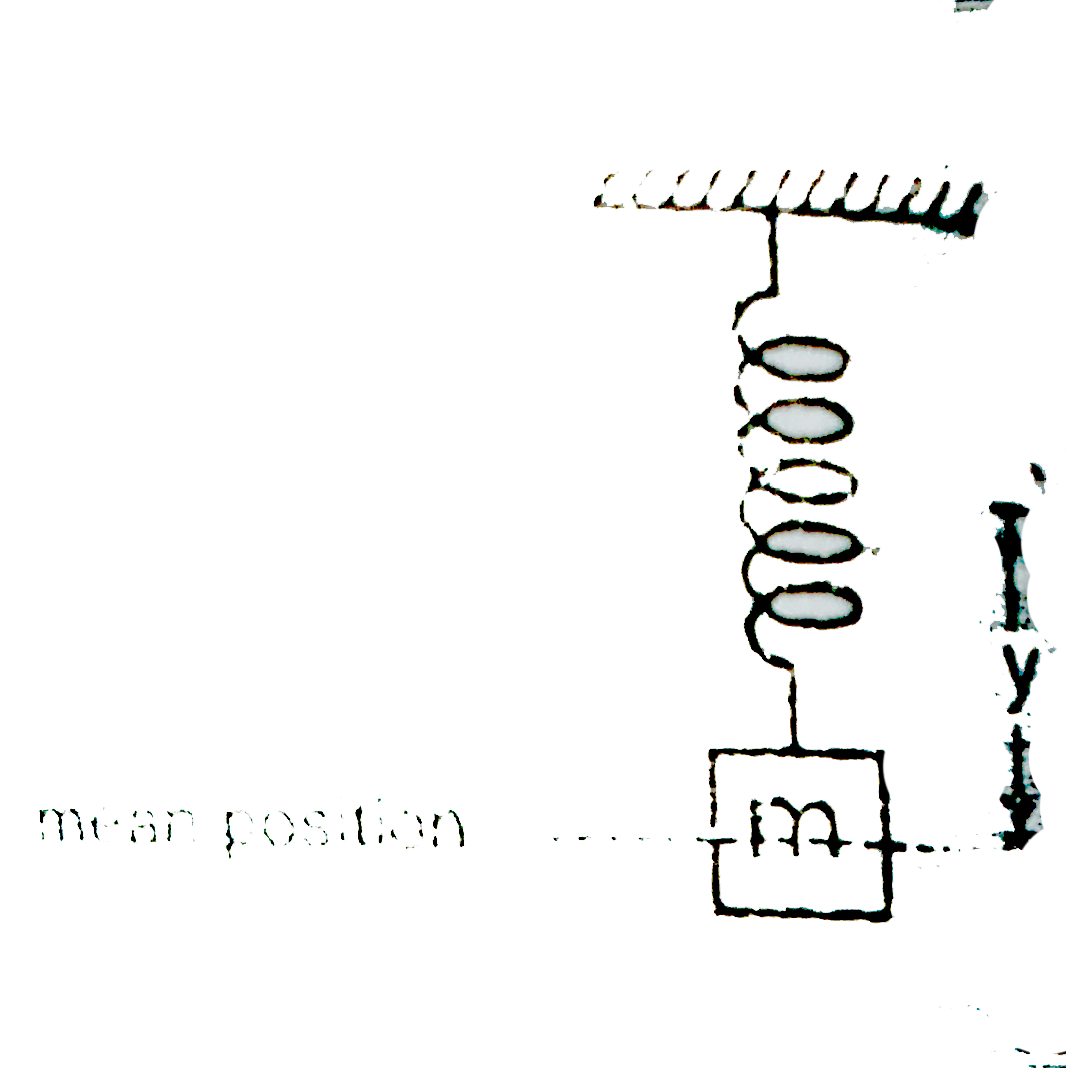
View SolutionIn a S.H.M. with amplitude 'A', what is the ratio of K.E. and P.E. at A2 distance from the mean position ?
View SolutionThe equation of a S.H.M. of amplitude A and angular frequency ω in which all distances are measured from one extreme position and time is taken to be zero at the other extreme position is
View SolutionA partical excutes SHM with amplitudeA and angular frequency ω. At an instant the particle is at a distance A/5 from the mean position and is moving away from it. Find the time after which it will come back to this position again and also find the time after which it will pass through the mean position.
View Solutionfind the potential energy of the block at 0.05 m from the mean position
View SolutionThe amplitude and the periodic time of a S.H.M. are 5 cm and 6 sec respectively. At a distance of 2.5 cm away from the mean position, the phase will be
View SolutionFor examples , find the potential energy of the block at 0.05 m from the mean position
View SolutionA particle performing SHM with amplitude 10cm. At What distance from mean position the kinetic energy of the particle is thrice of its potential energy ?
View SolutionA particle executed S.H.M. starting from its mean position at t=0, If its velocity is √3bω, when it is at a distance b from the mean positoin, when ω=2πT, the time taken by the particle to move from b to the extreme position on the same side is
View Solution
