X1 and X2 are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X(X1X<X2X) then particle crosses this point again at successive intervals of 2s and 4s with a speed of 5m/s.
The time period of SHM is
6s
3s
4s
2s
The correct Answer is:A
To find the time period of the simple harmonic motion (SHM) for the given problem, we can follow these steps:
Step 1: Understand the Problem
We have two points X1 and X2 where the particle's velocity is zero. The particle crosses a certain point X (where X1<X<X2) at intervals of 2 seconds and 4 seconds with a speed of 5 m/s.
Step 2: Identify the Time Intervals
The particle takes:
- 2 seconds to move from X to X1 (and back to X),
- 4 seconds to move from X to X2 (and back to X).
Step 3: Determine the Total Time for One Complete Cycle
The total time taken for the particle to go from X1 to X2 and back to X1 is the sum of the time intervals:
- Time from X to X1 and back to X: 2 seconds (up and down)
- Time from X to X2 and back to X: 4 seconds (up and down)
Thus, the total time for one complete cycle (from X1 to X2 and back to X1) is:
T=2+4=6 seconds
Step 4: Conclusion
The time period T of the simple harmonic motion is 6 seconds.
Final Answer
The time period of SHM is 6 seconds.
---
We have two points X1 and X2 where the particle's velocity is zero. The particle crosses a certain point X (where X1<X<X2) at intervals of 2 seconds and 4 seconds with a speed of 5 m/s.
The particle takes:
- 2 seconds to move from X to X1 (and back to X),
- 4 seconds to move from X to X2 (and back to X).
The total time taken for the particle to go from X1 to X2 and back to X1 is the sum of the time intervals:
- Time from X to X1 and back to X: 2 seconds (up and down)
- Time from X to X2 and back to X: 4 seconds (up and down)
T=2+4=6 seconds
The time period T of the simple harmonic motion is 6 seconds.
The time period of SHM is 6 seconds.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-01
117 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. The ratio AXBX is
View SolutionA and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. Amplitude of oscillation is
View SolutionA particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(APltBP) at successive intervals of 0.5s and 1.5 s with a speed of 3m/s.
View SolutionThe displacement time graph of a particle executing S.H.M. (in straight line) is shown. Which of the following statements is true?
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
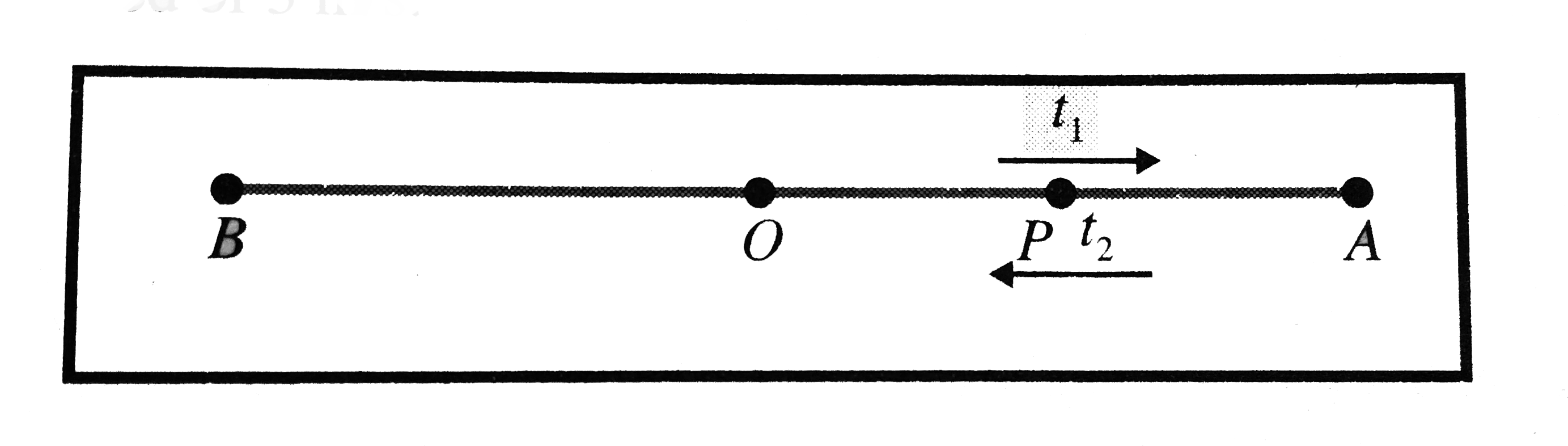
View SolutionA particle starts SHM from mean position O executing SHM A and B are the two point at which its velocity is zero . It passes through a certain point P at time t1=0.5andt2=1.5s with a speed of 3m/s.
i. The maximum speed .......
ii. ratio AP/PB ....
 .
.
View SolutionDisplacement of a particle executing SHM s x=10(cosπt+sinπt). Its maximum speed is
View SolutionThe maximum velocity of a particle, executing SHM with an amplitude 7 mm is 4.4 m/s. the period of oscillation is
View SolutionThe particle is executing SHM on a line 4 cm long. If its velocity at mean position is 12 m/s , then determine its frequency.
View Solution
