A point source of light is placed on the principal axis between F and 2F of a concave lens. On the other very far , a screen is placed perpendicular to principal axis. As the screen is brought close towards lens
the light intensity on screen continuously decreases
the light intensity on screen continuously increases
the light intensity on screen first increases, then decreases
the light intensity on screen first decreases, then increases
The correct Answer is:B
To solve the problem, we need to analyze the behavior of light as it passes through a concave lens when a point source of light is placed between F (the focal point) and 2F (twice the focal length).
1. Understanding the Setup:
- We have a concave lens, which is a diverging lens.
- A point source of light is placed on the principal axis between the focal point (F) and twice the focal point (2F).
- A screen is placed very far from the lens, perpendicular to the principal axis.
2. Behavior of Light through a Concave Lens:
- When light rays emanate from the point source, they pass through the concave lens.
- The concave lens diverges these rays, making them appear to come from a virtual image located on the same side as the object (the point source).
3. Position of the Virtual Image:
- Since the point source is between F and 2F, the virtual image formed by the concave lens will be located on the same side as the object (the point source) and will be virtual and upright.
4. Moving the Screen Closer:
- As the screen is brought closer to the lens, it intercepts more diverging rays from the lens.
- Since the concave lens diverges the rays, the intensity of light on the screen increases as more rays are captured by the screen.
5. Conclusion:
- Therefore, as the screen is brought closer to the lens, the intensity of light on the screen continuously increases.
Final Answer:
The intensity of light on the screen continuously increases as the screen is brought closer to the concave lens.
- We have a concave lens, which is a diverging lens.
- A point source of light is placed on the principal axis between the focal point (F) and twice the focal point (2F).
- A screen is placed very far from the lens, perpendicular to the principal axis.
- When light rays emanate from the point source, they pass through the concave lens.
- The concave lens diverges these rays, making them appear to come from a virtual image located on the same side as the object (the point source).
- Since the point source is between F and 2F, the virtual image formed by the concave lens will be located on the same side as the object (the point source) and will be virtual and upright.
- As the screen is brought closer to the lens, it intercepts more diverging rays from the lens.
- Since the concave lens diverges the rays, the intensity of light on the screen increases as more rays are captured by the screen.
- Therefore, as the screen is brought closer to the lens, the intensity of light on the screen continuously increases.
The intensity of light on the screen continuously increases as the screen is brought closer to the concave lens.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE-2
Explore 44 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE-3
Explore 12 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE - 05 (B) (ASSERTION & REASON )
Explore 9 Videos
- CURRENT ELECTRICITYBook:ALLENChapter:CURRENT ELECTRICITYExercise:EX.II
Explore 66 Videos
- GRAVITATIONBook:ALLENChapter:GRAVITATIONExercise:EXERCISE 4
Explore 9 Videos
Similar Questions
An extended object of size 2mm is placed on the principal axis of a converging lens of focal length 10cm. It is found that when the object is placed perpendicular to the principal axis the image formed is 4mm in size. The size of image when it is placed along the principal axis is ____________ mm.
View SolutionThe image of an exend object placed perpendicular to the principal axis of a mirror, will be erect if
View SolutionIf a equiconvex lens of focal length f is cut into two halves by a plane perpendicular to the principal axis, then
View SolutionA converging lens of focal length 15 cm and a converging mirror of focal length 20 cm are placed with their principal axes coinciding. A point source S is placed on the principal axis at a distance of 12 cm from the lens as shown in figure. It is found that the final beam comes out parallel to the principal axis. Find the separation between the mirror and the lens.
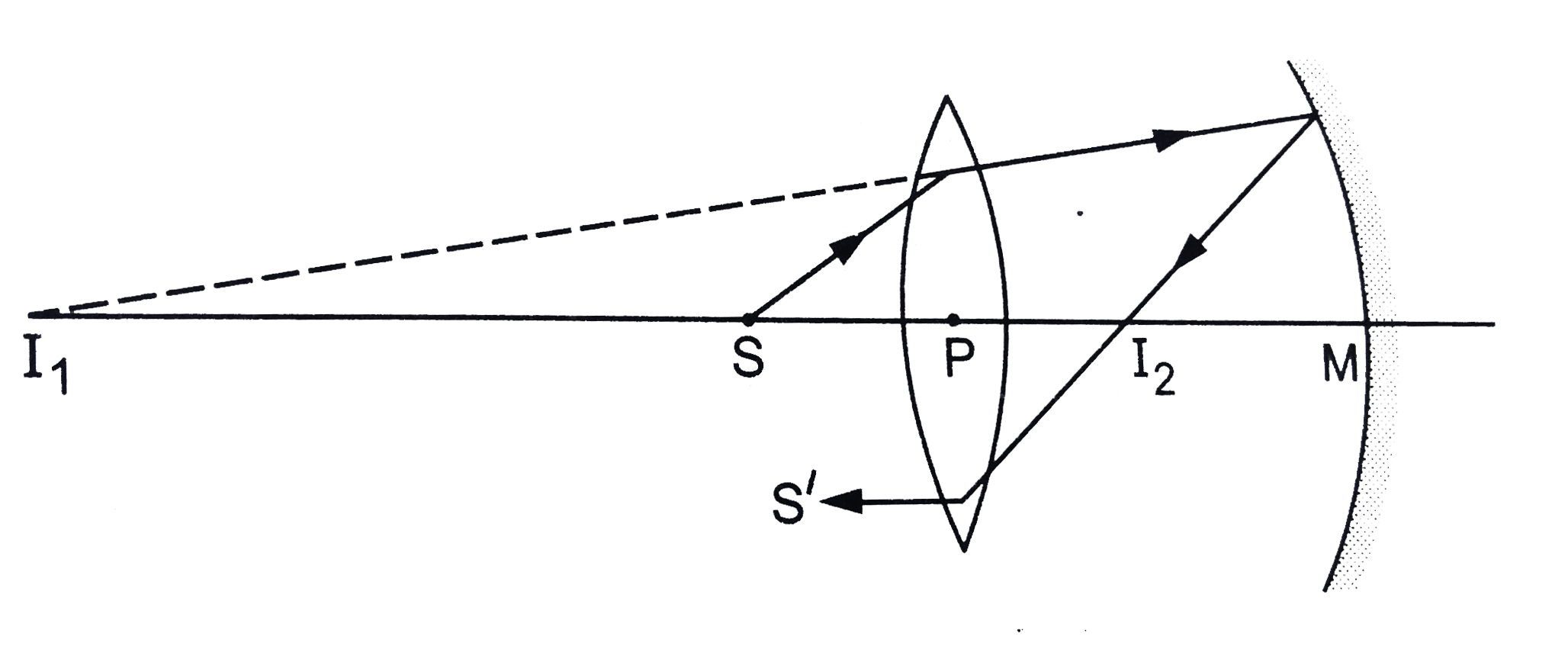
View SolutionA converging lens and a converging mirror are placed with their principal axis coinciding. Their separation equals 40cm. A point source S is placed on the principal axis at a distance of 12 cm from the lens as shown in Fig. It is found that the final beam comes out parallel to the principal axis. Focal length of the lens equals 15 cm. Find the focal length of the mirror.
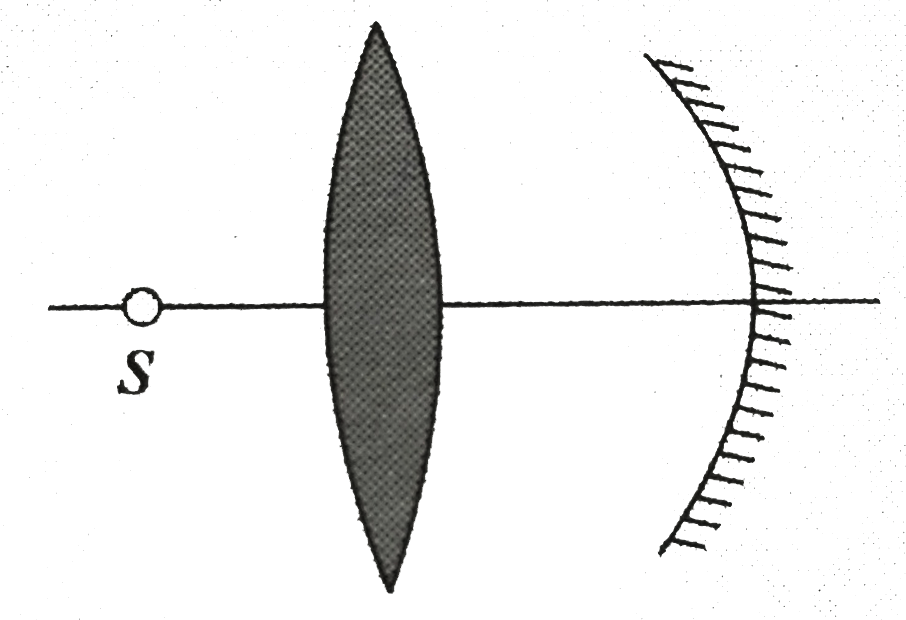
View SolutionA point object O is placed on the principal axis of a convex lens of focal length 10 cm at 12 cm from the lens. When object is displaced 1mm along the principal axis magnitude of displacement of image is x1. When the lens is displaced by 1mm perpendicular to the principal axis displacement of image is x2 in magnitude. find the value of x1x2
View SolutionA point source of light S is placed on the axis of a lens of focal length 20cm at a distance 25cm from lense. A screen is placed normal to the axis of lens at a distance x from it (treat all rays as paraxial). Identify the true or false statements.
a. As x is increased from zero , intensity continuously decreases.
b. As x is increased from zero, intensity first increases then decreases.
c. Intensity at center of screen for x=90cm and x=110cm is same.
View SolutionA point source of light is placed at a distance of 2f from a converging lens of focal length f. The intensity onteh other side of the lens is maximum at a distance
View SolutionAn object is placed on principal axis of concave mirror at distance of 1.5 f Image will be at
View SolutionA point object on the principal axis at a distance 1.5 cm in front of concave mirror of radius of curvature 20 cm has velocity 2mm/s is perpendicular to the principal axis. The velocity of image at that instant will be
View Solution