Helium gas goes through a cycle ABCDA (consisting of two isochoric and two isobaric lines) as shown in figure. Efficiency of this cycle is nearly :
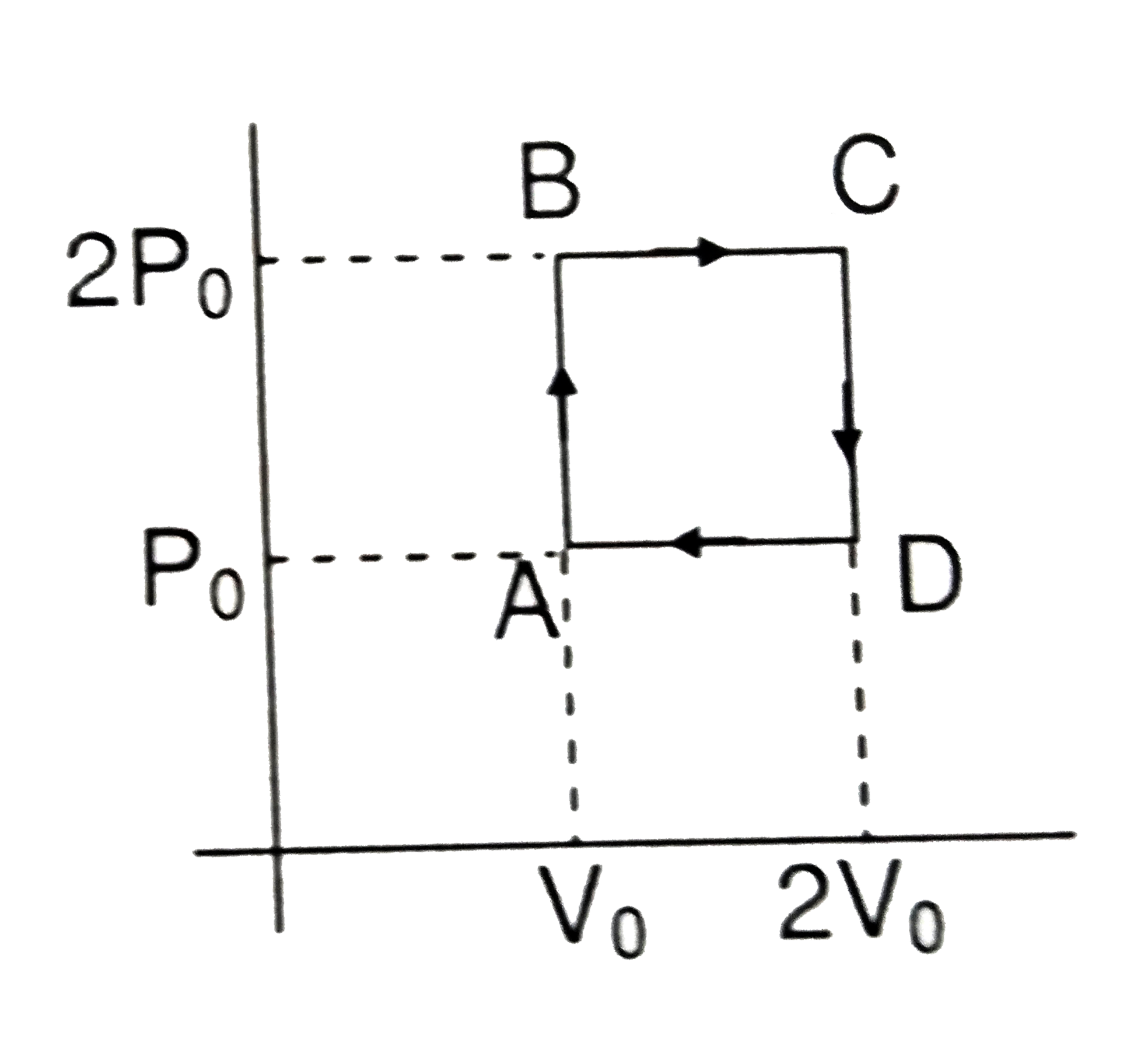
The correct Answer is:B
= Area bounded by curve =
Total heat supplied =
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (B)
58 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (B) (MCQ)
9 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (B)
15 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)

View SolutionHelium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)

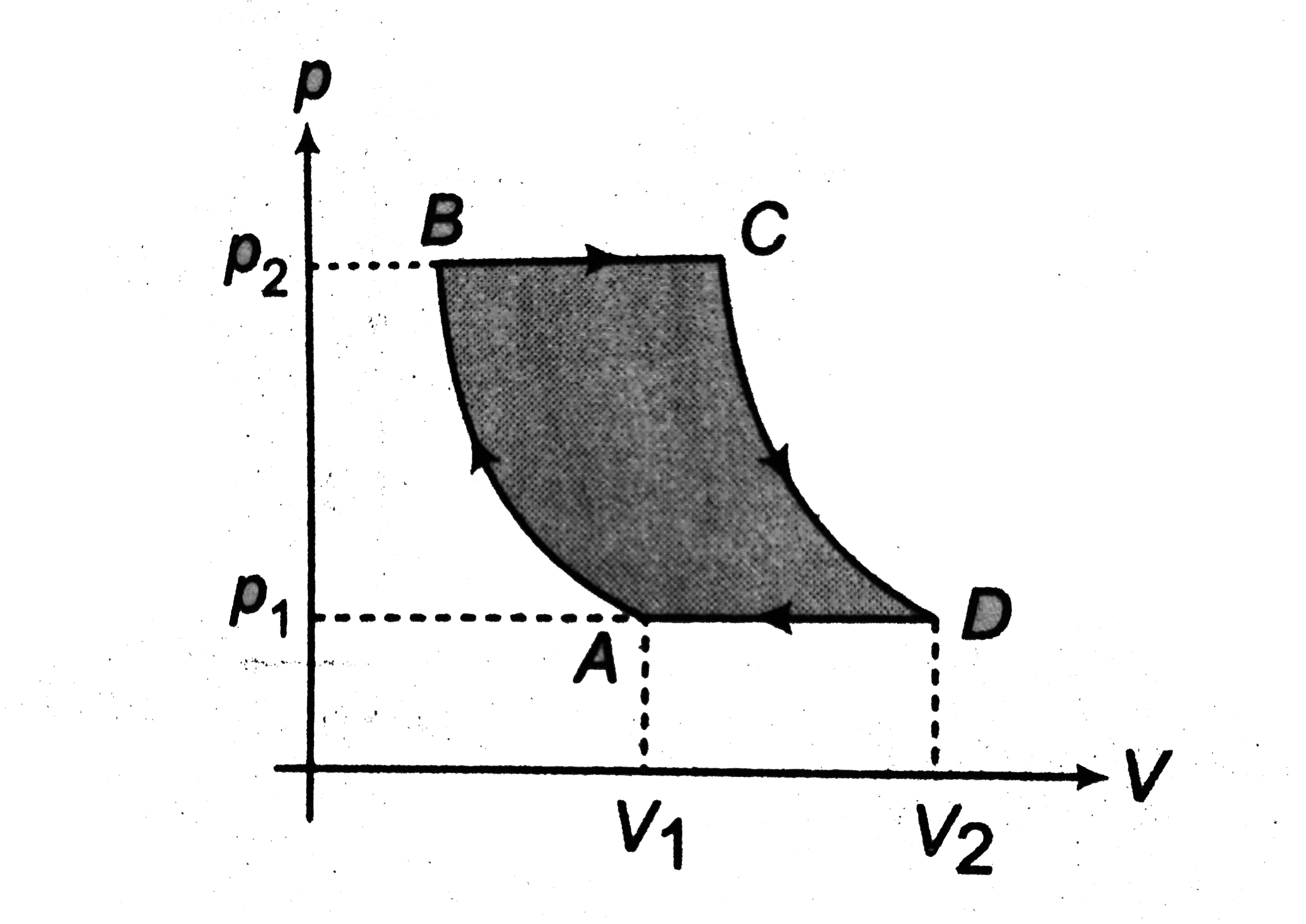
View SolutionAn ideal gas is carried through a thermodynamic cycle consisting of two isobaric and two isothermal processes as shown in figure. Show that the net work done in the entire cycle is given by the equation. Wnet=p1(V2−V1)Inp2p1
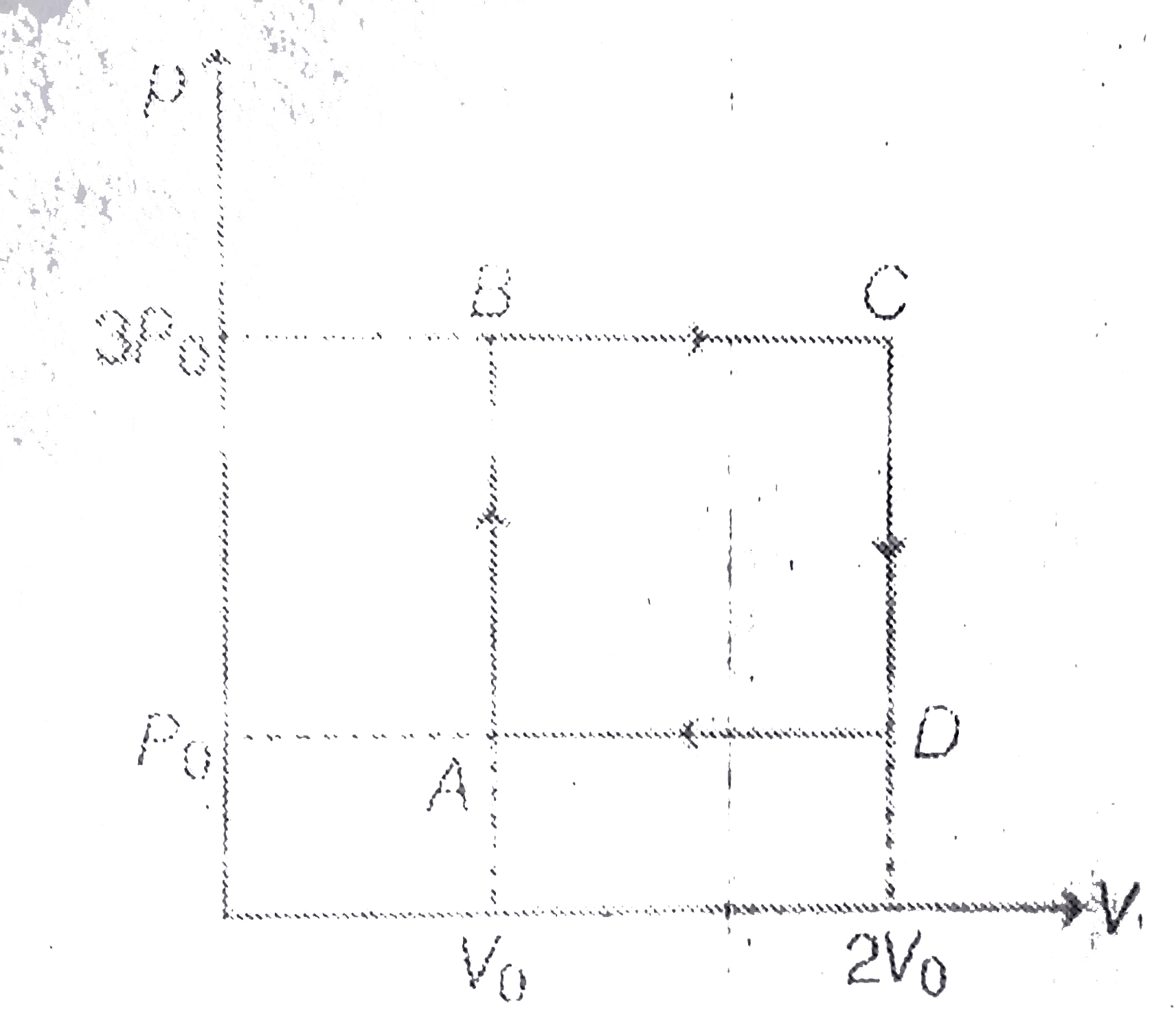
View SolutionAn ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the gas cycle is

View SolutionAn ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the cycle is ltBrgt
View SolutionA monoatomic gas undergoes a cycle consisting of two isothermals and two isobarics. The minimum and maximum temperatures of gas during the cycle are T1=400K and T2=800K, respectively, and the ratio of maximum to minimum volume is 4.
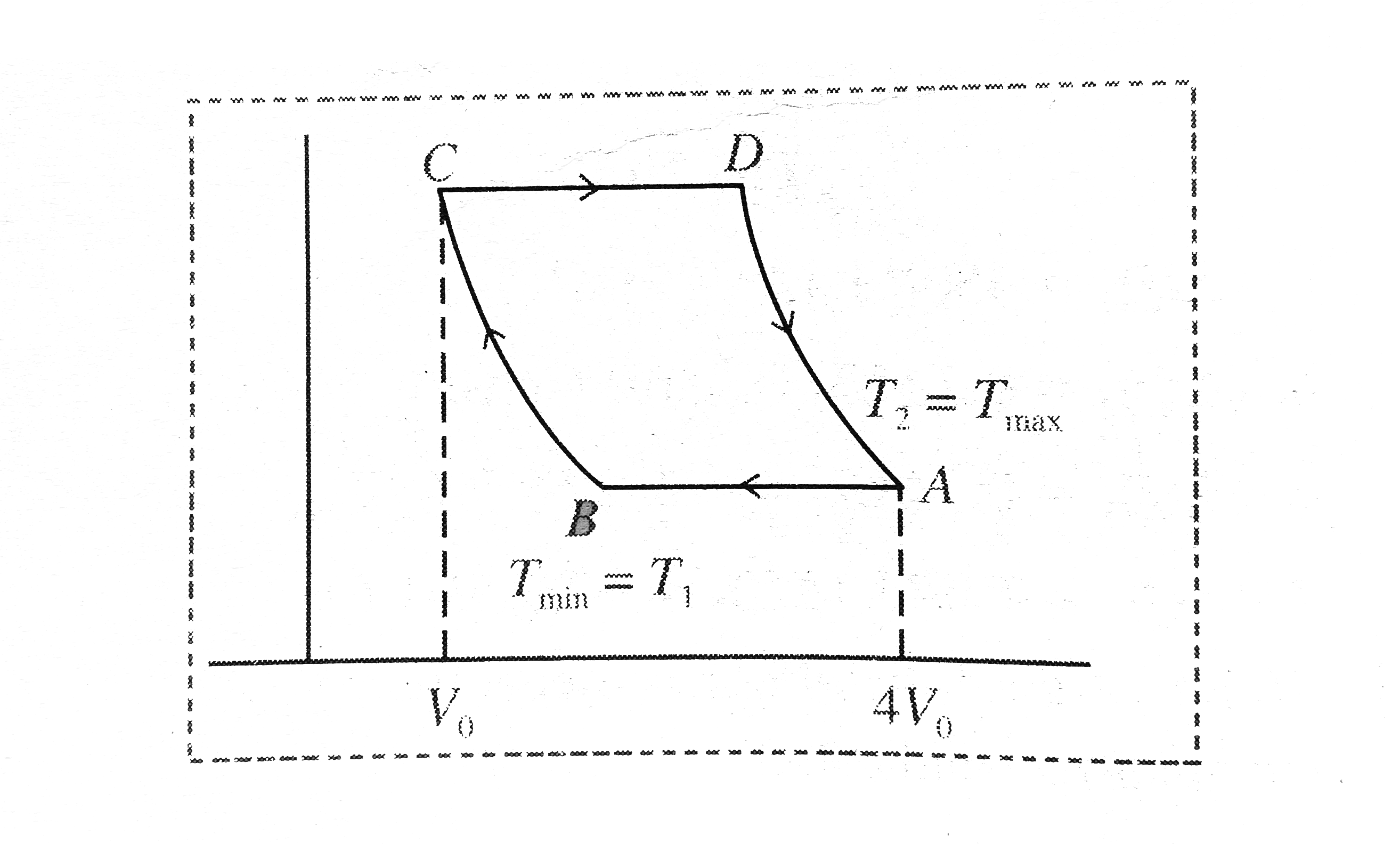
The efficiency of the cycle is
View SolutionA monatomic gas undergoes a cycle consisting of two isothermals and two isobarics. The minimum and maximum temperatures of gas during the cycle are T1=400K and T2=800K, respectively, and the ratio of maximum to minimum volume is 4.
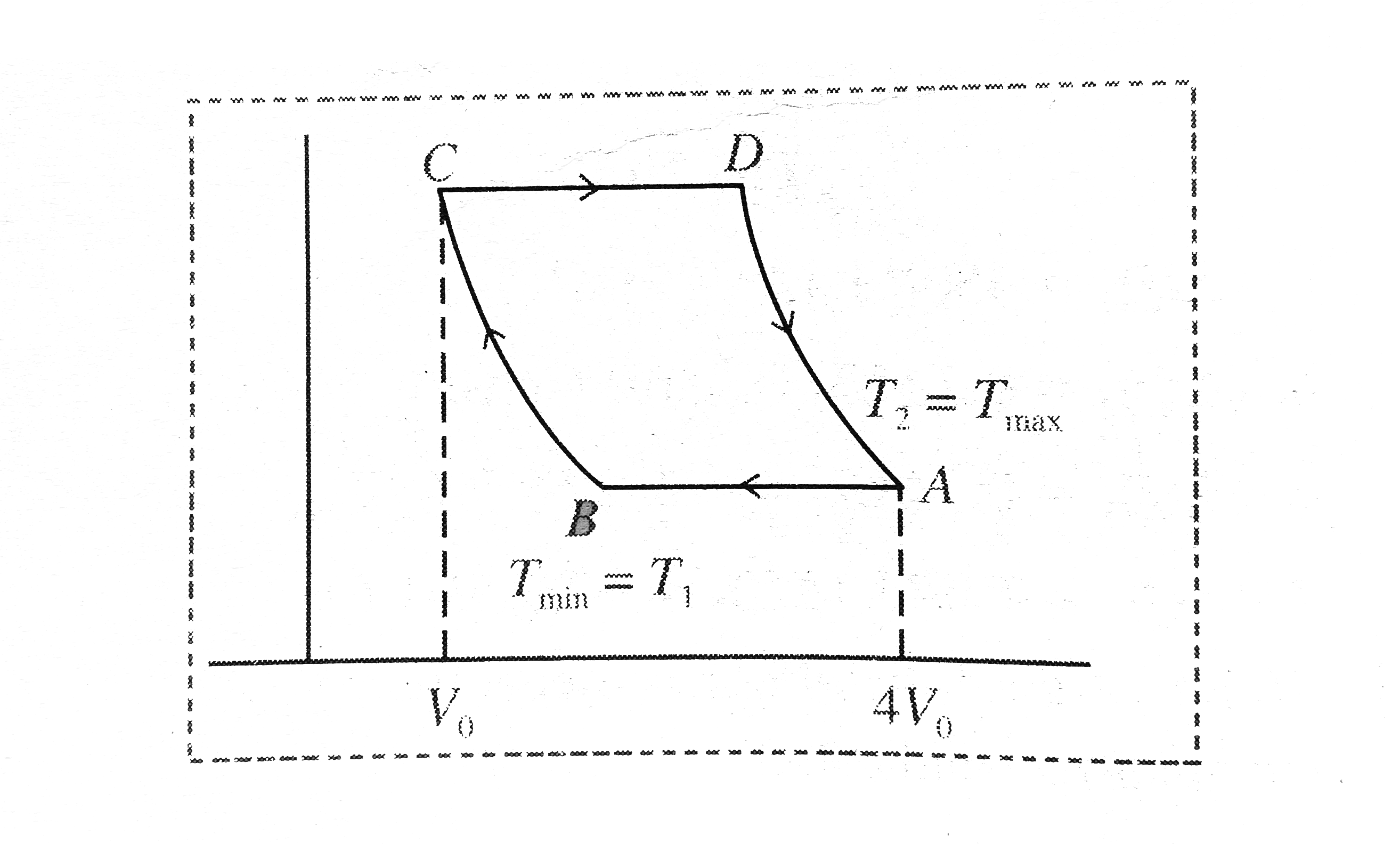
The heat is extracted from the system in process
View SolutionA monatomic gas undergoes a cycle consisting of two isothermals and two isobarics. The minimum and maximum temperatures of gas during the cycle are T1=400K and T2=800K, respectively, and the ratio of maximum to minimum volume is 4. The volume at B is
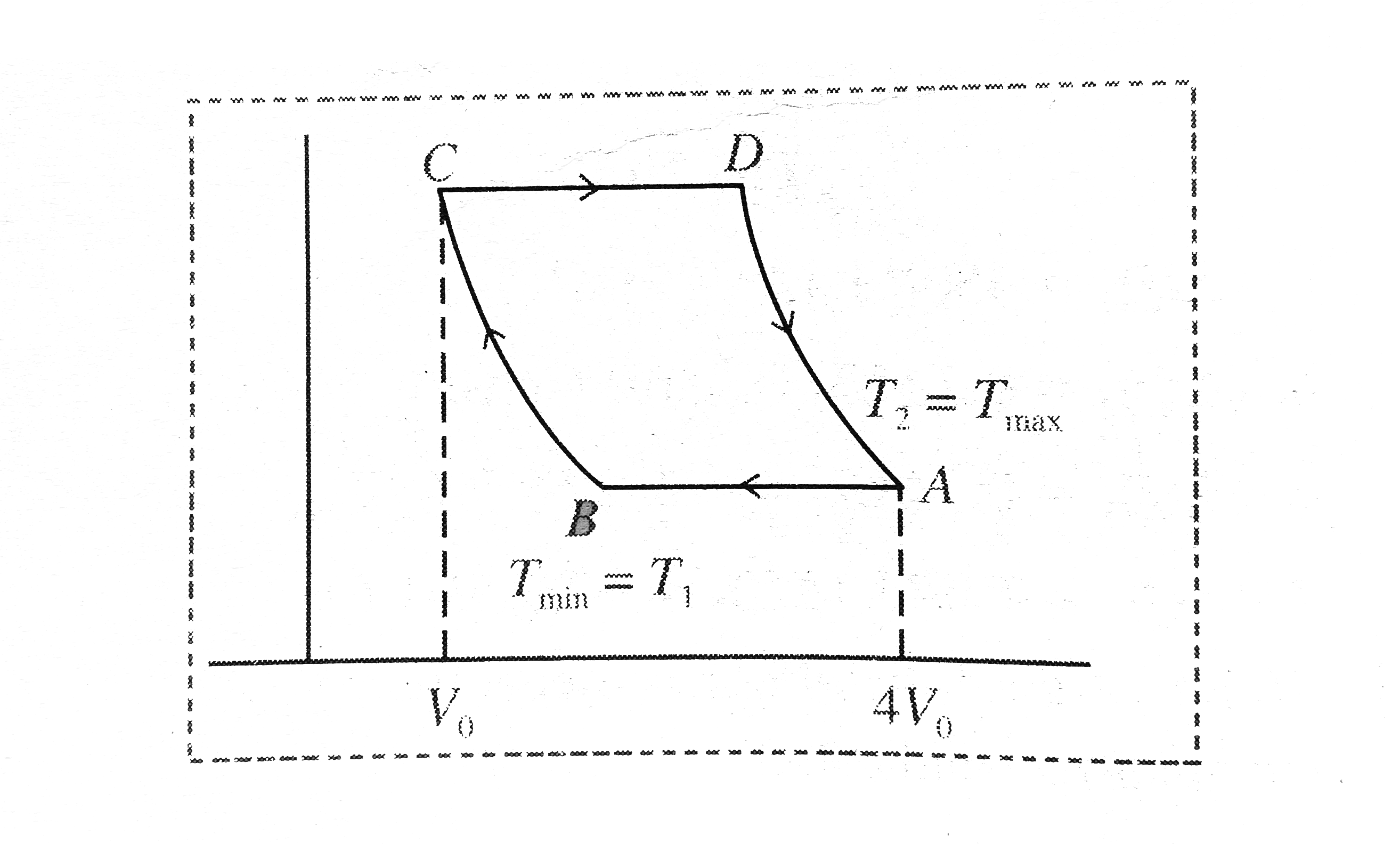
View SolutionA monatomic gas undergoes a cycle consisting of two isothermals and two isobarics. The minimum and maximum temperatures of gas during the cycle are T1=400K and T2=800K, respectively, and the ratio of maximum to minimum volume is 4.
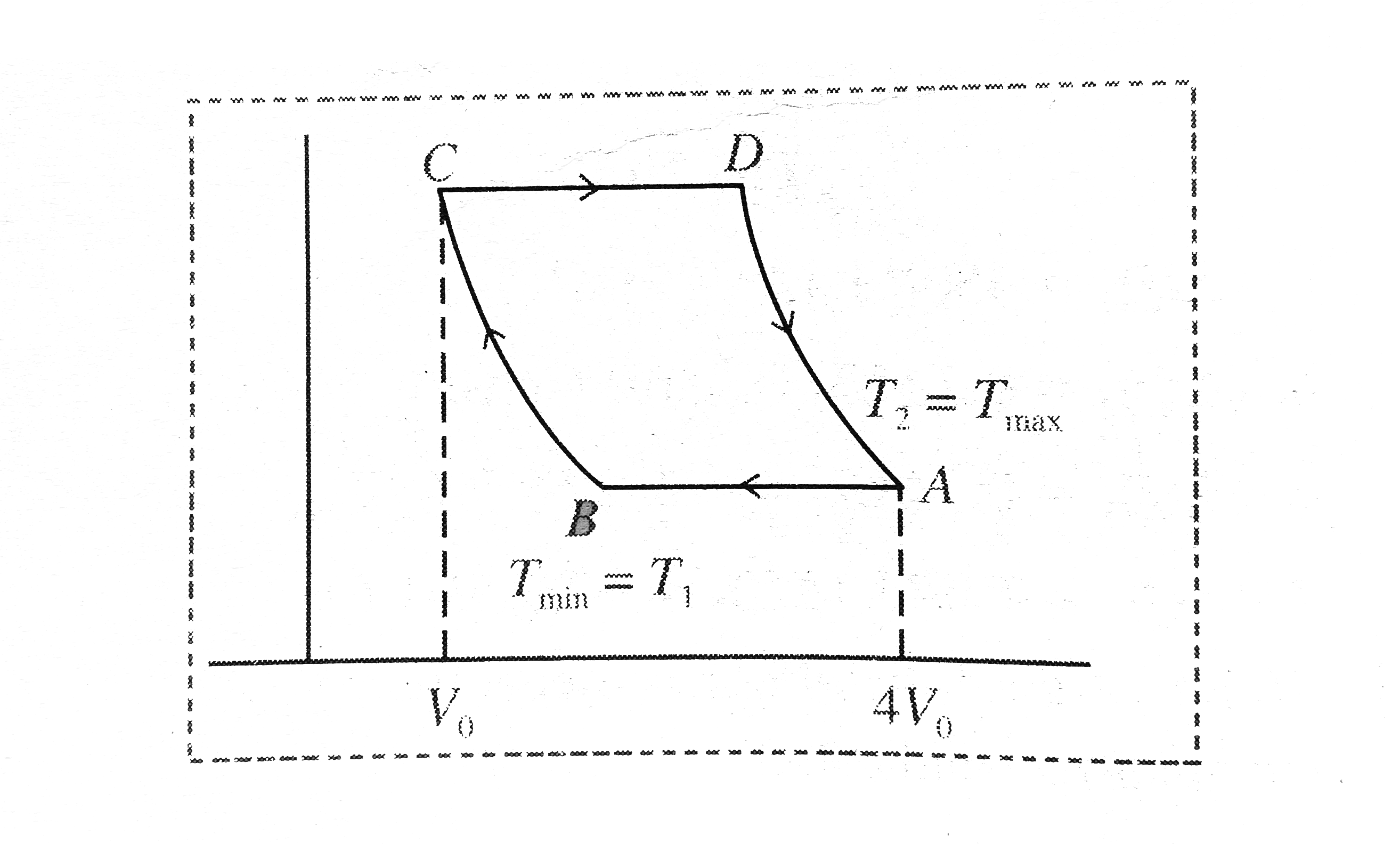
The volume is D is
View SolutionA sample of 1.0 mol of a monoatomic ideal gas is taken through a cyclic process of expansion and compression as shown in figure. What will be the value of ΔH for the cycle as a whole ?
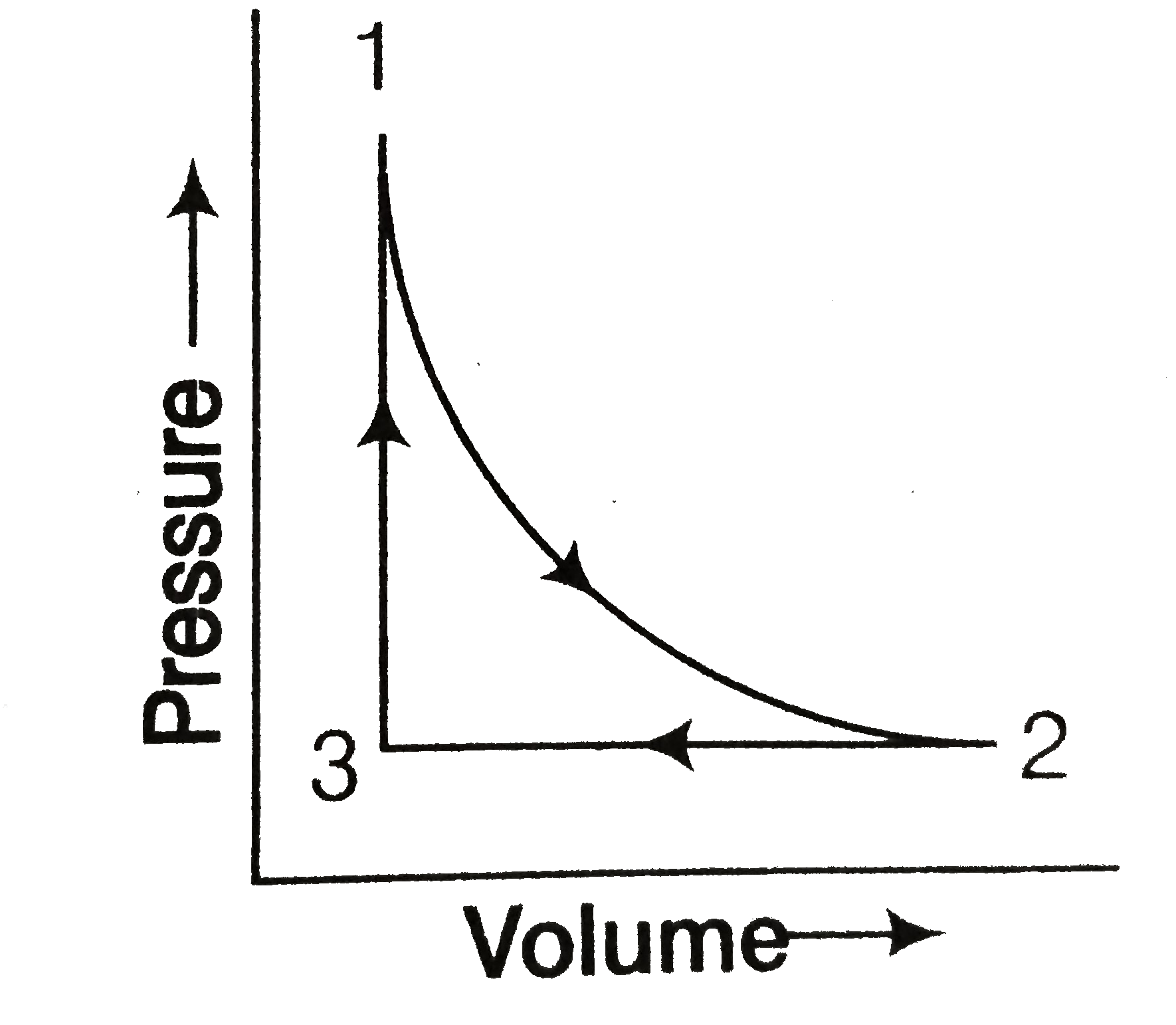
View Solution
