The frictional force of the air on the body of mass 0.25kg, falling with an acceleration of 9.2 m/s2 will be (g=9.8m/s2).
1.0N
0.55N
0.25N
0.15N
The correct Answer is:D
To solve the problem of finding the frictional force of air on a body of mass 0.25 kg falling with an acceleration of 9.2 m/s², we can follow these steps:
Step 1: Identify the forces acting on the body
When the body is falling, it experiences two main forces:
- The gravitational force (weight) acting downwards, which is given by Fg=mg.
- The frictional force (air resistance) acting upwards, which we will denote as Ff.
Step 2: Calculate the weight of the body
The weight of the body can be calculated using the formula:
Fg=mg
Where:
- m=0.25kg (mass of the body)
- g=9.8m/s2 (acceleration due to gravity)
Substituting the values:
Fg=0.25kg×9.8m/s2=2.45N
Step 3: Apply Newton's second law of motion
According to Newton's second law, the net force acting on the body is equal to the mass of the body multiplied by its acceleration:
Fnet=ma
Where:
- a=9.2m/s2 (downward acceleration)
Substituting the values:
Fnet=0.25kg×9.2m/s2=2.3N
Step 4: Relate the forces
The net force can also be expressed as the difference between the gravitational force and the frictional force:
Fnet=Fg−Ff
Substituting the known values:
2.3N=2.45N−Ff
Step 5: Solve for the frictional force
Rearranging the equation to solve for Ff:
Ff=2.45N−2.3N=0.15N
Conclusion
The frictional force of the air on the body is 0.15N.
---
When the body is falling, it experiences two main forces:
- The gravitational force (weight) acting downwards, which is given by Fg=mg.
- The frictional force (air resistance) acting upwards, which we will denote as Ff.
The weight of the body can be calculated using the formula:
Fg=mg
Where:
- m=0.25kg (mass of the body)
- g=9.8m/s2 (acceleration due to gravity)
Fg=0.25kg×9.8m/s2=2.45N
According to Newton's second law, the net force acting on the body is equal to the mass of the body multiplied by its acceleration:
Fnet=ma
Where:
- a=9.2m/s2 (downward acceleration)
Fnet=0.25kg×9.2m/s2=2.3N
The net force can also be expressed as the difference between the gravitational force and the frictional force:
Fnet=Fg−Ff
Substituting the known values:
2.3N=2.45N−Ff
Rearranging the equation to solve for Ff:
Ff=2.45N−2.3N=0.15N
The frictional force of the air on the body is 0.15N.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-III
Explore 28 Videos
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-I
Explore 52 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBook:ALLENChapter:NEWTON'S LAWS OF MOTION & FRICTIONExercise:EXERCISE (JA)
Explore 4 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
Explore 24 Videos
Similar Questions
Find the friction force due to air on a body of mass 1 kg falling with acceleration 8m/s2 :-
View SolutionHow much force is required to cause an object of mass 2 kg to have an acceleration of 4m/s2 ?
View SolutionCalculate the magnitude of force which when applied on a body of mass 0.5 kg produces an acceleration of 5 ms−2
View SolutionCalculate the magnitude of force which when applied on a body of mass 0.5 kg produces an acceleration of 5m s−2.
View SolutionIf coeffiecient of friction between the block of mass 2kg and table is 0.4 then out acceleration of the system and tension in the string. (g=10m/s2)
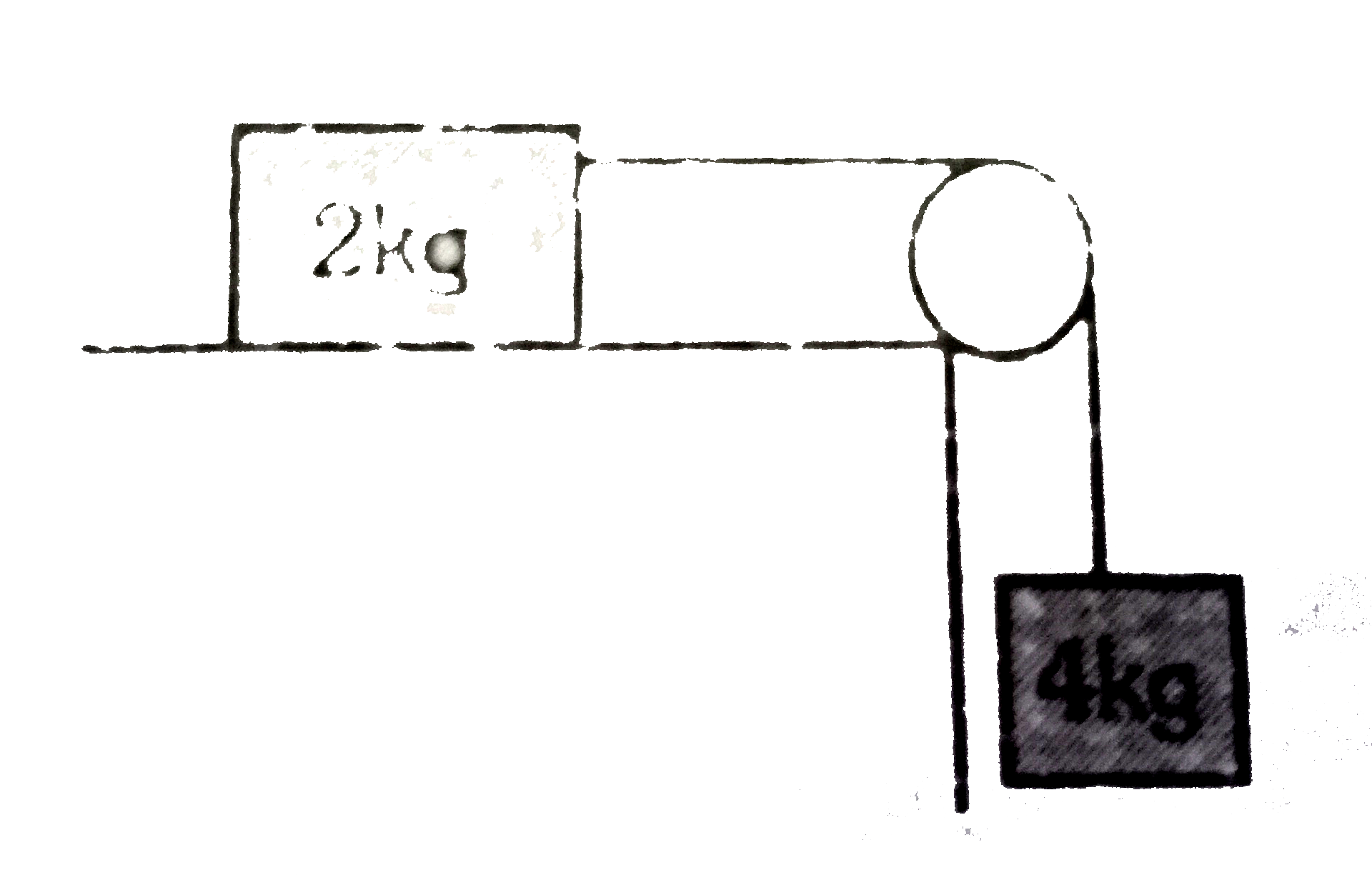
View SolutionBlock of mass 10 kg is moving on inclined plane with constant velocity 10 m/s. The coedfficient of kinetic friction between incline plane and block is :-
View SolutionA body of mass 2 kg slides down with an acceleration of 3 m/s2 on a rough inclined plane having a slope of 30∘ The external force required to take the same body up the plane with the same acceleration will be : (g=10m/s2)
View SolutionA simple pendulum of length 1 m with a bob of mass m swings with an angular amplitude 30∘. Then (g=9.8m/s2
View SolutionThe coefficient of friction between a body of mass 1kg and the surface of an inclined plane at 45∘ is 0.5. if g=9.8m/s2, the acceleration of the body downwards in m/s2 is
View SolutionCalculate the weight of a body of mass 10 kg in newton . Take g=9.8m s−2 .
View Solution