ΔU∘ of combustoin of methane is −XKJmol−1 The value
of ΔH∘ is :-
−X−596R
−X+596R
X+596R
X−596R
The correct Answer is:A
To find the standard enthalpy change (ΔH°) for the combustion of methane given the standard internal energy change (ΔU°) is -X kJ/mol, we can follow these steps:
Step 1: Understand the relationship between ΔH° and ΔU°
The relationship between the standard enthalpy change (ΔH°) and the standard internal energy change (ΔU°) is given by the equation:
ΔH∘=ΔU∘+ΔNgRT
where:
- ΔN_g = change in the number of moles of gas
- R = universal gas constant (8.314 J/(mol·K))
- T = temperature in Kelvin (298 K for standard conditions)
Step 2: Write the combustion reaction of methane
The balanced chemical equation for the combustion of methane (CH₄) is:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
Step 3: Calculate ΔN_g
ΔN_g is calculated as:
ΔNg=moles of gaseous products−moles of gaseous reactants
From the reaction:
- Moles of gaseous products = 1 (from CO₂)
- Moles of gaseous reactants = 1 (from CH₄) + 2 (from O₂) = 3
Thus,
ΔNg=1−3=−2
Step 4: Substitute values into the equation
Now we can substitute ΔU°, ΔN_g, R, and T into the equation:
ΔH∘=ΔU∘+ΔNgRT
Substituting the known values:
ΔH∘=−X+(−2)(8.314J/(mol⋅K))(298K)
Step 5: Convert R to kJ
Since ΔU° is given in kJ, we need to convert R from J to kJ:
R=8.314J/(mol⋅K)=0.008314kJ/(mol⋅K)
Step 6: Calculate ΔH°
Now substituting R in kJ:
ΔH∘=−X−2(0.008314)(298)
Calculating the second term:
ΔH∘=−X−4.964kJ
Final Answer
Thus, the final expression for ΔH° is:
ΔH∘=−X−4.964kJ/mol
The relationship between the standard enthalpy change (ΔH°) and the standard internal energy change (ΔU°) is given by the equation:
ΔH∘=ΔU∘+ΔNgRT
where:
- ΔN_g = change in the number of moles of gas
- R = universal gas constant (8.314 J/(mol·K))
- T = temperature in Kelvin (298 K for standard conditions)
The balanced chemical equation for the combustion of methane (CH₄) is:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
ΔN_g is calculated as:
ΔNg=moles of gaseous products−moles of gaseous reactants
From the reaction:
- Moles of gaseous products = 1 (from CO₂)
- Moles of gaseous reactants = 1 (from CH₄) + 2 (from O₂) = 3
ΔNg=1−3=−2
Now we can substitute ΔU°, ΔN_g, R, and T into the equation:
ΔH∘=ΔU∘+ΔNgRT
Substituting the known values:
ΔH∘=−X+(−2)(8.314J/(mol⋅K))(298K)
Since ΔU° is given in kJ, we need to convert R from J to kJ:
R=8.314J/(mol⋅K)=0.008314kJ/(mol⋅K)
Now substituting R in kJ:
ΔH∘=−X−2(0.008314)(298)
Calculating the second term:
ΔH∘=−X−4.964kJ
Thus, the final expression for ΔH° is:
ΔH∘=−X−4.964kJ/mol
Topper's Solved these Questions
- CHEMISTRY AT A GLANCEBOOK - ALLENCHAPTER - CHEMISTRY AT A GLANCEEXERCISE - INORGANIC CHEMISTRY
300 Videos
- CHEMISTRY AT A GLANCEBOOK - ALLENCHAPTER - CHEMISTRY AT A GLANCEEXERCISE - ORGANIC CHEMISTRY
472 Videos
- Chemical EquilibriumBOOK - ALLENCHAPTER - Chemical EquilibriumEXERCISE - All Questions
30 Videos
- ELECTROCHEMISTRYBOOK - ALLENCHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE -05 [B]
38 Videos
Similar Questions
Change in enthalpy and change in internal energy are state functions. The value of ΔH,ΔU can be determined by using Kirchoff's equation.
Calculate the heat of formation of methane, given that heat of formation of water =−286kJmol−1, heat of combustion of methane =−890kJmol−1 heat of combustion of carbon =−393.5kJmol−1
View SolutionThe value of ΔH∘ for the reaction Cu+(g)+I−(g)→CuI(g) is -446kJ mol−1. If the ionisation energy of Cu(g) is 745 kJ mol−1 and the electron affinity of I(g) is -295 kJ mol−1, then the value of ΔH∘ for the formation of one mole of CuI(g) from Cu(g) and I(g) is:
View Solution
Knowledge Check
ΔU∘ of combustion of methane is −XkJmol−1. The value of ΔH∘ is
A=ΔU∘B>ΔU∘C<ΔU∘D=0ΔU∘ of combustion of CH4(g) at certain temperature is −393 kJ mol−1. The value of ΔH∘ is
AzeroB<ΔH∘C>ΔU∘Dequal to ΔU∘
The ΔH0f(KF,s) is −563kJmol−1. The ionization enthalpy of K(g) is 419kJmol−1. and the enthalpy of sublimation of potassium is 88kJmol−1. The electron affinity of F(g) is 322kJmol−1 and F−F bond enthalpy is 158kJmol_1. Calculate the lattice enthalpy of KF(s).
The given data are as follows:
(i) K(s)+1/2F2(g)→KF(s) ΔH0f=−563kJmol−1
(ii) K(g)→K+(g)+e− Δ0Ioniz=419kJmol−1
(iii) K(s)→K(g) ΔH0sub=88kJmol−1
(iv) F(g)+e−→F−(g) ΔH0eg=−322kJmol−1
(v) F2(g)→2F(s) ΔH0diss=158kJmol−1
(vi) K+(g)+F−(g)→KF(s) ΔH0L=?
View SolutionThe first (ΔH1) and second (ΔH2) ionisation enthalpies (in kJmol−1) and the (ΔHeg) electron gain enthalpy (in kJmol−1) of a elements are given below:
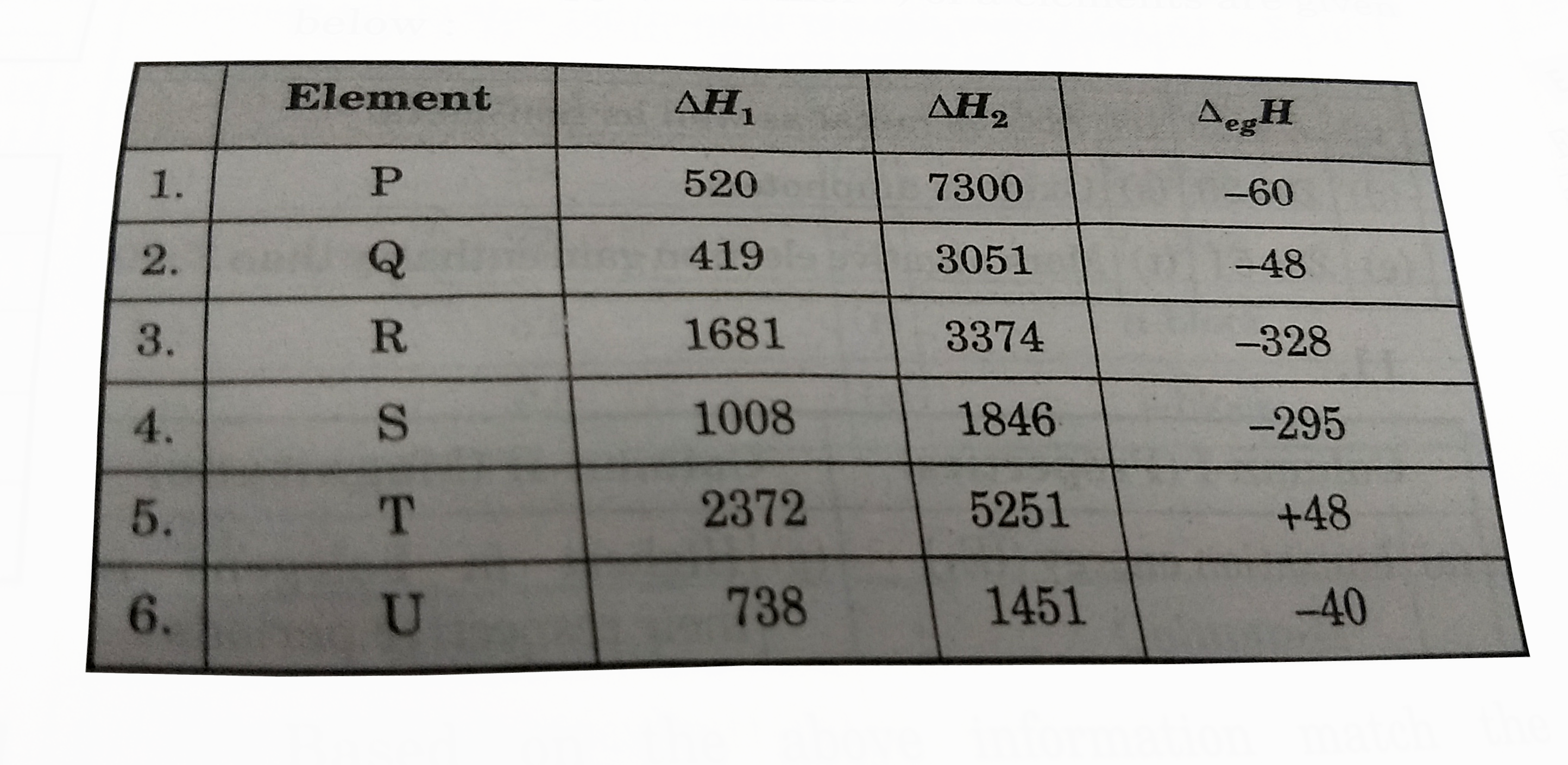
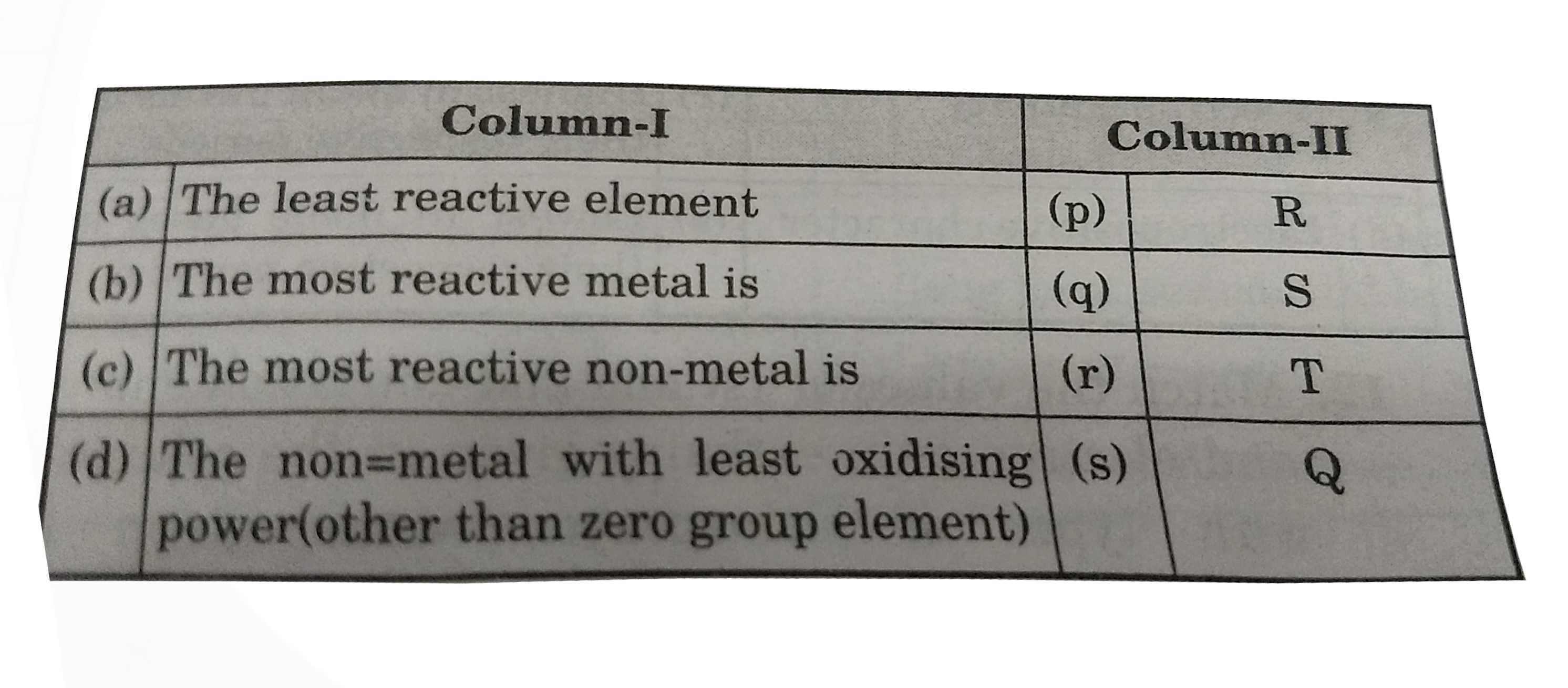
Based on the above information match the following columns.
View SolutionFor a gaseous reaction, A(g)+3B(g)→3C(g)+3D(g),ΔU is 17 kcal at 27∘C. Assuming R=2cal K−1mol−1, the value of ΔH for the above reaction is:
View SolutionGiven C2H2(g)+H2(g)→C2H4(g):ΔH∘=−175 kJ mol−1
ΔH∘f(C2H4,g)=50 kJ mol−1,ΔH∘f(H2O,l)=−280 kJ mol−1,ΔH∘f(CO2g)=−390 kJ mol−1
If ΔH∘ is enthalpy of combustion (in kJ mol−1) of C2H2(g), then calculate the value of ∣∣∣ΔH∘257∣∣∣
View SolutionCalculate the heat of formation of methane in kcalmol−1 using the following thermo chemical reactions
C(s)+O2→CO2(g),ΔH=−94.2kcalmol−1
H2(g)+12O2(g)→H2O(l),ΔH=−68.3kcalmol−1
CH4(g)+2O2(g)→CO2(g)+2H2O(l),ΔH=−210.8kcalmol−1
View SolutionStandard enthalpy of vapourisation ΔH∘ forwater is 40.66KJmol−1.The internal energy of vapourisation of water for its 2 mol will be :-
View Solution
