Statement-I : When an algebraic equation has been derved, it is advisable to check it for dimensional consistency.
Statement-II : This guarantee that the equation is correct.
Statement-I is true, Statement-II is true, Statement-II is correct explanation for statement-I
Statement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for statement-I
Statement-I is true, Statement-II is false.
Statement-I is false and Statement-II is true.
The correct Answer is:A
To analyze the statements provided in the question, we will break down the reasoning behind each statement step by step.
Step 1: Understanding Statement I
Statement I: "When an algebraic equation has been derived, it is advisable to check it for dimensional consistency."
- Explanation: Dimensional consistency means that both sides of an equation must have the same dimensions. This is crucial because if the dimensions do not match, it indicates that there is an error in the derivation of the equation. For example, if we derive an equation for force, we should ensure that the dimensions of mass and acceleration combine to give the dimensions of force.
Step 2: Understanding Statement II
Statement II: "This guarantees that the equation is correct."
- Explanation: While checking for dimensional consistency is a good practice and can help identify errors, it does not guarantee that the equation is correct in all cases. Dimensional analysis can show that the equation is dimensionally consistent, but it does not account for all possible errors, such as numerical coefficients or the underlying physics of the situation. Therefore, while Statement II is related to Statement I, it is not entirely accurate to claim that dimensional consistency guarantees correctness.
Conclusion
- Final Evaluation:
- Statement I is true because checking for dimensional consistency is a necessary step in verifying the correctness of a derived equation.
- Statement II is false because dimensional consistency does not guarantee that the equation is correct; it only suggests that the dimensions match.
Final Answer
- Statement I is true, and Statement II is false.
---
Statement I: "When an algebraic equation has been derived, it is advisable to check it for dimensional consistency."
Statement II: "This guarantees that the equation is correct."
- Final Evaluation:
- Statement I is true because checking for dimensional consistency is a necessary step in verifying the correctness of a derived equation.
- Statement II is false because dimensional consistency does not guarantee that the equation is correct; it only suggests that the dimensions match.
- Statement I is true, and Statement II is false.
Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Comprehension 1
5 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Comprehension 2
8 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Exersice-03
7 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLENCHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
8 Videos
Similar Questions
Knowledge Check
Assertion: A dimensionally wrong or inconsistaent equation must be wrong.
Reason: A dimensionally consistent equation is a exact or a correct equation.Aif both assertion and reason are true reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation fo assertion.CIf assertion is true but reaso is false.DIF both assertion and reason are false.
Tangents are drawn from the point (17, 7) to the circle x2+y2=169, Statement I The tangents are mutually perpendicular Statement, lls The locus of the points frorn which mutually perpendicular tangents can be drawn to the given circle is x2+y2=338 (a) Statement I is correct, Statement II is correct; Statement II is a correct explanation for Statementl (b( Statement I is correct, Statement I| is correct Statement II is not a correct explanation for Statementl (c)Statement I is correct, Statement II is incorrect (d) Statement I is incorrect, Statement II is correct
View SolutionStatement 1 : Order of a differential equation represents the number of arbitrary constants in the general solution. Statement 2 : Degree of a differential equation represents the number of family of curves. Which of the following Statement is/are are correct ?
View SolutionStatement I The equation |(x−2)+a|=4 can have four distinct real solutions for x if a belongs to the interval (−∞,4).
Statemment II The number of point of intersection of the curve represent the solution of the equation.
(a)Both Statement I and Statement II are correct and Statement II is the correct explanation of Statement I
(b)Both Statement I and Statement II are correct but Statement II is not the correct explanation of Statement I
(c)Statement I is correct but Statement II is incorrect
(d)Statement II is correct but Statement I is incorrect
View SolutionStatement I Order of differential equation of family of parabola whose axis is parallel to y-axis and latusrectum is fixed is 2. Statement II Order of first equation is same as actual number of arbitary constant present in differential equation.
View Solution(a) Statement I is true, Statement II is true , Statement II is a correct explanation for statement I.
(b) Statement I is true, Statement II is true, Statement II is not a correct explanation for Statement I.
(C) Statement I is true, Statement II is false.
(D) Statement I is false , Statement II is true.
Statement I through the point (π,π+1),π<2, there cannot be more than one normal to the parabola y2=4ax . Statement II The point (π,π+1) cannot lie inside the parabola y2=4ax .
View Solution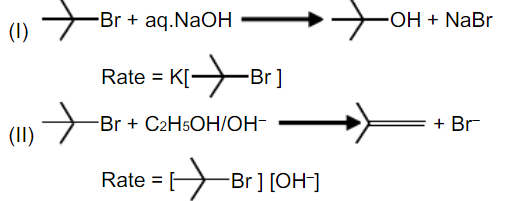
Correct statement regarding these two reaction I and II
View SolutionStatement-I : Each electron in an atom has two spin quantum numbers.
Because
Statement-II : Spin quantum numbers are obtained by solving Schrodinger wave equation.
View Solution
