Two trains A and B are moving in the same direction with velocities 30 m/s and 10 m/s respectively, B is behind from A, blows a horn of frequency 450 Hz. Then the apparent frequency heard by B is (The velocity of sound is 330 m/s) :-
425 Hz
300 Hz
450 Hz
350 Hz
The correct Answer is:A
To solve the problem of finding the apparent frequency heard by train B when it blows a horn while moving, we can use the Doppler effect formula. Here’s a step-by-step solution:
Step 1: Identify the given values
- Frequency of the horn (f) = 450 Hz
- Velocity of sound (v) = 330 m/s
- Velocity of train A (v_A) = 30 m/s
- Velocity of train B (v_B) = 10 m/s
Step 2: Understand the scenario
Train A is moving faster than train B, and both are moving in the same direction. Train B is behind train A and is the observer in this scenario.
Step 3: Use the Doppler effect formula
The apparent frequency (f') heard by an observer moving in the same direction as the source is given by the formula:
f′=f×v+vBv+vA
Where:
- f′ = apparent frequency
- f = actual frequency of the source
- v = speed of sound
- vB = speed of the observer (train B)
- vA = speed of the source (train A)
Step 4: Substitute the values into the formula
Substituting the known values into the formula:
f′=450×330+10330+30
Step 5: Calculate the values
1. Calculate the numerator:
330+10=340
2. Calculate the denominator:
330+30=360
3. Substitute these values back into the equation:
f′=450×340360
Step 6: Simplify the fraction
340360=3436=1718
Thus,
f′=450×1718
Step 7: Perform the multiplication
f′=450×0.9444≈425 Hz
Step 8: Conclusion
The apparent frequency heard by train B is approximately 425 Hz.
Final Answer
The apparent frequency heard by train B is 425 Hz.
---
- Frequency of the horn (f) = 450 Hz
- Velocity of sound (v) = 330 m/s
- Velocity of train A (v_A) = 30 m/s
- Velocity of train B (v_B) = 10 m/s
Train A is moving faster than train B, and both are moving in the same direction. Train B is behind train A and is the observer in this scenario.
The apparent frequency (f') heard by an observer moving in the same direction as the source is given by the formula:
f′=f×v+vBv+vA
Where:
- f′ = apparent frequency
- f = actual frequency of the source
- v = speed of sound
- vB = speed of the observer (train B)
- vA = speed of the source (train A)
Substituting the known values into the formula:
f′=450×330+10330+30
1. Calculate the numerator:
330+10=340
2. Calculate the denominator:
330+30=360
3. Substitute these values back into the equation:
f′=450×340360
340360=3436=1718
Thus,
f′=450×1718
f′=450×0.9444≈425 Hz
The apparent frequency heard by train B is approximately 425 Hz.
The apparent frequency heard by train B is 425 Hz.
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBook:ALLENChapter:WAVES AND OSCILLATIONSExercise:Part-1(Exercise-02)
Explore 19 Videos
- WAVES AND OSCILLATIONSBook:ALLENChapter:WAVES AND OSCILLATIONSExercise:Part-1(Exercise-03)
Explore 31 Videos
- WAVES AND OSCILLATIONSBook:ALLENChapter:WAVES AND OSCILLATIONSExercise:Part-3(Example)
Explore 32 Videos
- SEMICONDUCTORSBook:ALLENChapter:SEMICONDUCTORSExercise:Part-3(Exercise-4)
Explore 51 Videos
Similar Questions
Two cars moving in opposite direction approach each other with speed of 22m/s and 16.5m/s respectively . The driver of the first car blows a horn having a frequency 400Hz. The frequency heard by the driver of the second car is [ velocity of the sound 340m/s]
View SolutionTwo car moving in opposite directions approach each other with speed of 22m/s and 16.5m/s respectively. The driver of the first car blows a horn having a frequency 400Hz. The frequency heard by the driver of the second car is [velocity of sound 340m/s].
View SolutionOn a platform a man is watching two trains one leaving the station the other approaching the station, both with the same velocity 2 m/s and blowing their horn with a frequency 480 Hz. The number of beats heard by the man will be (Velocity of sound in air is 320 m/s)
View SolutionA train is moving with speed 72 km/h towards a hill blows a whistle of frequency 1240Hz. the frequency of echo heard by driver is(speed of sound=330m/s)
View Solutiona bus is moving in a circle around a listener with speed 20 m/s of radius 2 m. Bus driver blows a horn with frequency 210 Hz. the frequency of sound heard by the listener is
View SolutionDistance travelled by the sound produced by a tuning fork of frequency 50 Hz completing 200 vibrations is, (the velocity of sound in air is 330 m/s)
View SolutionTwo trains A and B approach a station from opposite sides, sounding their whistles. A stationary observer on the platform hears no beats. If the velocities of A and B are 15 m/s and 30 m/s respectively and the real frequency of the whistle of B is 600 Hz, find the real frequency of the whistle of A. (Velocity of sound = 330 m/s)
View SolutionAn observer is moving towards a source with speed 15 m/s. The source is moving with speed 5 m/s in the same direction. Air is blowing with speed 10 m/s from observer to source. If frequency of sound emitted is 325 Hz then find frequency of sound heard by observer (velocity of sound in air = 330 m/s) in Hz .
View Solution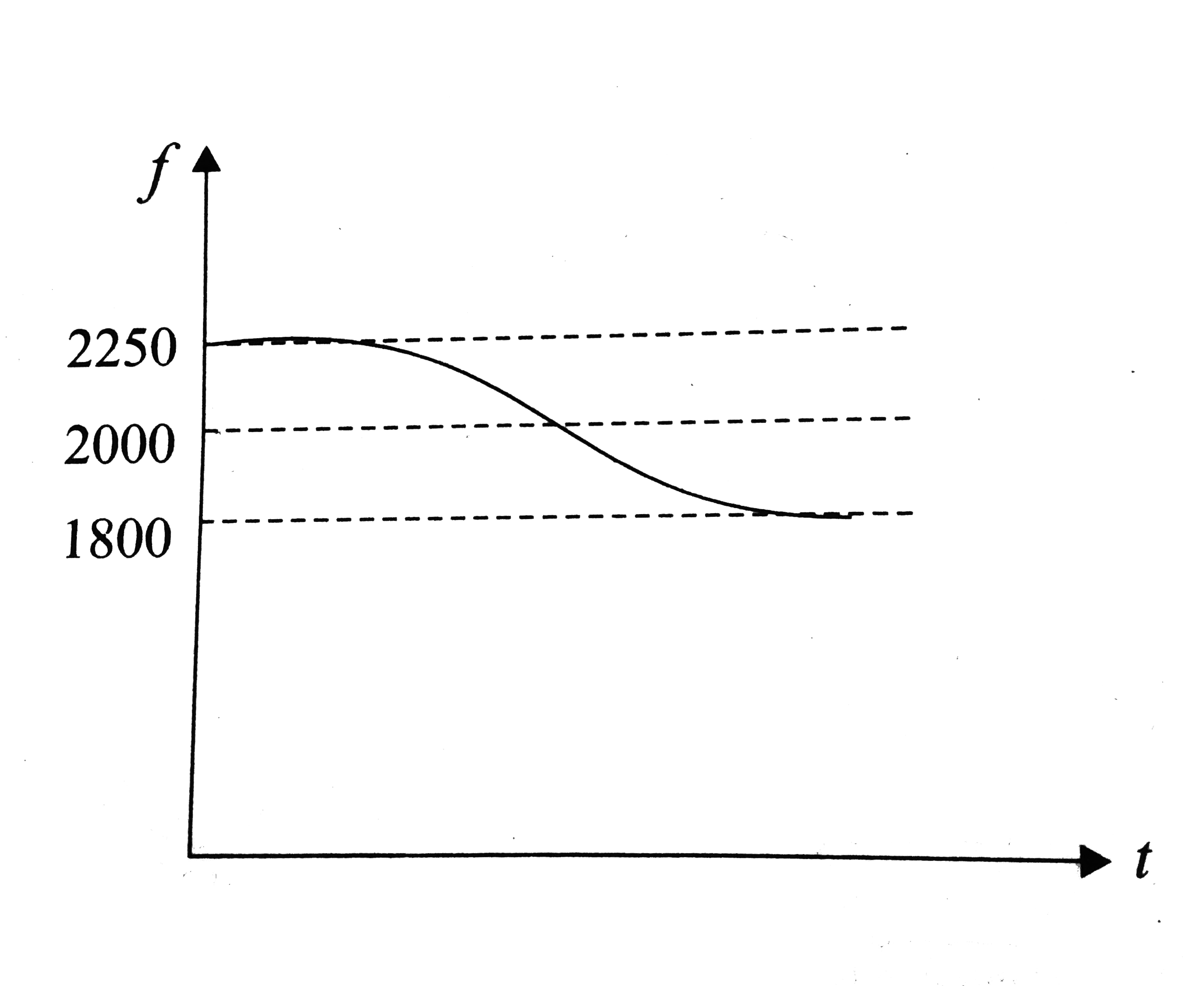
A statinary observer receives a sound of frequency 2000 Hz. The variation of apparent frequency and time is shown. Find the speed of source, if velocity of sound is 300ms
View SolutionTwo cars A and B are moving in same direction with velocities 30 m/s and 20 m/s. When car A is at a distance d behind the car B, the driver of the car A applies brakes producing uniform retardation of 2m/s2. There will be no collision when :-
View Solution