The e.m.f. of cell: H2(g) |Buffer| Normal calomal electrode is 0.6885V at 40∘C when the barometric pressure is 725mm of Hg. What is the pH of the solution E∘calomal=0.28.
The correct Answer is:6.6
To solve the problem step by step, we will use the Nernst equation and the information provided in the question.
Step 1: Write the Nernst Equation
The Nernst equation for the cell can be written as:
Ecell=E∘cell−RTnFlnQ
Where:
- Ecell = EMF of the cell
- E∘cell = Standard electrode potential
- R = Universal gas constant (8.314 J/(mol·K))
- T = Temperature in Kelvin
- n = Number of electrons transferred in the reaction
- F = Faraday's constant (96500 C/mol)
- Q = Reaction quotient
Step 2: Convert Temperature to Kelvin
The temperature given is 40°C. To convert this to Kelvin:
T=40+273.15=313.15K≈313K
Step 3: Substitute Known Values into the Nernst Equation
Given:
- Ecell=0.6885V
- E∘cell=0.28V
- n=2 (since 2 electrons are involved in the reaction)
- R=8.314J/(mol⋅K)
- F=96500C/mol
Substituting these values into the Nernst equation:
0.6885=0.28−(8.314)(313)(2)(96500)lnQ
Step 4: Calculate the Value of RTnF
Calculating RTnF:
(8.314)(313)(2)(96500)=2600.862193000≈0.0135
Step 5: Rearrange the Nernst Equation to Solve for Q
Rearranging the equation gives:
0.6885=0.28−0.0135lnQ
0.0135lnQ=0.28−0.6885
0.0135lnQ=−0.4085
lnQ=−0.40850.0135≈−30.24
Step 6: Calculate Q
Taking the exponent to find Q:
Q=e−30.24≈8.5×10−14
Step 7: Relate Q to H+ Concentration
For the reaction H2(g)⇌2H+(aq)+2e−, we can express Q as:
Q=(H+)2PH2
Given that the barometric pressure is 725 mm Hg, we convert this to atm:
PH2=725760≈0.9539atm
Thus,
Q=(H+)20.9539
Step 8: Solve for (H+)
Substituting Q into the equation:
8.5×10−14=(H+)20.9539
(H+)2=8.5×10−14×0.9539
(H+)2≈8.1×10−14
(H+)≈√8.1×10−14≈2.85×10−7M
Step 9: Calculate pH
Using the formula for pH:
pH=−log(H+)
Calculating pH:
pH=−log(2.85×10−7)≈6.6
Final Answer
The pH of the solution is approximately 6.6.
---
The Nernst equation for the cell can be written as:
- Ecell = EMF of the cell
- E∘cell = Standard electrode potential
- R = Universal gas constant (8.314 J/(mol·K))
- T = Temperature in Kelvin
- n = Number of electrons transferred in the reaction
- F = Faraday's constant (96500 C/mol)
- Q = Reaction quotient
The temperature given is 40°C. To convert this to Kelvin:
Given:
- Ecell=0.6885V
- E∘cell=0.28V
- n=2 (since 2 electrons are involved in the reaction)
- R=8.314J/(mol⋅K)
- F=96500C/mol
Calculating RTnF:
Rearranging the equation gives:
Taking the exponent to find Q:
For the reaction H2(g)⇌2H+(aq)+2e−, we can express Q as:
Substituting Q into the equation:
Using the formula for pH:
The pH of the solution is approximately 6.6.
Topper's Solved these Questions
- ELECTROCHEMISTRYBOOK - ALLENCHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE-05 [A]
28 Videos
- ELECTROCHEMISTRYBOOK - ALLENCHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE -05 [B]
38 Videos
- ELECTROCHEMISTRYBOOK - ALLENCHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE-04 [A]
55 Videos
- CHEMISTRY AT A GLANCEBOOK - ALLENCHAPTER - CHEMISTRY AT A GLANCEEXERCISE - ORGANIC CHEMISTRY
472 Videos
- HYDROCARBONBOOK - ALLENCHAPTER - HYDROCARBONEXERCISE - MCQ
15 Videos
Similar Questions
The cell Pt|H2(g)(1atm)|H+,pH=x∣∣∣ Normal calomal electrode has EMF of 0.64 volt at 25∘C. The standard reduction potential of normal calomal electrode is 0.28V. What is the pH of solution in anodic compartment. Take 2.303RTF=0.06 at 298K.
View SolutionThe cell Pt,H2(1atm)H+(pH=x)∣∣ Normal calomel Electrode has an EMF of 0.67V at 25∘C .Calculate the pH of the solution. The oxidation potential of the calomel electrode on hydrogen scale is −0.28V.
View SolutionThe cell Pt∣H2(g,01 bar) ∣∣H+(aq),pH=x∣∣∣∣Cl−(1M)∣∣Hg2Cl2|Hg|Pt has emf of 0.5755 V at 25∘C the SOP of calomel electrode is −0.28V then pH of the solution will be
(a)11
(b)4.5
(c)5
(d)None of these
View SolutionThe e.m.f of the following cell
Pt|H2(1 atm.)/H+(aq||Ag+(1M)1Ag(s) at 25∘C is 0.87V. Calculate the pH of the acid solution.
EΘAg+/Ag=0.80V,E2H+/H2=0.0V
View SolutionThe quinhydrone electrode (Q,H⊕∣H2Q) is used in conjunction with a saturated calomel electrode, as represented below:
EMFcell=0.264V at 30∘C. Calculate the pH of unknown solution at this temperature.
Given :Ecalomel=0.24V and Ec−.2H⊕,Q∣H2Q=0.7V
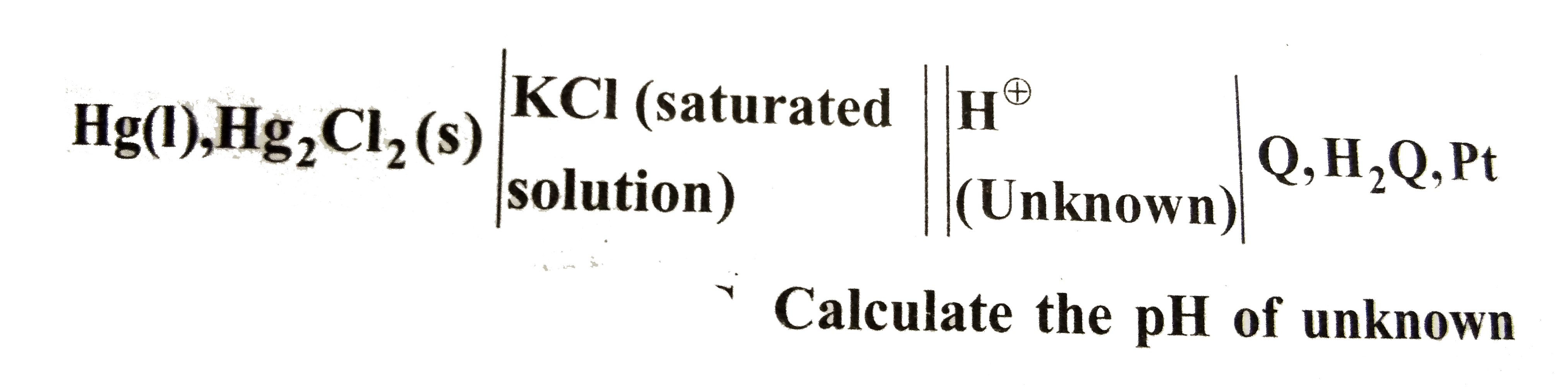
View SolutionIn the acid base titration [H3PO4(0.1M)+NaOH(0.1M)] e.m.f of the solution is measured by coupling this electrodes with suitable reference electrode.When alkali is added pH of solution is in acoodance with equation
ECell=E∘Cell+0.059pH
For H3PO4Ka1=10−3,Ka2=10−8,Ka3=10−13
What is the cell e.m.f at the lind end point of the titration if E∘cell at this stage is 1.3805 V.
View Solution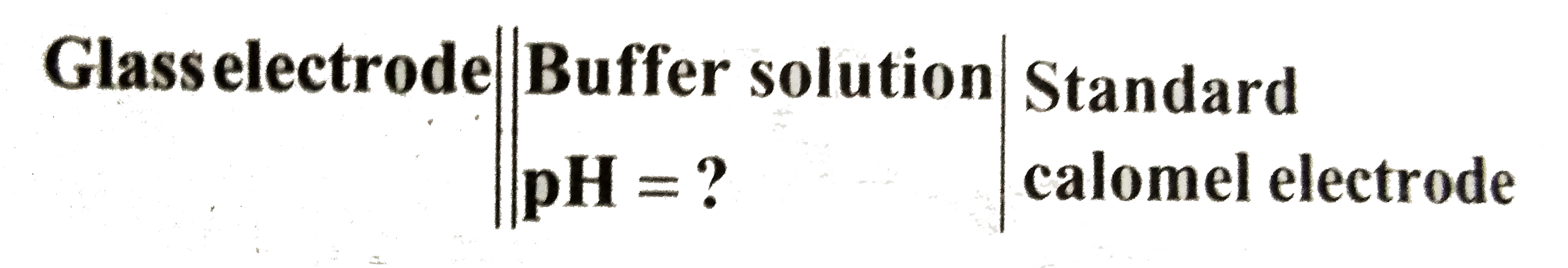
If the EMF of the above cell is 0.03V,ESCE=0.24V,Ec−.glass=0.51V, then calculate the pH of buffer solution at 30∘C.
View SolutionThe e.m.f. of the cell obtained by combining Zn and Cu electrode of a Daniel cell with N calomel electrode in two different arrangements are 1.083V and 0.018V respectively at 25∘C. If the standard reduction potential of N calomel electrode is 0.28V find the emf of Daniel cell.
View SolutionThe oxidation electrode potential E, of a 0.1 M solution of M+ ions (EoRP=−2.36V) is :
View SolutionThe measured voltage of the cell, Pt(s)|H2(1.0atm)|H+(aq)∣∣∣Ag+(1.0M)∣∣Ag(s) is 1.02V at 25∘C . Given E∘cell is 0.80 V, calculate the pH of the solution .
View Solution
