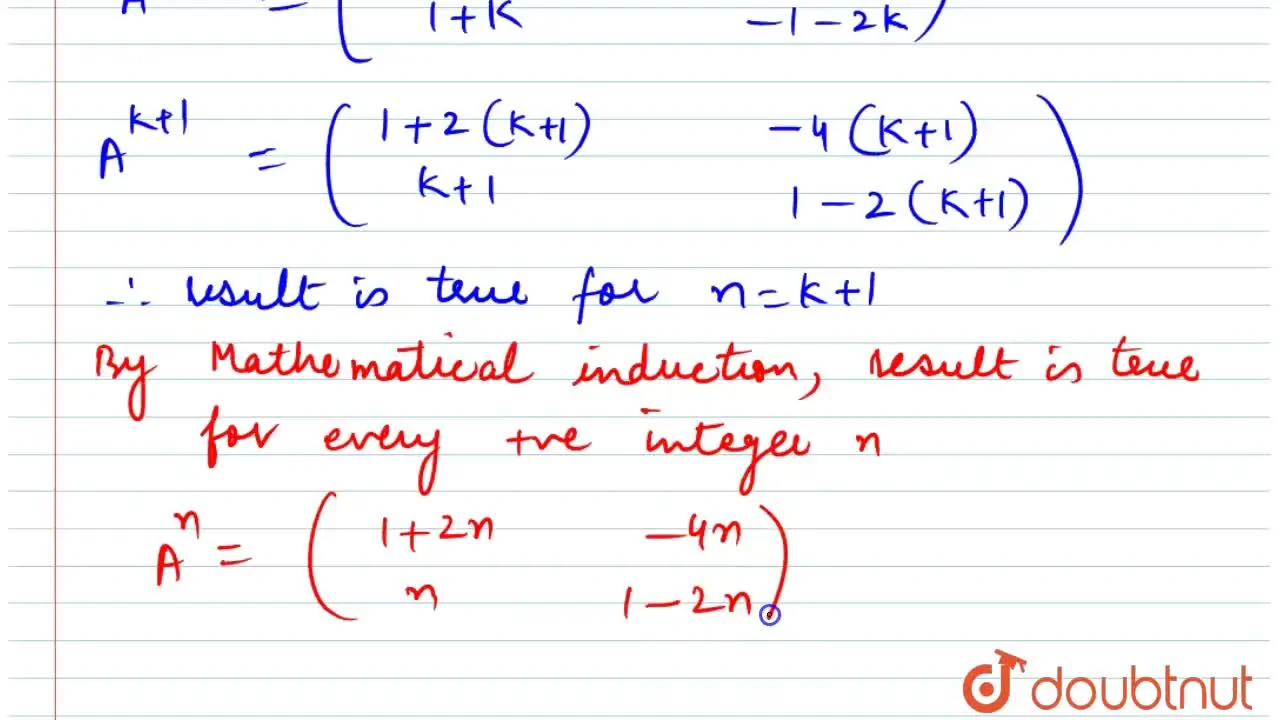
If A=(3−41−1), then prove by Mathematical Induction that : An=(1+2n−4nn1−2n), where n∈NSolution in Punjabi
The correct Answer is:P.M.I., the result is true for any positive integer k
Step by step video, text & image solution for If A= ((3,-4),(1,-1)), then prove by Mathematical Induction that : A^n = ((1+2n,-4n),(n,1-2n)), where n in N by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - MATRICESEXERCISE - Assignment (Section I) M.C.Q10videos
MATRICES
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - MATRICESEXERCISE - Assignment (Section II) Short answer type questions14videos
MATRICES
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - MATRICESEXERCISE - Solve Examples (Section IV) Short answer type questions13videos
MATRICES
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - LINEAR PROGRAMMINGEXERCISE - Previous Years Board.s Questions For Practice10videos
LINEAR PROGRAMMING
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Previous year Board.s questions for practice43videos
PROBABILITY
Similar Questions
If A is matrix of 4×3 then each column of A contains
10:02View SolutionIf a matrix has 8 elements , what are the possible order it can have ?
06:43View SolutionIf A=⎡⎢⎣−123579−211⎤⎥⎦andB=⎡⎢⎣−41−5120131⎤⎥⎦ , then verify that (A+B)'=A'+B'
06:39View SolutionMinimize and Maximize Z=2x+6y subject to the constraints x+2y≤10,x+y≤7,x≥2,x,y≥0
03:49View SolutionIf P(A)=57,P(B)=37 and P(A∪B)=67, then find
(i) P(A∩B)
(ii) P(B/A)05:09View SolutionIf A=[[3,-4] , [1,-1]] then by the method of mathematical induction prove that A^n=[[1+2n,-4n] , [n,1-2n]]
04:04View SolutionProve the following by principle of mathematical induction If A=[3−41−1], then An=[1+2n−4nn1−2n] for every positive integer n
06:09View Solutionयदि A=[3−41−1] तो सिद्ध कीजिए कि An=[1+2n−4nn1−2n] जहाँ n एक धन पूर्णांक है |
03:43View Solutionयदि A=[3−41−1], तो सिद्ध कीजिए कि An=[1+2n−4nn1−2n], जहाँ n एक धन पूर्णांक है।
06:14View SolutionIf A=[3−41−1], then by principle of mathematics induction show that, An=[1+2n−4nn1−2n] for all n∈N.
04:57View SolutionProve by mathematical induction 4 + 8 + 12 +... 4n = 2n(n + 1).
04:22View SolutionLet A=[−1−413], prove by Mathematical Induction that An=[1−2n−4nn1+2n], where n∈N.
03:46View SolutionLet A=[−1−413], by Mathematical Induction prove that :
An[1−2n−4nn1+2n], where n∈N.08:49View SolutionProve the following by principle of mathematical induction If A=[3−41−1], then An=[1+2n−4nn1−2n] for every positive integer n
03:58View SolutionIf A=(−1−413), then prove by Mathematical Induction that : An=(1−2n−4nn1+2n), where n∈N
08:08View SolutionIf A=(3−41−1), then prove by Mathematical Induction that : An=(1+2n−4nn1−2n), where n∈N
08:23View Solution