What is the maximum number of stereoisomers possible for each compound?
CH3CH(OH)CH(OH)CH2CH3
To determine the maximum number of stereoisomers for the compound CH3CH(OH)CH(OH)CH2CH3, we will follow these steps:
Step 1: Identify Chiral Centers
A chiral center is a carbon atom that has four different substituents attached to it. In the given structure, we need to identify the carbon atoms that meet this criterion.
1. The first carbon CH3 is not chiral because it has three hydrogen atoms.
2. The second carbon CH(OH) is chiral because it has four different groups: CH3, OH, H, and the rest of the carbon chain.
3. The third carbon CH(OH) is also chiral for the same reason as the second carbon; it has four different groups attached.
4. The fourth carbon CH2CH3 is not chiral because it has two hydrogen atoms.
Step 2: Count the Number of Chiral Centers
From our analysis, we found that there are 2 chiral centers in the compound.
Step 3: Use the Formula for Stereoisomers
The maximum number of stereoisomers can be calculated using the formula:
Number of stereoisomers=2n
where n is the number of chiral centers.
Step 4: Calculate the Maximum Number of Stereoisomers
Since we have determined that n=2:
Number of stereoisomers=22=4
Conclusion
The maximum number of stereoisomers possible for the compound CH3CH(OH)CH(OH)CH2CH3 is 4.
---
A chiral center is a carbon atom that has four different substituents attached to it. In the given structure, we need to identify the carbon atoms that meet this criterion.
2. The second carbon CH(OH) is chiral because it has four different groups: CH3, OH, H, and the rest of the carbon chain.
3. The third carbon CH(OH) is also chiral for the same reason as the second carbon; it has four different groups attached.
4. The fourth carbon CH2CH3 is not chiral because it has two hydrogen atoms.
From our analysis, we found that there are 2 chiral centers in the compound.
The maximum number of stereoisomers can be calculated using the formula:
Number of stereoisomers=2n
where n is the number of chiral centers.
Since we have determined that n=2:
Number of stereoisomers=22=4
The maximum number of stereoisomers possible for the compound CH3CH(OH)CH(OH)CH2CH3 is 4.
Similar Questions
Knowledge Check
Number of stereoisomers of the given compound is
CH3−CH=CH−CH∣OH−CH3A2B4C3D6
How many stereoisomers are possible for the compound CH3CH=CHCHClCH3?
View SolutionCompound CH3CH2OH is:
View SolutionThe number of stereoisomers possible for a compound of the molecular formula CH3−CH=CH−CH(OH)−Me is
View SolutionThe number of stereoisomers of compound CH3−CH=CH−CH(Br)CH3 is :
View SolutionNumber of stereoisomers of the given compound is
CH3−CH=CH−CH∣OH−CH3
View SolutionThe number of stereo isomers possible for a compound of the molecular formula is CH3−CH=CH−CH(NH2)−CH3 is
View SolutionWrite its bond line formula
(i) CH3CH(OH)CH2CH2OH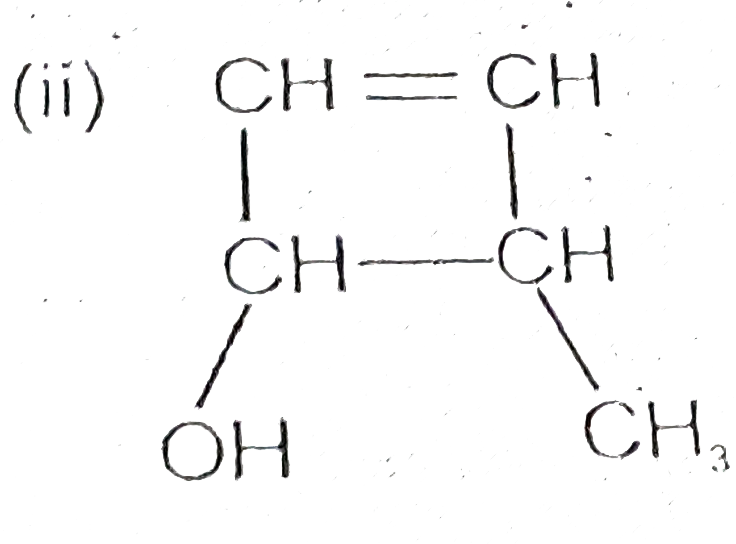
View Solution
