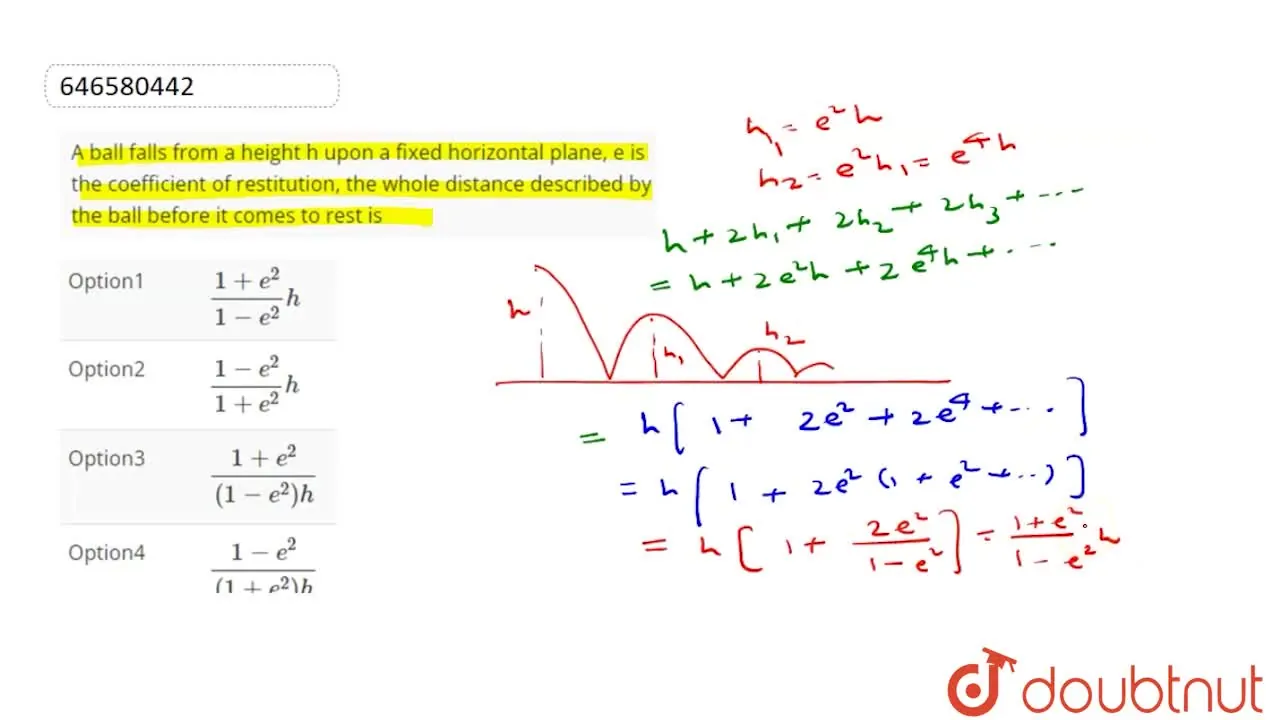
A ball falls from a height h upon a fixed horizontal plane, e is the coefficient of restitution, the whole distance described by the ball before it comes to rest is
1+e21−e2h
1−e21+e2h
1+e2(1−e2)h
1−e2(1+e2)h
The correct Answer is:A
Step by step video, text & image solution for A ball falls from a height h upon a fixed horizontal plane, e is the coefficient of restitution, the whole distance described by the ball before it comes to rest is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A spherical ball falls from a height h on to a floor of coefficient of restitution e . Calculate the total distance described by the ball before it comes to rest .
View SolutionA particle falls from a height h on a fixed horizontal plate and rebounds. If e is the coefficient of restitution, the total distance travelled by the particle before it stops rebounding is
View SolutionA partical falls from a height h upon a fixed horizontal plane and rebounds. If e is the coefficient of restitution, the total distance travelled before rebounding has stopped is
View SolutionA particle falls from a height h upon a fixed horizontal plane and rebounds. If e is the coefficient of restitution, the total distance travelled before rebounding has stopped is :
View SolutionA particle falls from a height h, upon a fixed horizontal plane. If e be the coefficient of restitution, the whole time taken before the particle has finished rebounding is √(2hg)1+e1−e
View SolutionA ball is dropped from a height 'h' on to a floor of coefficient of restitution 'e'. The total distance covered by the ball just before second hit is
View SolutionA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e = 1/2, the total distance travelled before the ball comes to rest is
View SolutionA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e=1/2, the total distance travelled before the ball comes to rest is
View SolutionA ball is dropped from a height h on a horizontal plane and the coefficient of restitution for the impact is e, the velocity with which the ball rebounds from the flor is
View SolutionA ball of mass M falls from a height on a floor for which the coefficient of restitution is e. The height attained by the ball after two rebounds is
View Solution