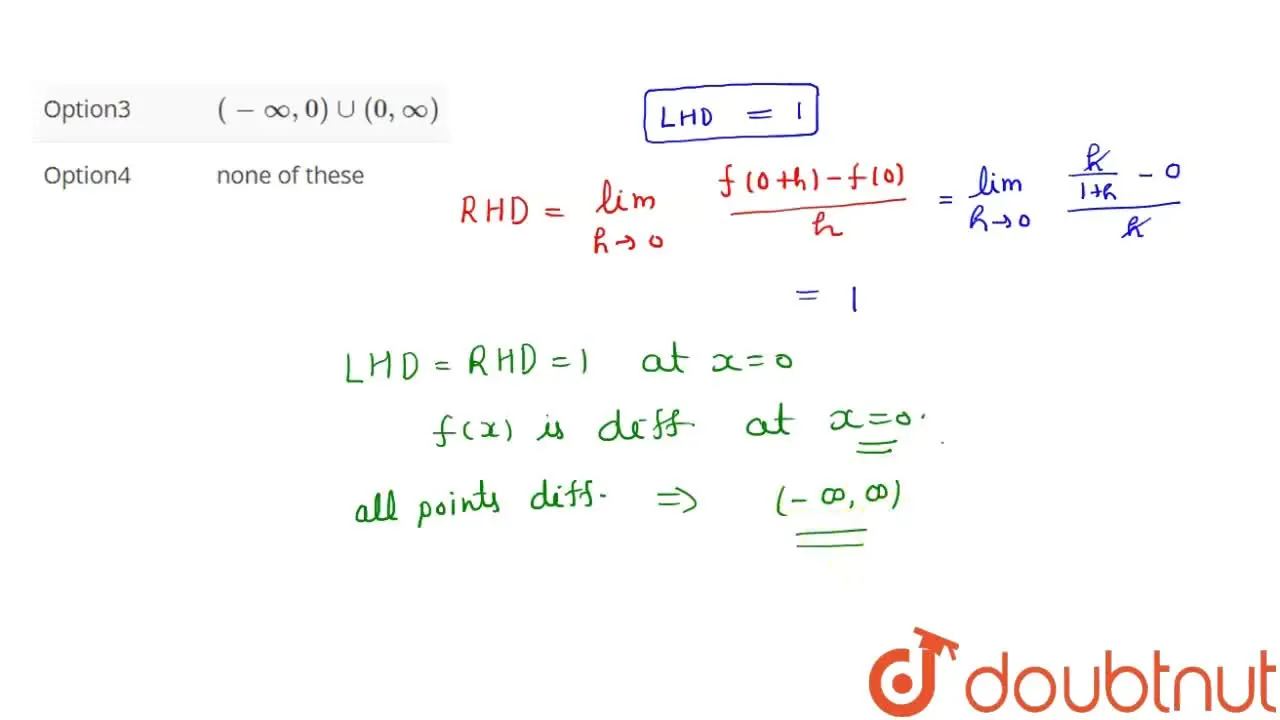
The set of all points, where the function f(x)=x1+|x| is differentiable, is
(−∞,∞)
(−0,∞)
(−∞,0)∪(0,∞)
none of these
The correct Answer is:A
Step by step video, text & image solution for The set of all points, where the function f(x) =x/(1+|x|) is differentiable, is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (2) (TRUE AND FALSE)4videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (2) (FILL IN THE BLANKS)2videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (1) (FILL IN THE BLANKS)7videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - INVERSE CIRCULAR FUNCTIONS EXERCISE - Self Assessment Test25videos
INVERSE CIRCULAR FUNCTIONS
- BOOK - ML KHANNACHAPTER - LINEAR PROGRAMMING EXERCISE - Self Assessment Test8videos
LINEAR PROGRAMMING
Similar Questions
The set of all points, where the function f(x)=x1+|x| is differentiable, is
View SolutionThe set of all points where the function f(x)=3√x2|x| is differentiable, is
View SolutionThe function f(x)=x1+|x| is differentiable in:
View SolutionThe set of all points where the function f(x)=2x|x| is differentiable is
View SolutionThe set of points where the function f(x)=x|x| is differentiable is
View SolutionThe set of points where the function f(x)=|x−2|cosx is differentiable is
View SolutionThe set of all points where the function f(x)=√1−e−x2 is differentiable is
View SolutionThe set of all points where the function f(x)=√1−e−x2 is differentiable is :
View SolutionThe set of points where the function f(x)=|x−1|ex is differentiable, is
View SolutionThe set of points where the function f(x)=x|x| is differentiable is
View Solution