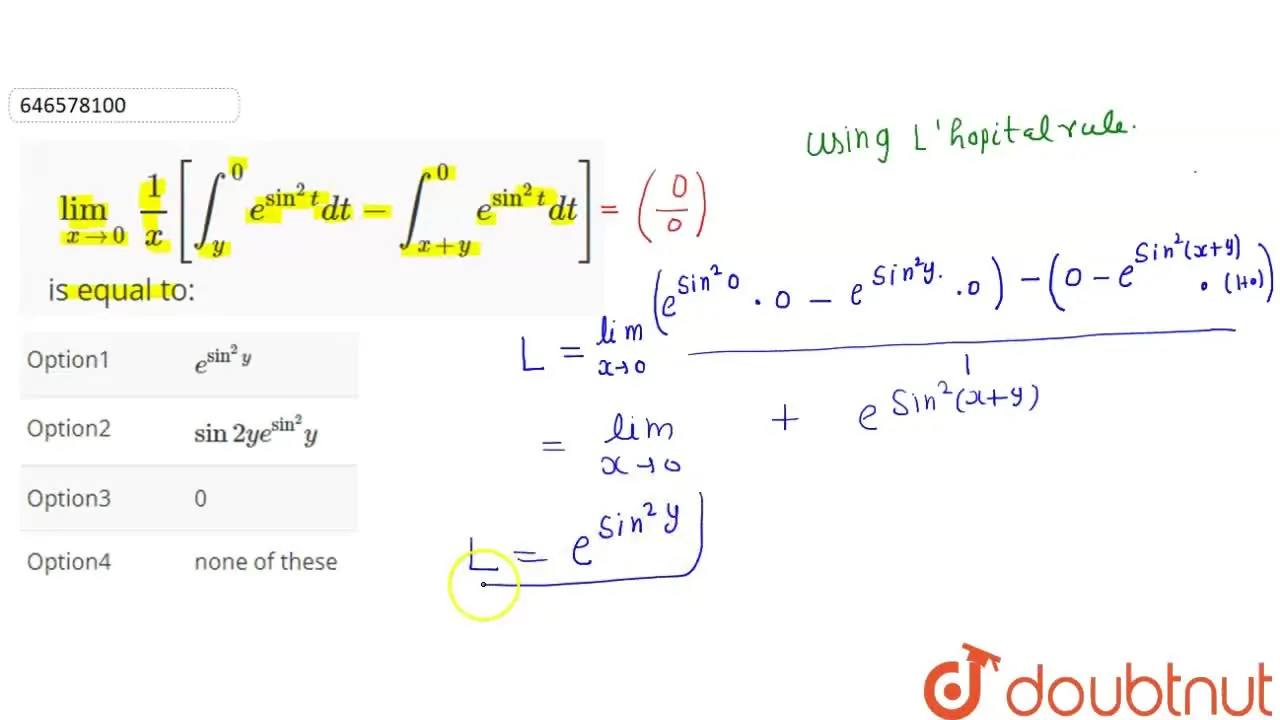
is equal to:
0
none of these
The correct Answer is:A
Step by step video, text & image solution for lim_(x to 0)1/x[int_(y)^(0) e^(sin^(2)t) dt - int_(x+y)^(0) e^(sin^(2)t) dt] is equal to: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (1) (TRUE AND FALSE)4videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (1) (FILL IN THE BLANKS)7videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - INVERSE CIRCULAR FUNCTIONS EXERCISE - Self Assessment Test25videos
INVERSE CIRCULAR FUNCTIONS
- BOOK - ML KHANNACHAPTER - LINEAR PROGRAMMING EXERCISE - Self Assessment Test8videos
LINEAR PROGRAMMING
Similar Questions
limx→01x[∫0yesin2tdt−∫0x+yesin2tdt] is equal to:
View Solutionlimx→01x[∫ayesin2tdt−∫ax+yesin2tdt] is equal to
View Solution(lim)n→∞1x[∫ayesin2t)dt−∫ax+yesin2t)dt−]isequa<o (0,1] (b) [1,∞) (0,∞) (d) none of these
View Solutionlimx→0∫x20(tan−1t)dt∫x20sin√tdt is equal to :
View SolutionThe value of limx→∞(∫x+y0et2dt)2∫x+y0e2t2dt is equal to (i)0 (ii)1 (iii) limx→0(∫x0cost2x)−1 (iv)−1
View Solutionlimx→∞(∫x0et2dt)2∫x0e2t2dt is equal to
View Solutionlimx→01x[∫y→aesin2tdt−∫x+y→aesin2tdt] is equal to
View SolutionThe value of limx→0∫x0sint2dtx2 is
View SolutionIf k=∫10et1+tdt, then ∫10etloge(1+t)dtis equal to
View SolutionThe value of limx→0+∫cosx1(cos−1t)dt2x−sin2x is equal to:
View Solution