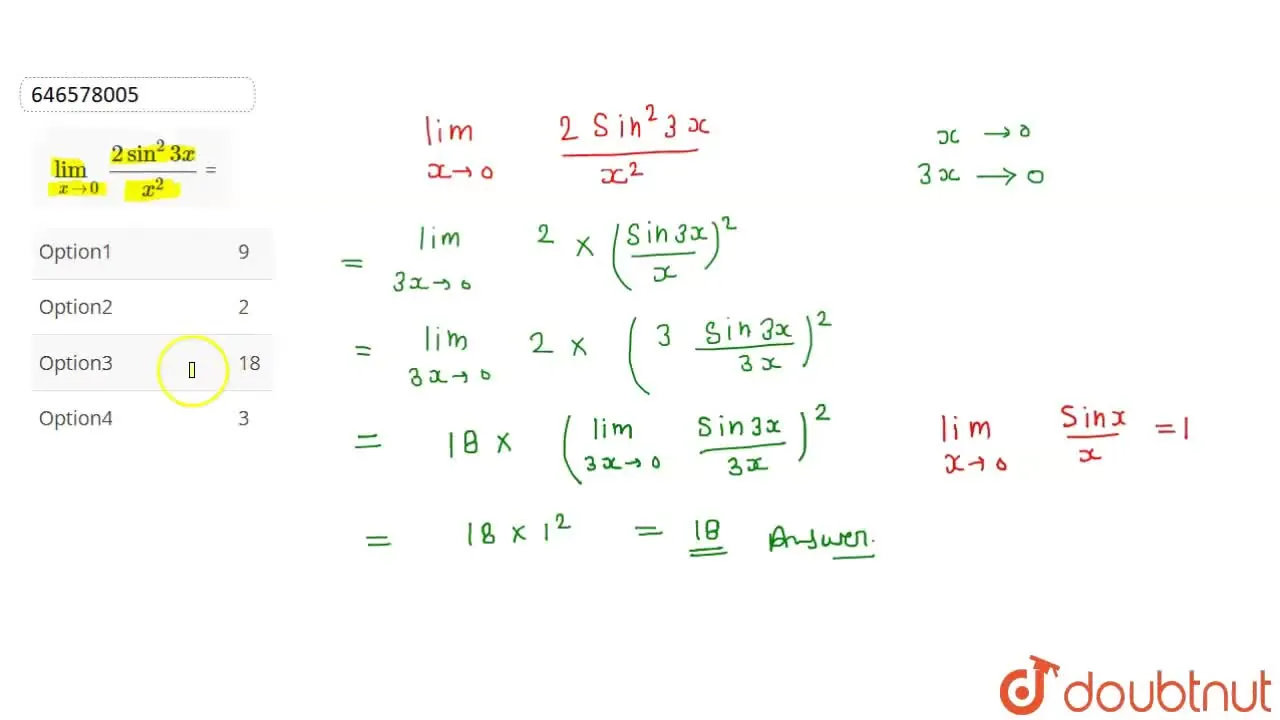limx→02sin23xx2 =
A
9
B
2
C
18
D
3
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for lim_(x to 0) (2 sin^(2) 3x)/x^(2) = by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (1) (TRUE AND FALSE)4videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - LIMITS, CONTINUITY AND DIFFERENTIABILITY EXERCISE - PROBLEM SET (1) (FILL IN THE BLANKS)7videos
LIMITS, CONTINUITY AND DIFFERENTIABILITY
- BOOK - ML KHANNACHAPTER - INVERSE CIRCULAR FUNCTIONS EXERCISE - Self Assessment Test25videos
INVERSE CIRCULAR FUNCTIONS
- BOOK - ML KHANNACHAPTER - LINEAR PROGRAMMING EXERCISE - Self Assessment Test8videos
LINEAR PROGRAMMING
Similar Questions
Evaluate limx→0sin24xx2
View SolutionEvaluate the following :
limx→0sin2x2xView Solutionlimx→0sin(πcos2x)x2 is equal to
View SolutionEvaluate limx→0sin2(3x/2x)x
View Solutionlimx→0x+2sinx√x2+2sinx+1−√sin2x−x+1is
View Solutionlimx→0(sinx+cos3x)2/x =
View Solutionlimx→0x2sin2xx2−sin2x
View Solutionlimx→0xtan3xsin2x is
View Solutionlimx→02sinx−sin2xx3 ie equal to
View SolutionIf limx→0(x−3sin3x+ax−2+b) exists and is equal to 0, then
View Solution