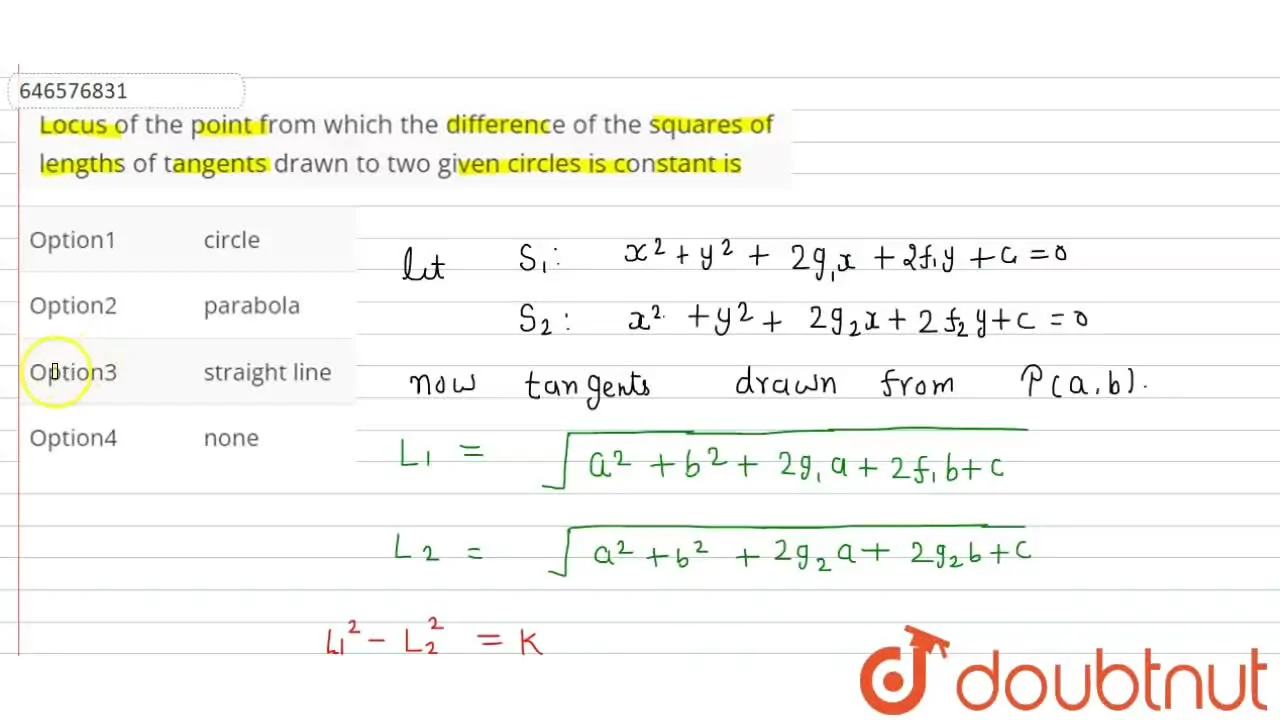
Locus of the point from which the difference of the squares of lengths of tangents drawn to two given circles is constant is
circle
parabola
straight line
none
The correct Answer is:C
Step by step video, text & image solution for Locus of the point from which the difference of the squares of lengths of tangents drawn to two given circles is constant is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - THE CIRCLE EXERCISE - Problem Set (6) (TRUE AND FALSE)1videos
THE CIRCLE
- BOOK - ML KHANNACHAPTER - THE CIRCLE EXERCISE - Problem Set (7) (MULTIPLE CHOICE QUESTIONS)23videos
THE CIRCLE
- BOOK - ML KHANNACHAPTER - THE CIRCLE EXERCISE - Problem Set (5) (FILL IN THE BLANKS)2videos
THE CIRCLE
- BOOK - ML KHANNACHAPTER - TANGENTS AND NORMALSEXERCISE - SELF ASSESSMENT TEST (MULTIPLE CHOICE QUESTIONS)19videos
TANGENTS AND NORMALS
- BOOK - ML KHANNACHAPTER - THE ELLIPSEEXERCISE - SELF ASSESSMENT TEST9videos
THE ELLIPSE
Similar Questions
Locus of the point, the difference of the square of length of the tangents drawn from which to two given circle is constant is :
View SolutionThe locus of a point which moves such that the difference of the squares of lengths of tangents drawn from it to two given circles is constant, is :
View SolutionShwo that the difference of the squares of the tangents to two coplanar circles from any point P in the plane of the circles varies as the perpendicular from P on their radical axis. Also, prove that the locus of a point such that the difference of the squares of the tangents from it to two given circles is constant is a line parallel to their radical axis.
View SolutionThe locus of a point which moves so that the difference of the squares of its distances from two given points is constant, is a
View SolutionThe locus of a point which moves so that the difference of the squares of its distance from two given points is constant, is a
View SolutionTwo points PandQ are taken on the line joining the points A(0.0)andB(3a,0) such that AP=PQ=QB. Circles are drawn on AP,PQandQB as diameters. The locus of the point S from which, the sum ofsquares of the lengths of the tangents to the three circles is equal to b2 is
View SolutionA and B are two points (0,0) and (3a,0) respectively. Points P and Q are taken on AB such that AP=PQ=QB. Circles are drawn on AP, PQ and QB as diameters. If T be the point from where the sum of the squares of the lengths of tangents to these three circles be b2 then locus of the point T is
View SolutionLength of tangent from a Point to a Circle.
View SolutionNumber of tangents drawn from a point inside the circle is :
View SolutionProve that the locus of a point, which moves so that its distance from a fixed line is equal to the length of the tangent drawn from it to a given circle, is a parabola.
View Solution