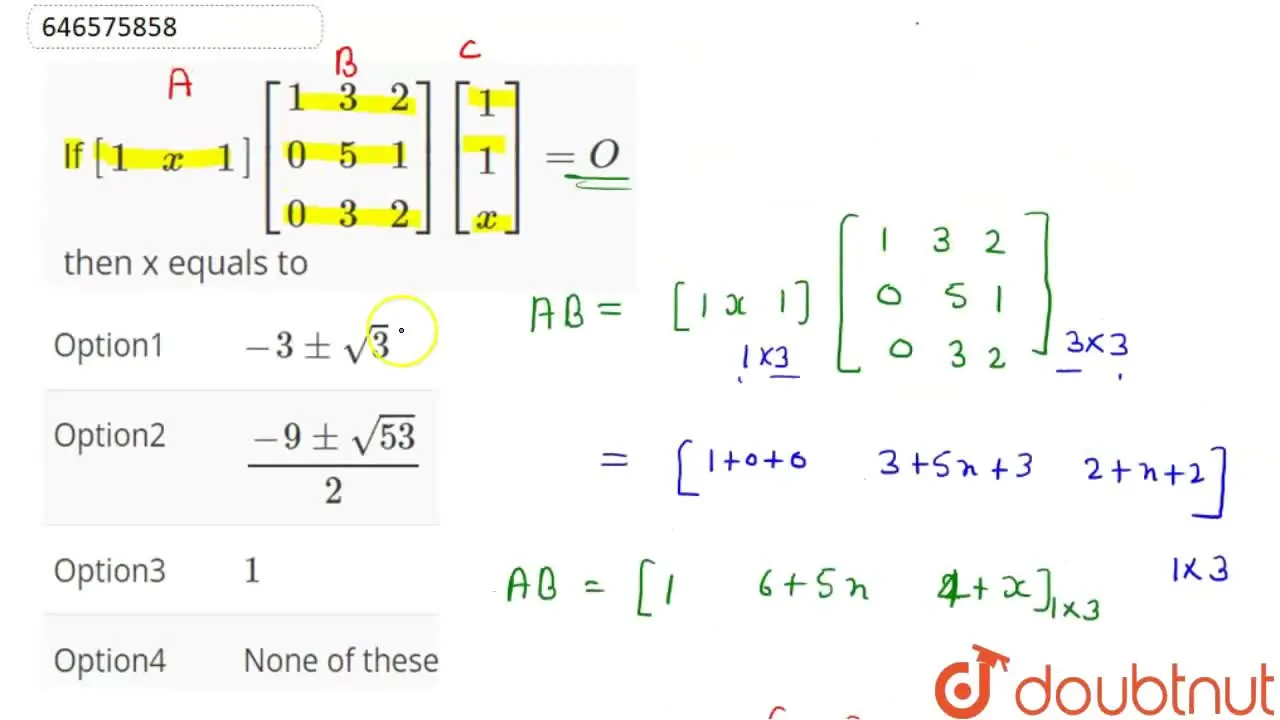
If [1x1]⎡⎢⎣132051032⎤⎥⎦⎡⎢⎣11x⎤⎥⎦=O then x equals to
−3±√3
−9±√532
1
None of these
The correct Answer is:B
Step by step video, text & image solution for If [(1,x,1)][(1,3,2),(0,5,1),(0,3,2)][(1),(1),(x)]=O then x equals to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - MATRICESEXERCISE - PROBLEM SET(1) (ASSERTION /REASON)3videos
MATRICES
- BOOK - ML KHANNACHAPTER - MATRICESEXERCISE - PROBLEM SET(1) (TRUE AND FALSE)9videos
MATRICES
- BOOK - ML KHANNACHAPTER - MATRICESEXERCISE - EXAMPLE4videos
MATRICES
- BOOK - ML KHANNACHAPTER - MATHEMATICAL REASONINGEXERCISE - PROBLEM SET (2) ASSERTION/REASON3videos
MATHEMATICAL REASONING
- BOOK - ML KHANNACHAPTER - MAXIMA AND MINIMA EXERCISE - MISCELANEOUS EXERCISE (COMPREHENSION)3videos
MAXIMA AND MINIMA
Similar Questions
If [1x1]⎡⎢⎣132051032⎤⎥⎦⎡⎢⎣x1−2⎤⎥⎦=[0] then x is
View SolutionIf [1λ1]⎡⎢⎣132051032⎤⎥⎦⎡⎢⎣λ1−2⎤⎥⎦=O then λ=
View SolutionIf [1 x 1]⎡⎢⎣123051032⎤⎥⎦,⎡⎢⎣x1−2⎤⎥⎦=0, then the value of x is
View SolutionIf [1x1]⎡⎢⎣123051032⎤⎥⎦⎡⎢⎣x1−2⎤⎥⎦=O, then x =
View SolutionIf [1x1]⎡⎢⎣132051020⎤⎥⎦⎡⎢⎣11x⎤⎥⎦=0 then x is
View SolutionFind the value of x, if [1x1]⎡⎢⎣1322511532⎤⎥⎦⎡⎢⎣12x⎤⎥⎦=0
View SolutionIf [1x1]⎡⎢⎣132051032⎤⎥⎦⎡⎢⎣x1−2⎤⎥⎦=0, then x is
View SolutionIf [1x1]⎡⎢⎣123051032⎤⎥⎦⎡⎢⎣x1−2⎤⎥⎦=0, then the value of x is
View Solution[1 x 1 ] ⎡⎢⎣1322511532⎤⎥⎦⎡⎢⎣12x⎤⎥⎦ = 0, if x =
View SolutionSolve the matrix equations: [x−5−1]⎡⎢⎣102021203⎤⎥⎦⎡⎢⎣x41⎤⎥⎦=0
View Solution