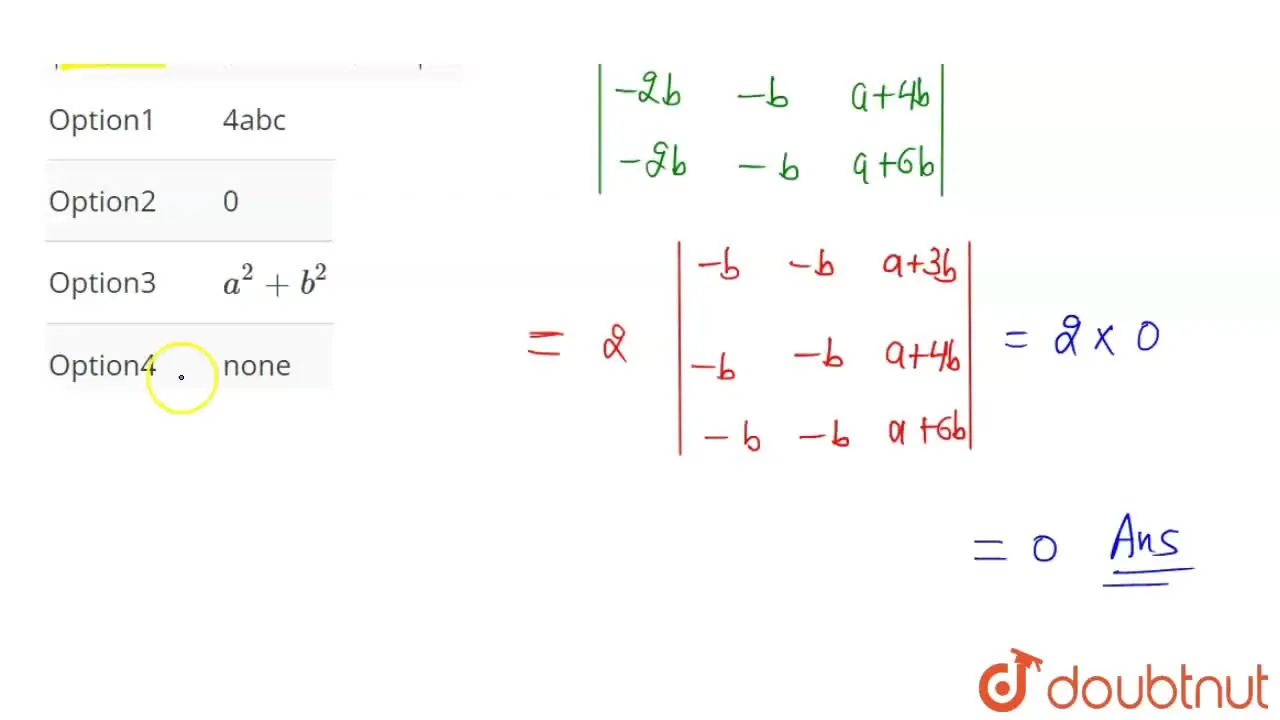
The value of the determinant is
4abc
0
none
The correct Answer is:B
Step by step video, text & image solution for The value of the determinant |(a+b,a+2b,a+3b),(a+2b,a+3b,a+4b),(a+4b,a+5b,a+6b)| is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - DETERMINANTS EXERCISE - Problem Set (1) (TRUE AND FALSE)7videos
DETERMINANTS
- BOOK - ML KHANNACHAPTER - DETERMINANTS EXERCISE - Problem Set (2) (MULTIPLE CHOICE QUESTIONS)21videos
DETERMINANTS
- BOOK - ML KHANNACHAPTER - DEFINITE INTEGRALEXERCISE - Miscellaneous Questions (Assertion/Reason)1videos
DEFINITE INTEGRAL
- BOOK - ML KHANNACHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - MISCELLANEOUS EXERCISE (Matching Entries)2videos
DIFFERENTIAL EQUATIONS
Similar Questions
Without expanding the determinant, prove that
∣∣ ∣∣a+b2a+b3a+b2a+b3a+b4a+b4a+b5a+b6a+b∣∣ ∣∣=0.View SolutionThe determinant ∣∣ ∣∣a+b+ca+ba4a+3b+2c3a+2b2a10a+6b+3c6a+3b3a∣∣ ∣∣ is independent of which one of the following ?
View SolutionWithout expanding, show that the value of each of the determinants is zero: |a+b2a+b3a+b2a+b3a+b4a+b4a+b5a+b6a+b|
View SolutionProve that ∣∣ ∣∣a+ba+2ba+3ba+3ba+4ba+5ba+5ba+6ba+7b∣∣ ∣∣=0
View SolutionIf a=i,b=ωandC=ω2, then the value of determinant ∣∣ ∣∣aa+ba+b+c3a4a+3b5a+4b+3c6a9a+6b11a+9a+6c∣∣ ∣∣
View SolutionThe value of the product (4a2+3b)(9b2+4a) at a=1,b=−2 is
View Solutionthe value of the determinant
∣∣ ∣ ∣ ∣ ∣∣(a1−b1)2(a1−b2)2(a1−b3)2(a1−b4)2(a2−b1)2(a2−b2)2(a2−b3)2(a3−b4)2(a3−b1)2(a3−b2)2(a3−b3)2(a3−b4)2(a4−b1)2(a4−b2)2(a4−b3)2(a4−b4)2∣∣ ∣ ∣ ∣ ∣∣ isView SolutionThe three points [(a+b)(a+2b),(a+b)],[(a+2b)(a+3b),(a+2b)] and [(a+3b)(a+4b),(a+3b)]
View SolutionSuppose a,b,cεR and
Δ=∣∣ ∣∣aa+ba+b+c3a4a+3b5a+4b+3c6a9a+6b11a+9b+6c∣∣ ∣∣ If Δ=11.728 then a=______View SolutionFind the value of the products: (4a2+3b)(4a2+3b)at a=1, b=2.
View Solution