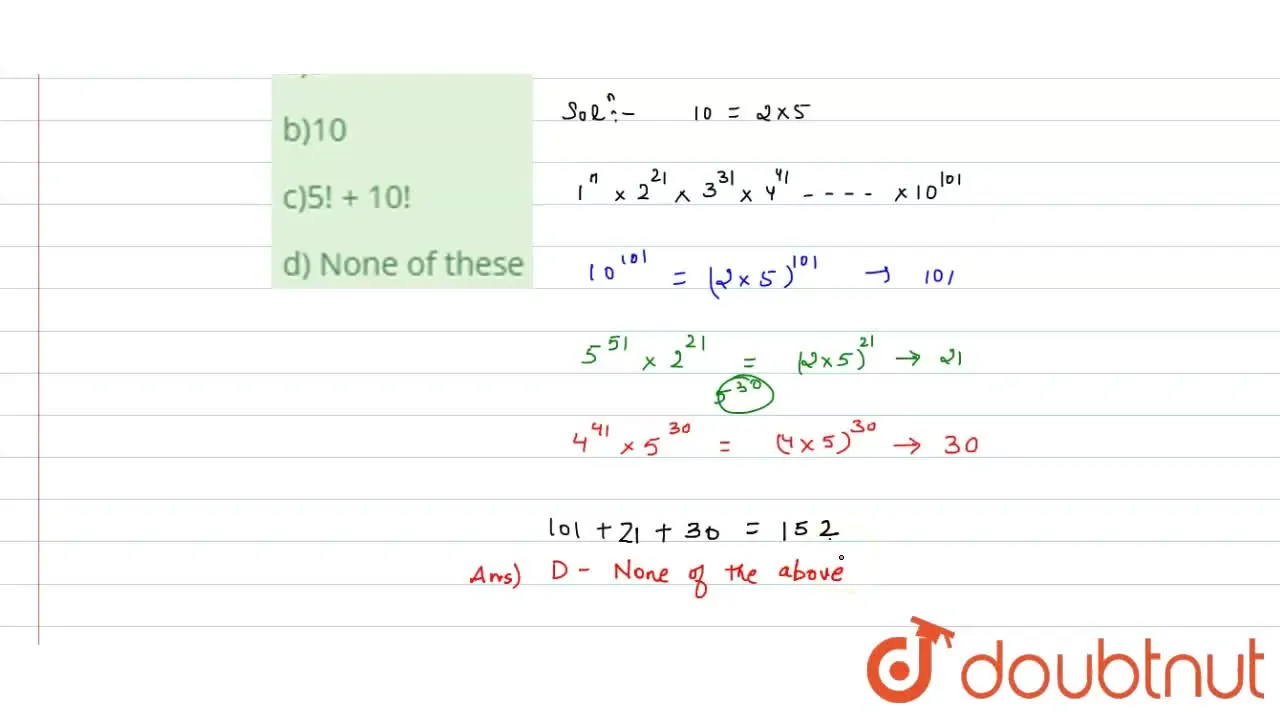
Find the number of Zeros at the end of the product -
111×221×331×441.......10101
a)51
b)10
c)5! + 10!
d) None of these
The correct Answer is:c
Step by step video, text & image solution for Find the number of Zeros at the end of the product - 1^11 xx 2^21 xx 3^31 xx 4^41 ....... 10^101 by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
Find the number of Zeros at the end of the product -
25!x32!x45!View SolutionFind the number of zeros at the end of the product of 2222×5555
View SolutionFind the number of Zeros at the end of the product -
1×3×5×7×9×11......99×101View SolutionFind the number of Zeros at the end of the product -
140!×5×15×22×11×44×135View SolutionFind the number of Zeros at the end of the product -
33×44×55......4949View SolutionFind the number of Zeros at the end of the given product
15×25×35......325View SolutionFind the number of Zeros at the end of the product -
12×5×15×24×13×30×75View SolutionFind the number of Zeros at the end of the product -
12×27×63×113×1250×24×650View SolutionFind the number of zeros in the end of the product of 2222×5555.
View SolutionFind the number of Zeros at the end of the product -
23×54×42×108×610×1512×814X2016×1018×2520View Solution