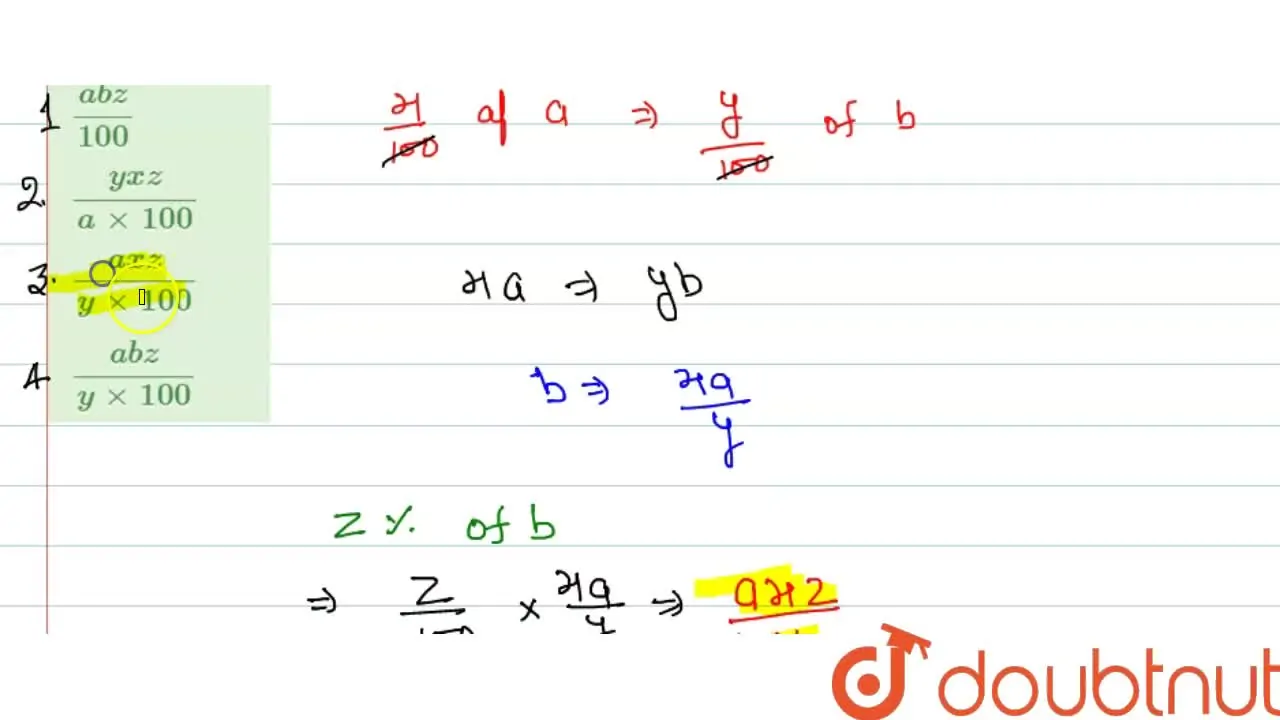
If of of b, then of b is
A
B
C
D
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for If x% of a=y% of b, then z% of b is by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
x% of y+y% of x=?
View SolutionIf x% of 50= y% of 60 then x:y=
View Solutionx% of y is y% of ?
View SolutionIf x% of a is the same as y% of b, then z% of b will be
View Solutionx% of y is y% of ?
View SolutionIf 60% of A=30% of B, B=40% of C and C=x% of A, then value of x is
View SolutionIf 40% of x=y, then y% of 40 is same as ______of x.
View SolutionIf x% of a equals to y of b, then find z% of b:
View SolutionIf x% of y% of 80 is the same as 25% of 900, then the value of xy is
View Solution2% of a = b, then b% of 10 is the same as
View Solution