A student scored 70%,80%,50%and48% in four papers. It was decided to give double weight age to the 1st and the 3rd paper and half to the 2nd and 4th paper. Find the changed average marks.
The correct Answer is:60.8%
To find the changed average marks of the student after applying the specified weightages to the scores, we can follow these steps:
Step 1: Identify the Scores
The scores in the four papers are:
- Paper 1 (x1) = 70%
- Paper 2 (x2) = 80%
- Paper 3 (x3) = 50%
- Paper 4 (x4) = 48%
Step 2: Determine the Weightages
The weightages for each paper are:
- Weightage for Paper 1 (w1) = 2 (double weightage)
- Weightage for Paper 2 (w2) = 0.5 (half weightage)
- Weightage for Paper 3 (w3) = 2 (double weightage)
- Weightage for Paper 4 (w4) = 0.5 (half weightage)
Step 3: Calculate the Weighted Scores
Now, we will calculate the weighted scores for each paper:
- Weighted score for Paper 1 = w1 * x1 = 2 * 70 = 140
- Weighted score for Paper 2 = w2 * x2 = 0.5 * 80 = 40
- Weighted score for Paper 3 = w3 * x3 = 2 * 50 = 100
- Weighted score for Paper 4 = w4 * x4 = 0.5 * 48 = 24
Step 4: Sum of Weighted Scores
Now, we add all the weighted scores together:
Total weighted score = 140 + 40 + 100 + 24 = 304
Step 5: Calculate Total Weightage
Next, we calculate the total weightage:
Total weightage = w1 + w2 + w3 + w4 = 2 + 0.5 + 2 + 0.5 = 5
Step 6: Calculate the Changed Average Marks
Finally, we calculate the changed average marks using the formula:
Changed Average = Total Weighted Score / Total Weightage
Changed Average = 304 / 5 = 60.8
Final Answer
The changed average marks of the student is 60.8%.
---
The scores in the four papers are:
- Paper 1 (x1) = 70%
- Paper 2 (x2) = 80%
- Paper 3 (x3) = 50%
- Paper 4 (x4) = 48%
The weightages for each paper are:
- Weightage for Paper 1 (w1) = 2 (double weightage)
- Weightage for Paper 2 (w2) = 0.5 (half weightage)
- Weightage for Paper 3 (w3) = 2 (double weightage)
- Weightage for Paper 4 (w4) = 0.5 (half weightage)
Now, we will calculate the weighted scores for each paper:
- Weighted score for Paper 1 = w1 * x1 = 2 * 70 = 140
- Weighted score for Paper 2 = w2 * x2 = 0.5 * 80 = 40
- Weighted score for Paper 3 = w3 * x3 = 2 * 50 = 100
- Weighted score for Paper 4 = w4 * x4 = 0.5 * 48 = 24
Now, we add all the weighted scores together:
Total weighted score = 140 + 40 + 100 + 24 = 304
Next, we calculate the total weightage:
Total weightage = w1 + w2 + w3 + w4 = 2 + 0.5 + 2 + 0.5 = 5
Finally, we calculate the changed average marks using the formula:
Changed Average = Total Weighted Score / Total Weightage
Changed Average = 304 / 5 = 60.8
The changed average marks of the student is 60.8%.
Topper's Solved these Questions
Similar Questions
A student scored 70%,80%,50%and48% in four papers. It was decided to give double weight age to the 1st and the 3rd paper and half to the 2nd and 4th paper. What would have been the average had the weightage system been inter changed?
View SolutionA student scored 70%,80%,50%and48% in four papers. It was decided to give double weight age to the 1st and the 3rd paper and half to the 2nd and 4th paper. By what % does it change from the average before the decision was taken?
View Solution
Knowledge Check
In mathematics exam. A student scored 30% marks in the first paper out of a total of 180. How much should he score in second paper out of a total of 150. if he is to get an overall average of atleast 50%?
A74%B76%C70%D80%48% of the 1st number is 60% of the 2nd number. What is the ratio of the 1st number to the 2nd number?
A4:7B3:4C5:4DCouldn't be determinedOne-half of a certain number is equal to 65% of the 2nd number. Find the ratio of 1st to 2nd number.
A10:13B8:13C13:8D13:10
In a proportion, the 1st, 2nd and 4th terms are 51, 68 and 108 respectively. Find the 3rd term.
View SolutionSarita secures 84% marks in hindi paper. If the maximum marks in the paper are 150 find the marks secured by her in the paper.
View SolutionSarita secured 84% marks in Hindi paper. If the maximum marks in the paper are 150, find the marks secured by her in the paper.
View SolutionIn a hostel 60% of the students read Hindi news paper, 40% read English news paper and 20% read both Hindi and English news papers. A student is selected at random.(a) Find the probability that she reads neither Hindi nor English news papers.(b) If she reads Hindi news paper, find the probability that she reads English news paper.(c) If she reads English news paper, find the probability that she reads Hindi news paper.
View SolutionThe full marks for a paper is 300. The break-up of the marks into theory (X), practical (Y) and project (Z), which are the three components of evaluation is 6:5:4. In order to pass one has to score at least 40%, 50% and 50% respectively in X, Y, Z and 60% in aggregate. The marks scored by four students A, B, C and D are shown in the given Bar Graph.
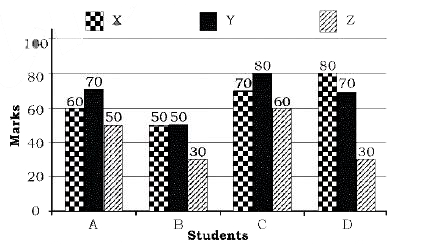
What is the average marks of the four students is theory?
View Solution
