Statement 1 : In ΔABC, −−→AB+−−→BC+−−→CA=0
Statement 2 : If −−→OA=→a,−−→OB=→b, then −−→AB=→a+→bSolution in Punjabi
Both Statement I and Statement II are correct and statement II is the correct explanation of statement I
Both statement I and statement II are correct but statement II is not the correct explanation of statement I
Statement I is correct but statement II is incorrect
Statement II is correct but statement I is incorrect
The correct Answer is:C
In ΔABC,AB+BC=AC=−CA
or AB+BC+CA=0
OA+AB=OB is the triangle law of addition.
Hence, statement 1 is true and statement 2 is false.
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Passage Based Questions)
11 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Matching Type Questions)
1 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (More Than One Correct Option Type Questions)
7 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
Let OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel. Also, OA:CB=2:1 and OD:AB=1:3.
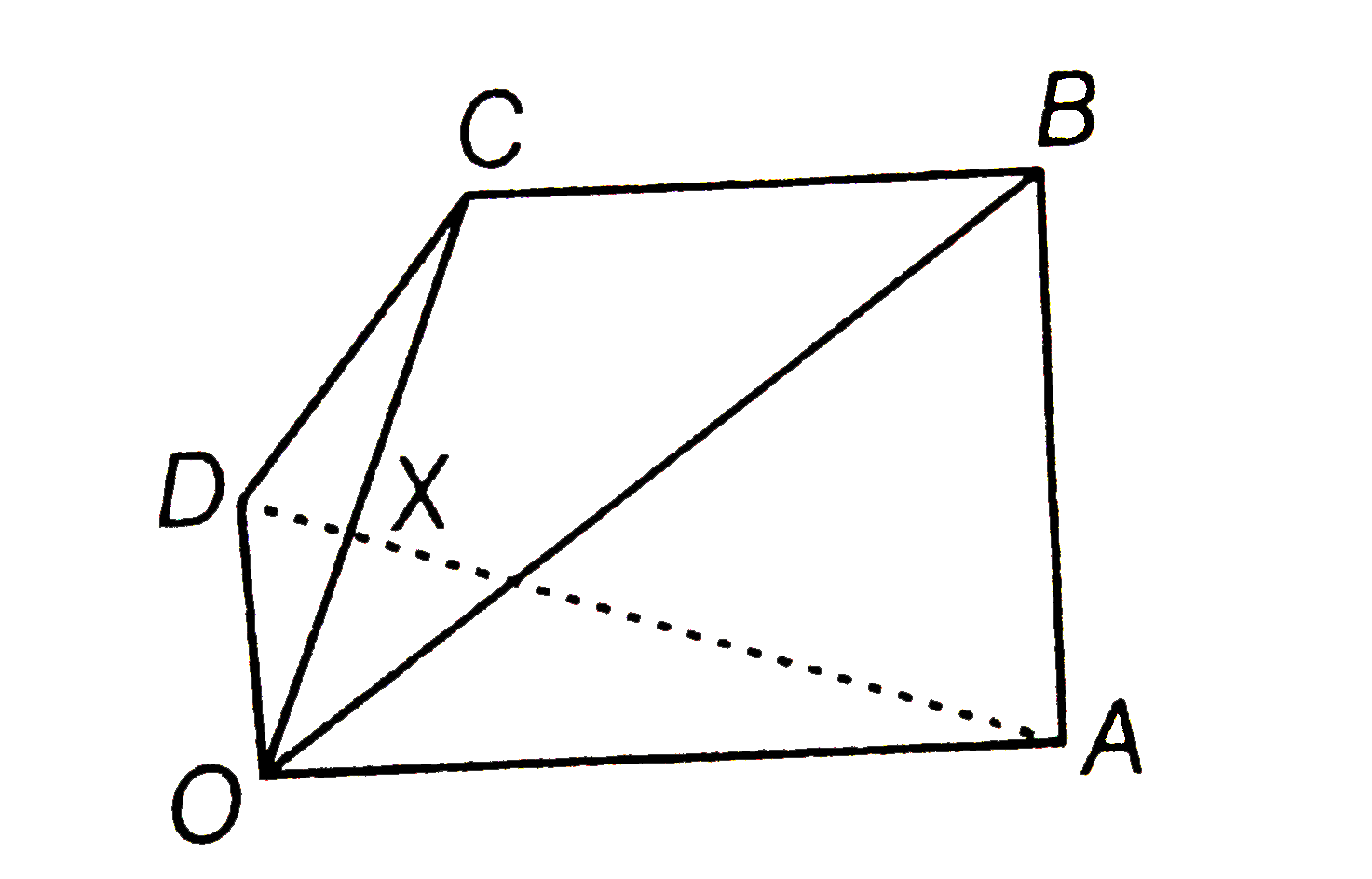
Q. The ratio AXXD is
View Solutiona and b form the consecutive sides of a regular hexagon ABCDEF.
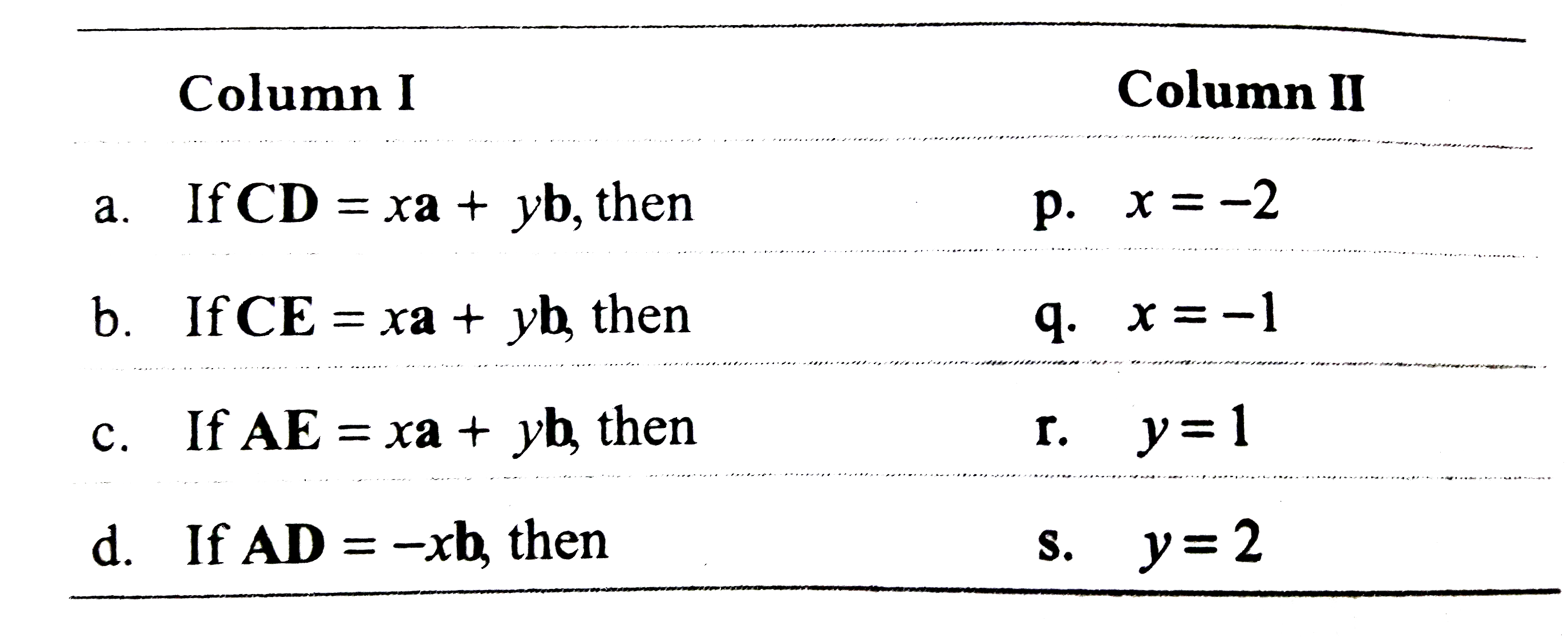
View SolutionIf the vectors ˆi−ˆj,ˆj+ˆkand→a form a triangle then →a may be
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionStatement 1: In DeltaABC,→AB+→BC+→CA=0 Statement 2: If →OA=→a,→OB=→b,then→AB=→a+→b
View SolutionStatement-1:If ,∣∣→A+→B∣∣=∣∣→A−→B∣∣ then angle between vecA and vecB is 90^(@)
Statement-2 :vecA+vecB=vecB+vecA
View SolutionLet →a,→b,→c represent respectively −−→BC,−−→CAand−−→AB where ABC is a triangle , Then ,
View Solutionयदि −−→OA=→a,−−→OB=→b तो सदिश −−→BA का मान बताओ।
View Solutionदिए गए चित्र में −−→OA=→a,−−→AB=→b तो −−→OB और −−→CD ज्ञात करो जबकि C,D क्रमशः OA और AB के मध्य बिंदु है।
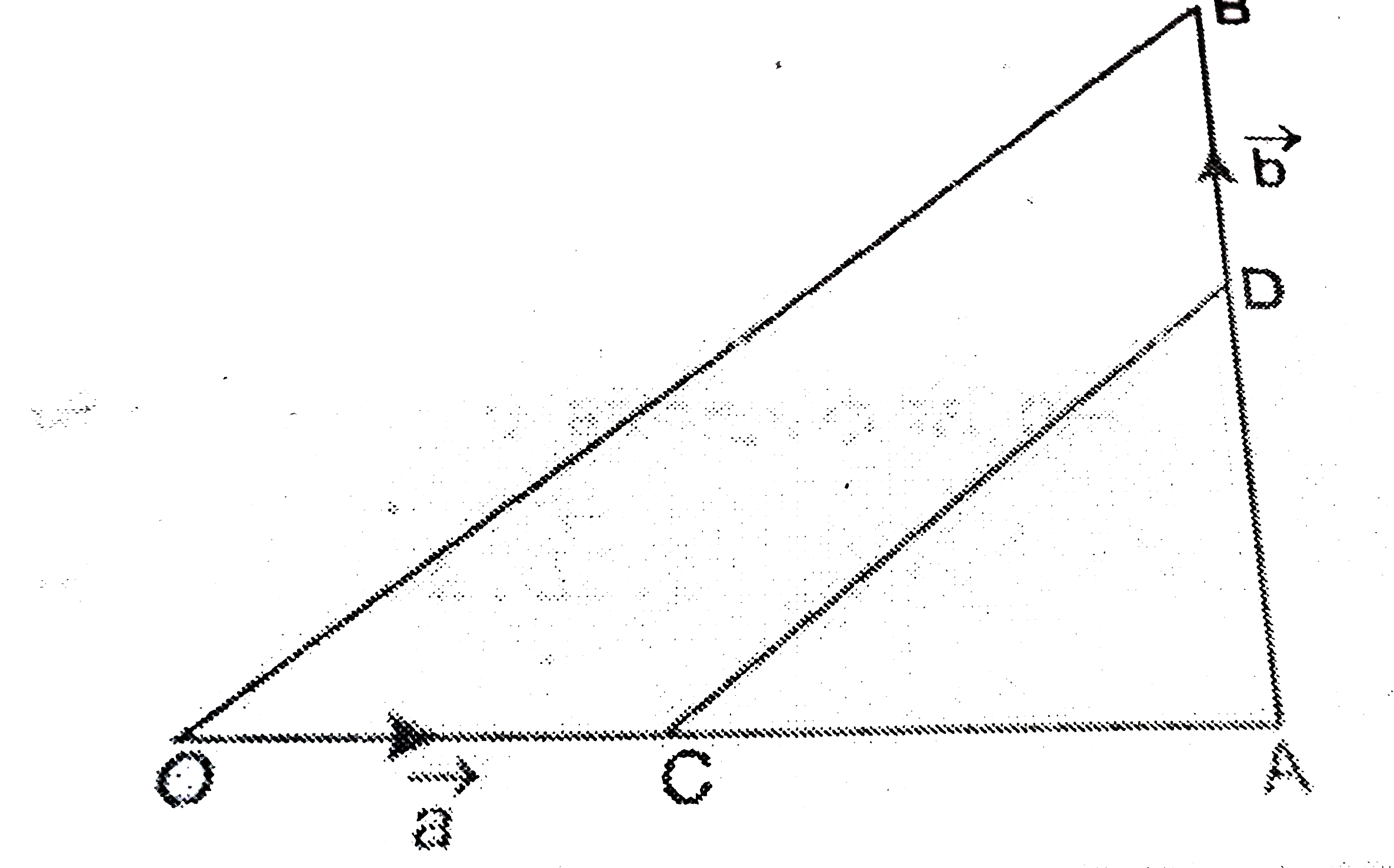
View SolutionOrthocenter of an equilateral triangle ABC is the origin O. If −−→OA=→a,−−→OB=→b,−−→OC=→c, then −−→AB+2−−→BC+3−−→CA=
View SolutionΔABC के लिए निम्नलिखित में से कोण-सा कथन सत्य नहीं है|
(i) −−→AB+−−→BC+−−→CA=0, (ii) −−→AB+−−→BC−−−→AC=0
(c ) −−→AB+−−→BC−−−→CA=0, (d) −−→AB−−−→CB+−−→CA=0
View SolutionIf →a,→b,→c are unit vectors such that →a+→b+→c=→o, then the value of →a⋅→b+→b⋅→c+→c⋅→a is -
View Solutionयदि →a,→b,→c कोई सदिश है , तब (→ab+→ca+→bc) बराबर है -
View SolutionIf →a⋅→b=→b⋅→c=→c⋅→a=0, then the value of [→a,→b,→c] is :
View SolutionIf [→a,→b,→c]=1, then the value of →a⋅(→b×→c)(→c×→a)⋅→b+→b⋅(→c×→a)(→a×→b)⋅→c+→c⋅(→a×→b)(→c×→b)⋅→a is
View SolutionvecA+vecB+vec C=0 If so, show that, vecA×vecB=vecB×vec C=vec C×vecA
View Solution
