ABC is an isosceles triangle right angled at A. forces of magnitude 2√2,5and6 act along BC, CA and AB respectively. The magnitude of their resultant force isSolution in Punjabi
4
5
11+2√2
30
The correct Answer is:B
R cosθ=6cos0∘+2√2cos(180∘−B)+5sin270∘
Rcosθ=6−2√2cosB . . (i)
Rsinθ=6sin0∘+2√2sin(180∘−B)+5sin270∘
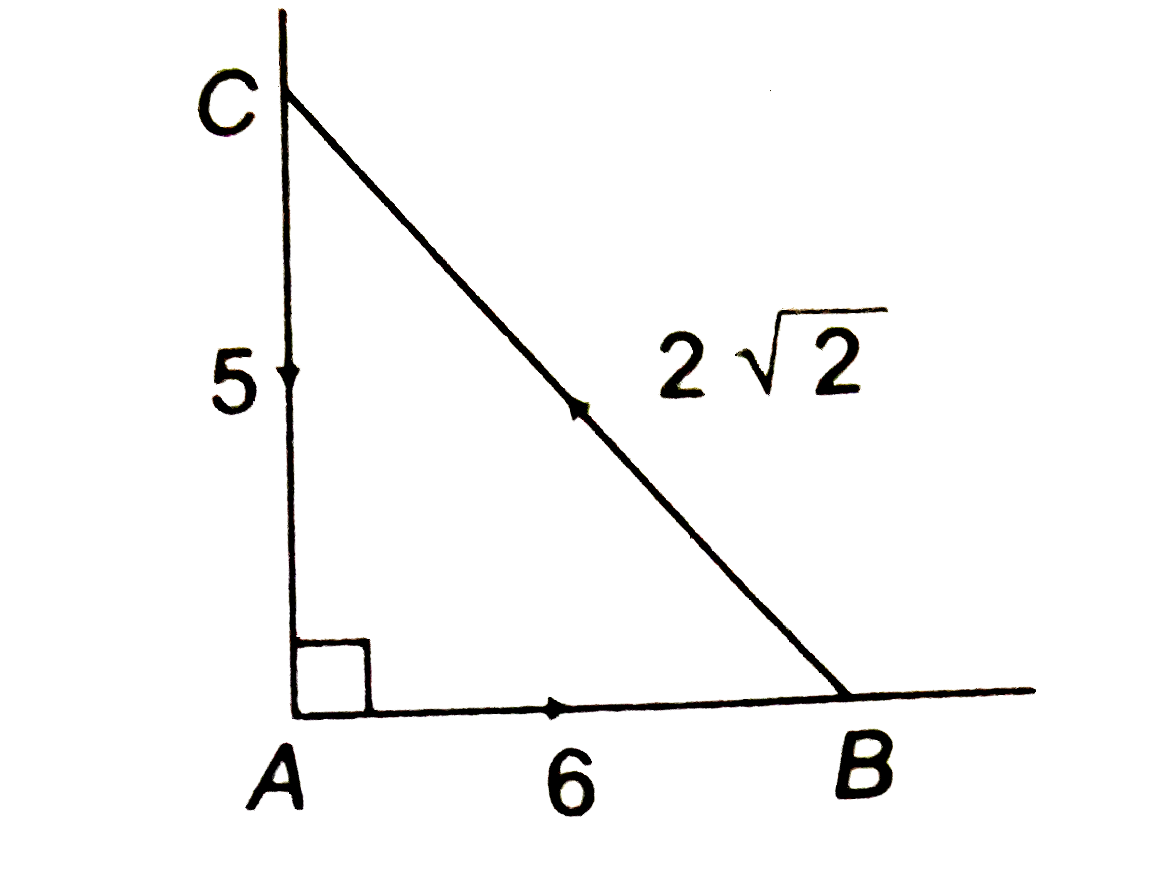
Rsinθ=2√2sinB−5 . . . (ii)
From Eqs. (i) and (ii), we get
R2=36+8cos2B−24√2cosB+8sin2B+25−20√2sinB
=61+8(cos2B+sin2B)−24√2cosB−20√2sinB
∵ ABC is a right angled isosceles triangle.
i.e., ∠B=∠C=45∘
∴R2=61+8(1)−24√21√2−20√2⋅1√2=25
∴R=5.
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 1
7 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 2
17 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
Classify the following measure as scalar and vector: 10Kg
View SolutionIf a=2ˆi−ˆj+2ˆkandb=−ˆi+ˆj−ˆk, then find a+b. Also, find a unit vector along a+b.
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionABC is triangle,right angled at A.The resultant of the forces acting along vec AB.vec AC with magnitudes (1)/(AB) and respectively is the force along vec AB ,where D is the foot of the perpendicular from A onto BC.The magnitude of the resultant is-
View SolutionForces proportional to AB , BC and 2 CA act along the slides of a triangle ABC in magnitude and direction by
View SolutionThree equla force, each of magnitude P, act along the side AB, BC, and CA of a trangle. Calculate the resultant force.
View SolutionForces proportional to AB, BC & 2 CA act along the sides of triangle ABC in order, their resultant represented in magnitude and direction as:
View SolutionABC is isosceles triangle, right angled at A. The resultant of the forces acting along −−→AB,−−→AC with magnitudes 1AB and 1AC respectively is the force along −−→AD, where D is the foot of the perpendicular from A on BC. The magnitude of the resultant is:
View SolutionABC एक समकोण त्रिभुज है −Q आवेश पर बल के परिमाण की गणना कीजिए।
View SolutionA current carrying loop in the form of a right angle isosceles triangle ABC is placed in a uniform magnetic field acting along AB. If the magnetic force on the arm BC is F, what is the force on the arm AC?
View SolutionDelta ABC , But is a right angle. The forces along the AB and AC whose magnitude respectively (1)/(AB) And (1)/(AC) The resulting force of is is perpendicular to AD, where D is the length of the perpendicular from A to BC. The magnitude of the resultant force is
View SolutionA current carrying closed lop in the form of a right angle isosceles triangle ABC is placed in a uniform magnetic field acting along AB if the magnetic force on the arm BC is vecF the force on the arm AC is
View SolutionABC is isosceles triangle, right angled at A. The resultant of the forces acting along −−→AB,−−→AC with magnitudes 1AB and 1AC respectively is the force along −−→AD, where D is the foot of the perpendicular from A on BC. The magnitude of the resultant is:
View SolutionA current carrying closed loop in the form of a right angle isosceles triangle ABC is placed in a uniform magnetic field acting along AB. If the magnetic force on the arm BC is →F, the force on the arm AC is
View SolutionForces proportional to AB, BC & 2 CA act along the sides of triangle ABC in order, their resultant represented in magnitude and direction as:
View SolutionForces proportional to AB, BC and 2CA act along the sides of triangle ABC in order, their resultants represented in magnitude and direction is
View Solution
