R1 on Z defined by (a,b)∈R1 iff |a−b|≤7,R2 on Q defined by (a,b)∈R2 iff ab=4andR3 on R defined by (a,b)∈R3 iff a2−4ab+3ab2=0
Relation R2 isSolution in Punjabi
reflexive
symmetric
transitive
equivalence
The correct Answer is:B
We have (a, b) ∈R2 iff ab = 4, where a, b ∈ Q
Reflexivity 5∈Q and (5)(5) = 25≠4
therefore (5, 5) cancelin R_(2)
The relation R2 is not reflexive.
Symmetry
(a, b) ∈R2
⇒ab=4⇒ba=4
⇒(b,a)∈R2
∴ The relation R2 is symmetric.
Transitivity We have (8,12),(12,8)∈R2 because
8(12)=4and(12)(8)=4
Also, 8(8) = 64 ≠ 4
therefore (8, 8) cancelin R_(2)
∴ The relation R2 is not transitive.
Topper's Solved these Questions
- SETS, RELATIONS AND FUNCTIONS Book:ARIHANT MATHSChapter:SETS, RELATIONS AND FUNCTIONS Exercise:Exercise (Single Integer Answer Type Questions)
Explore 5 Videos
- SETS, RELATIONS AND FUNCTIONS Book:ARIHANT MATHSChapter:SETS, RELATIONS AND FUNCTIONS Exercise:Exercise (Matching Type Questions)
Explore 2 Videos
- SETS, RELATIONS AND FUNCTIONS Book:ARIHANT MATHSChapter:SETS, RELATIONS AND FUNCTIONS Exercise:Exercise (More Than One Correct Option Type Questions)
Explore 3 Videos
- SEQUENCES AND SERIESBook:ARIHANT MATHSChapter:SEQUENCES AND SERIESExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 38 Videos
- THE STRAIGHT LINESBook:ARIHANT MATHSChapter:THE STRAIGHT LINESExercise:The Straight Lines Exercise 8 : (Questions Asked in Previous 13 years Exams)
Explore 1 Video
Similar Questions
In a group of 45 students, 22 can speak Hindi only and 12 can speak English only. If (2λ+1) student can speak both Hindi and English, the value of λ is
View SolutionThe functions defined have domain R
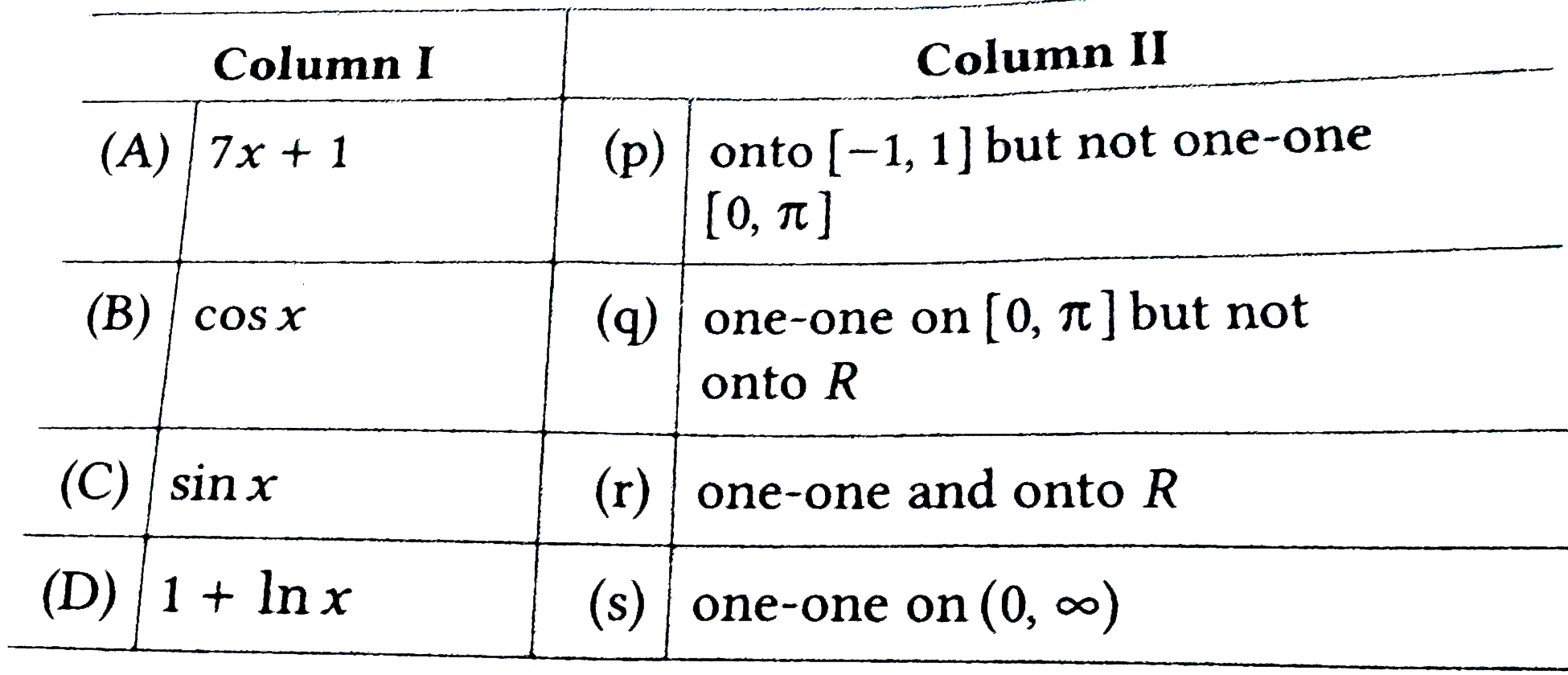
View SolutionLet L be the set of all straight lines in the Euclidean plane. Two lines l1 and l2 are said to be related by the relation R if l1 is parallel to l2. Then, relation R is not
View SolutionLet a,b,c be in A.P. and |a|<1,|b|<1|c|<1.ifx=1+a+a2+....to ∞,y=1+b+b2+....to ∞and,z=1+c+c2+...to ∞, then x,y,z are in
View SolutionSolve the following system of inequalities graphically
x+5y≤25,2x+2y≥2,2x−2y≤0,x≥0,y≥0
View SolutionFor the relation R1 defined on R by the rule (a,b)∈R1:1+ab>0. Prove that: for(a,b)∈R1and(b,c)∈R1then(a,c)∈R1 is not true for all a,b,c∈R
View SolutionTest whether, R3 on R defined by (a, b)∈R3⇔a2−4 ab+3b2=0 .
View SolutionTwo resistors of resistances R_(1)=100 pm 3 ohm and R_(2)=200 pm 4 ohm are connected (a) in series, (b) in parallel. Find the equivalent resistance of the (a) series combination, (b) parallel combination. Use for (a) the relation R=R_(1)+R_(2) and for (b) 1/(R')=1/R_(1)+1/R_(2) and (Delta R')/R'^(2)=(Delta R_(1))/R_(1)^(2)+(Delta R_(2))/R_(2)^(2)
View SolutionProve that r1r2+r2r3+r3r1=14(a+b+c)2
View SolutionLet R1 be a relation defined by
R1={(a,b)∣a>b,a,b∈R}. Then R1 , is
View SolutionProve that 4(r1r2+r2r3+r3r1)=(a+b+c)2
View SolutionProve the questions
a(rr1+r2r3)=b(rr2+r3r1)=c(rr3+r1r2)
View SolutionLet R1 and R2 be two relation defined as follows : R1={(a,b)∈R2,a2+b2∈Q} and R_(2) = {(a,b) in R^(2) , a^(2) + b^(2) cancel(in)Q) where Q is the set of the rational numbers. Then:
View SolutionTwo resistors of resistances R_(1)=100 pm 3 ohm and R_(2)=200 pm 4 ohm are connected (a) in series, (b) in parallel. Find the equivalent resistance of the (a) series combination, (b) parallel combination. Use for (a) the relation R=R_(1)+R_(2) and for (b) 1/(R')=1/R_(1)+1/R_(2) and (Delta R')/R'^(2)=(Delta R_(1))/R_(1)^(2)+(Delta R_(2))/R_(2)^(2)
View SolutionProve that r1r2+r2r3+r3r1=14(a+b+c)2
View Solutionसमुच्चय A = {a, b, c} में तीन संबंध R1,R2तथाR3 निम्न प्रकार परिभाषित किया गए है |
(i) R1{(a,a),(a,b),(a,c),(b,b),(b,c),(c,a),(c,b),(c,c)}
(ii) R2{(a,b),(b,a),(a,c),(c,a)}
(iii) R3{(a,b),(b,c),(c,a)}
यह ज्ञात करो कि प्रत्येक R1,R2तथाR3 स्वतुल्य, सममित और संक्रामक होगा |
View Solution