In a plane there two families of lines : y=x+r, y=-x+r, where r∈{0,1,2,3,4}. The number of the squares of the diagonal of length 2 formed by these lines is____.Solution in Punjabi
9
16
32⋅.4C2
5C2+.3P2
The correct Answer is:A, C
There are two sets of five parallel lines at equal distance. Clearly, lines like l1,l3,m1andm3 form a square whose diagonal's length is 2.
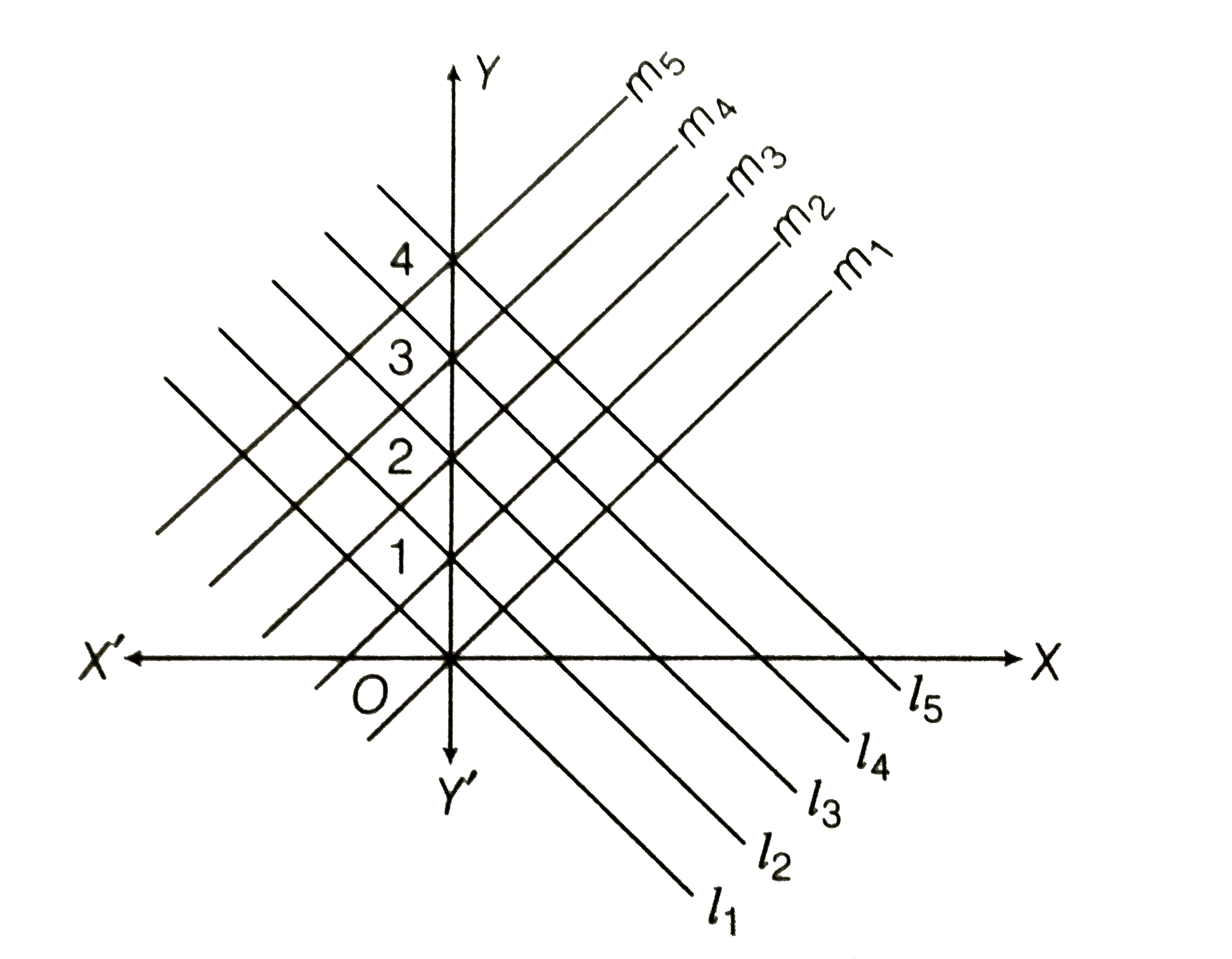
∴Number of required squares=3×3=9=32⋅.4C2
[∵ are (l1,l2),(l2,l4)and(l3,l5) for one set, etc.]
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - JEE Type Solved Examples: Single Matching Type Questions
1 Video
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise For Session 1
11 Videos
- PARABOLABOOK - ARIHANT MATHSCHAPTER - PARABOLAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
35 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
55 Videos
Similar Questions
Solve the quadratic equation 2x^2 + 3x − 6 = 0 using the formula
View SolutionThere are three routes: air, rail and road for going from Chennai to Hyderabad. But from Hyderabad to Vikarabad, there are two routes, rail and road. The number of routes from Chennai to Vikarabad via Hyderabad is:
View SolutionLet A and B be two finite sets such that n(A) =85, n(B) = 65and n(A ∪ B) = 135, find n(A ∩ B)?
View Solutionfind the derivative of sin x cos x
View SolutionIn a plane there are two families of lines y=x+r,y=-x+r, where r in{0,1,2,3,4}. The number of squares of diagonals of length 2 formed by the lines is:
View SolutionA straight line x=y+2 touches the circle 4(x2+y2)=r2 , The value of r is:
View SolutionIf x2+y2=R2(whereR>0)andk=y''(1+y'2)3 then find k in terms of R alone.
View SolutionConsider the fourteen lines in the plane given by y=x+r, y=-x+r, where r∈{0,1,2,3,4,5,6}. The number of squares formed by these lines whose diagonals are of length 2, is
View SolutionIf common tangents of x2+y2=r2 and x216+y29=1 forms a square, then the length of diagonal of the square is
View SolutionConsider the fourteen lines in the plane given by y=x+r,y=−x+r, where r∈{0,1,2,3,4,5,6). The number of squares formed by these lines, whose sides are of length √2, is
View SolutionIf the line x+2y=3 cuts a chord of length r unit with the circle x2+y2=r2 then find r2.
View SolutionIf the length of the chord of the circle , x2+y2=r2(r>0) along the line , y−2x=3 is r, then r2 is equal to
View SolutionThe equation of the plane containing the straight line x2=y3=z4 and perpendicular to the plane containing the straight lines x3=y4=z2andx4=y2=z3 is
px+qy+r=0, then p+q+r is
View SolutionIn a plane there are two families of lines y = x+c, y=-x+c where ce{0, 1, 2, 3, 4} the number of squares of diagonals of length 2 units formed by the lines is
View SolutionIn a plane there are two families of lines y=x+r,y=−x+r, where r∈{0,1,2,3,4}. The number of squares of diagonals of length 2 formed by the lines is:
View SolutionArea of the triangle formed by the lines x2+4xy+y2=0andx−y=4 is____
View Solution
