If both the potential difference and the resistance in a circuit are doubled then,
current remains same
current is doubled
current is halved
current is quadrupled
To solve the problem, we will use Ohm's Law, which states that the current (I) flowing through a conductor between two points is directly proportional to the voltage (V) across the two points and inversely proportional to the resistance (R) of the conductor. The relationship is given by the formula:
V=I×R
1. Identify the Initial Conditions:
- Let the initial potential difference (voltage) be V.
- Let the initial resistance be R.
2. Apply Ohm's Law to Find Initial Current:
- According to Ohm's Law, the initial current I can be expressed as:
I=VR
3. Determine the New Conditions:
- If both the potential difference and the resistance are doubled, we have:
- New potential difference V′=2V
- New resistance R′=2R
4. Calculate the New Current:
- Using Ohm's Law again for the new conditions, the new current I′ can be expressed as:
I′=V′R′=2V2R
5. Simplify the Expression for New Current:
- Simplifying the expression gives:
I′=2V2R=VR
6. Compare the New Current with the Initial Current:
- From the above simplification, we see that:
I′=I
- This shows that the new current I′ is equal to the initial current I.
Conclusion:
Thus, if both the potential difference and the resistance in a circuit are doubled, the current remains the same.
- Let the initial potential difference (voltage) be V.
- Let the initial resistance be R.
- According to Ohm's Law, the initial current I can be expressed as:
I=VR
- If both the potential difference and the resistance are doubled, we have:
- New potential difference V′=2V
- New resistance R′=2R
- Using Ohm's Law again for the new conditions, the new current I′ can be expressed as:
I′=V′R′=2V2R
- Simplifying the expression gives:
I′=2V2R=VR
- From the above simplification, we see that:
I′=I
- This shows that the new current I′ is equal to the initial current I.
Thus, if both the potential difference and the resistance in a circuit are doubled, the current remains the same.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - CENGAGE PHYSICSCHAPTER - CURRENT ELECTRICITYEXERCISE - MANDATORY EXERCISE(EXERCISE SET III)
5 Videos
- CURRENT ELECTRICITYBOOK - CENGAGE PHYSICSCHAPTER - CURRENT ELECTRICITYEXERCISE - MANDATORY EXERCISE(EXERCISE SET IV)
3 Videos
- CURRENT ELECTRICITYBOOK - CENGAGE PHYSICSCHAPTER - CURRENT ELECTRICITYEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
- ATOMIC AND NUCLEAR PHYSICS BOOK - CENGAGE PHYSICSCHAPTER - ATOMIC AND NUCLEAR PHYSICS EXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
8 Videos
- ELECTROMAGNETIC INDUCTION BOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
Similar Questions
(a) Define the unit of resistance (or Define the unit ''ohm'').
(b) What happens to the resistance as the conductor is made thinner?
(c) Keeping the potential difference constant, the resistance of a circuit is doubled. By how much does the current change?
View SolutionKeeping the potential difference constant, the resistance of an electric circuit is doubled. State the change in the reading of an ammeter connected in the circuit.
View Solution
Knowledge Check
Keeping the potential difference constant, the resistance of a circuit is doubled. The current will becomes:
AdoubleBhalfCone-fourthDfour timesIf both the resistance and the inductance in an LR AC series circuit are doubled the new impedance will be
AhalvedBfourfoldCdoubledDquadruptedWhat is the potential difference across resistor in the given circuit ?
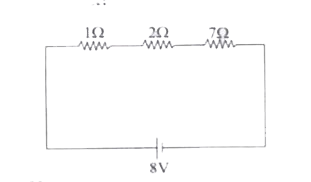 A80VB0VC32VD16V
A80VB0VC32VD16V
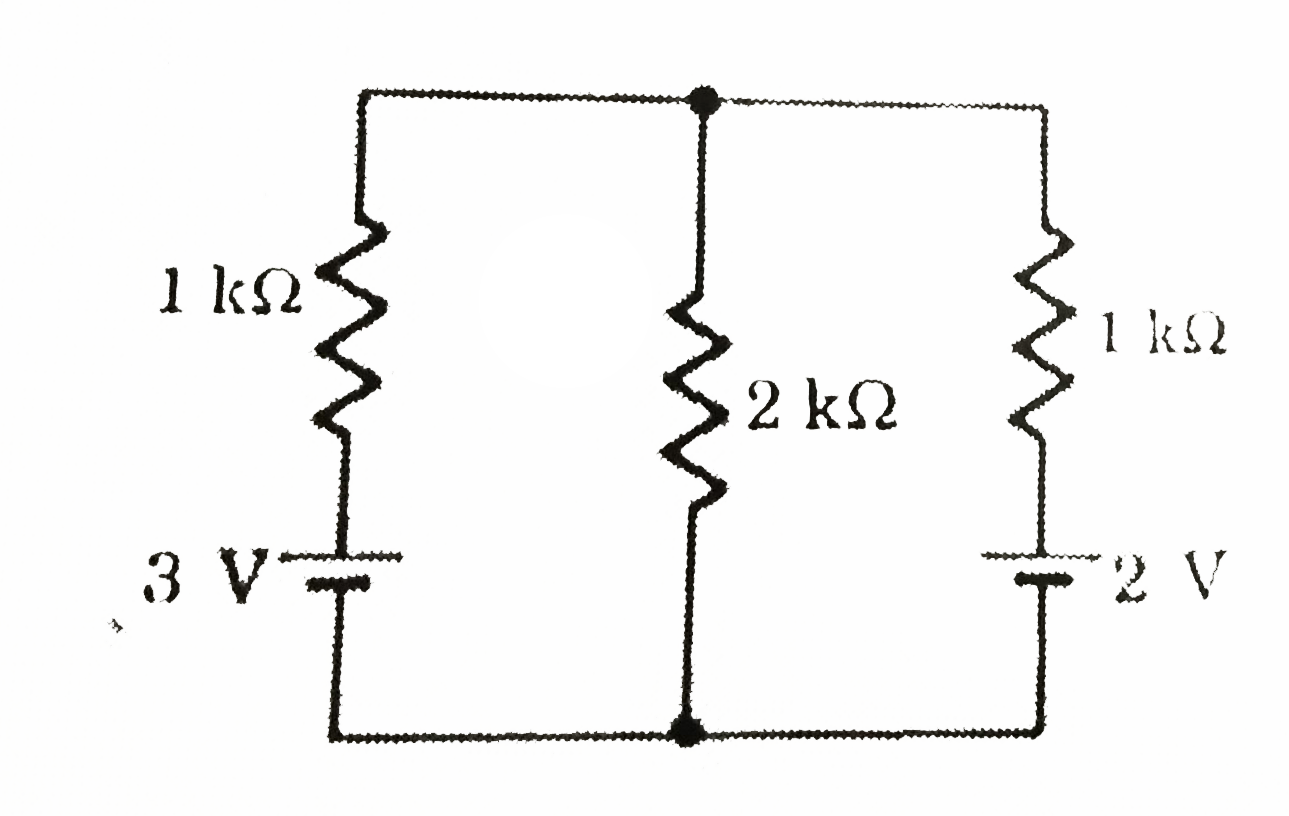
Calculate the potential difference across the 2k Ω resistor in the circuit shown in figure. The internal resistance of the cells are negligible.
View SolutionThe potential differences across the resistance, capacitance and inductance are 80V, 40V and 100V respectively in an L-C-R circuit. The power factor of this circuit is
View SolutionThe potential differences across the resistance, capacitance and inductance are 80V,40V and 100V respectively in an L−C−R circuit. The power factor of this circuit is
View SolutionThe resistance of a metal is given by R=V/I , where V is potential difference and I is current . In a circuit , the potential difference across resistance is V=(8±0.5)V and current in resistance , I=(4±0.2)A. What is the value of resistance with its percentage error?
View SolutionAssertion: An inductance and a resistance are connected in series with an AC circuit. In this circuit the current and the potential difference across the resistance lag behind potential difference across the inductance by an angle π/2.
Reason: In LR circuit voltage leads the current by phase angle which depends on the value of inductance and resistance both.
View Solution