Show that density varies with temperature as ρ2=ρ1(1−γΔT) for small change in temperature.
ρ1=mV1andρ2=mV2
V2=V1(1+γΔT)
∴ρ2=ρ1v1(1+γΔT)
⇒ρ2=ρ1(1+γΔT)
⇒ρ2=ρ1(1+γΔT)
⇒ρ2=(ρ1(1−γ)ΔT)(1+γΔT)(1−γΔT)
⇒ρ=ρ1(1−γΔT)(1−γ2ΔT2)
Usually γ is very small
γ2<<1
1−γ2ΔT2≈1
ρ2=ρ1(1−γΔT)
Topper's Solved these Questions
- HEAT AND TEMPERATURE BOOK - CENGAGE PHYSICSCHAPTER - HEAT AND TEMPERATURE EXERCISE - CONSOLIDATED EXERCISE
12 Videos
- HEAT AND TEMPERATURE BOOK - CENGAGE PHYSICSCHAPTER - HEAT AND TEMPERATURE EXERCISE - CONSOLIDATED EXERCISE (Multiple Choice Questions with one or More than One Correct Answer)
4 Videos
- HEAT AND TEMPERATURE BOOK - CENGAGE PHYSICSCHAPTER - HEAT AND TEMPERATURE EXERCISE - MANDATORY EXERCISE (Exercise Set II)
36 Videos
- HEAT AND MECHANICAL ENERGYBOOK - CENGAGE PHYSICSCHAPTER - HEAT AND MECHANICAL ENERGYEXERCISE - Olympiad and NTSE Level Exercises
7 Videos
- LASERSBOOK - CENGAGE PHYSICSCHAPTER - LASERSEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
1 Video
Similar Questions
How does the density of a solid or a liquid vary with temperature? Show that its variation with temperature is given by ρ'=ρ(1−γ△T), where γ is the coefficient of cubical expansion.
View SolutionHow does the density of a solid or liquid change with temperature ? Show that its variation with temperature is given by ρ=ρ(1−γ△T), where γ is the coefficient of cubical expansion.
View Solution
Knowledge Check
Solids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_200_Q01.png)
Imagine the depth of the block submerged in the liquid ,does not change on increasing temperature thenAγL=2αBγL=3αCγL=(3/2)αDγL=(4/3)αSolids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_199_Q01.png)
Imagine fraction submerged does not change on increasing temperature the relation between γL and αS isAγL=3αSBγL=2αSCγL=4αSDγL=(3/2)αSSolids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_197_Q01.png)
The relation between densities of solid and liquid at temperature T isAρS=2ρLBρS=(1/2)ρLCρS=ρLDρS=(1/4)ρL
A sphere of radius r, density,ρ and specific heat s is heated to temperature θ and then cooled in an enclosure at temperature θ0 . How , the rate of fall of temperature is related with r, ρ and s?
View SolutionA small ball of density 4ρ0 is released from rest just below the surface of a liquid. The density of liquid increases with depth as ρ=ρ0(1+ay) where a=2m−1 is a constant. Find the time period of its oscillation. (Neglect the viscosity effects).
View SolutionThe ednsity (ρ) of an ideal gas varies with temperature T as shown in figure. Then
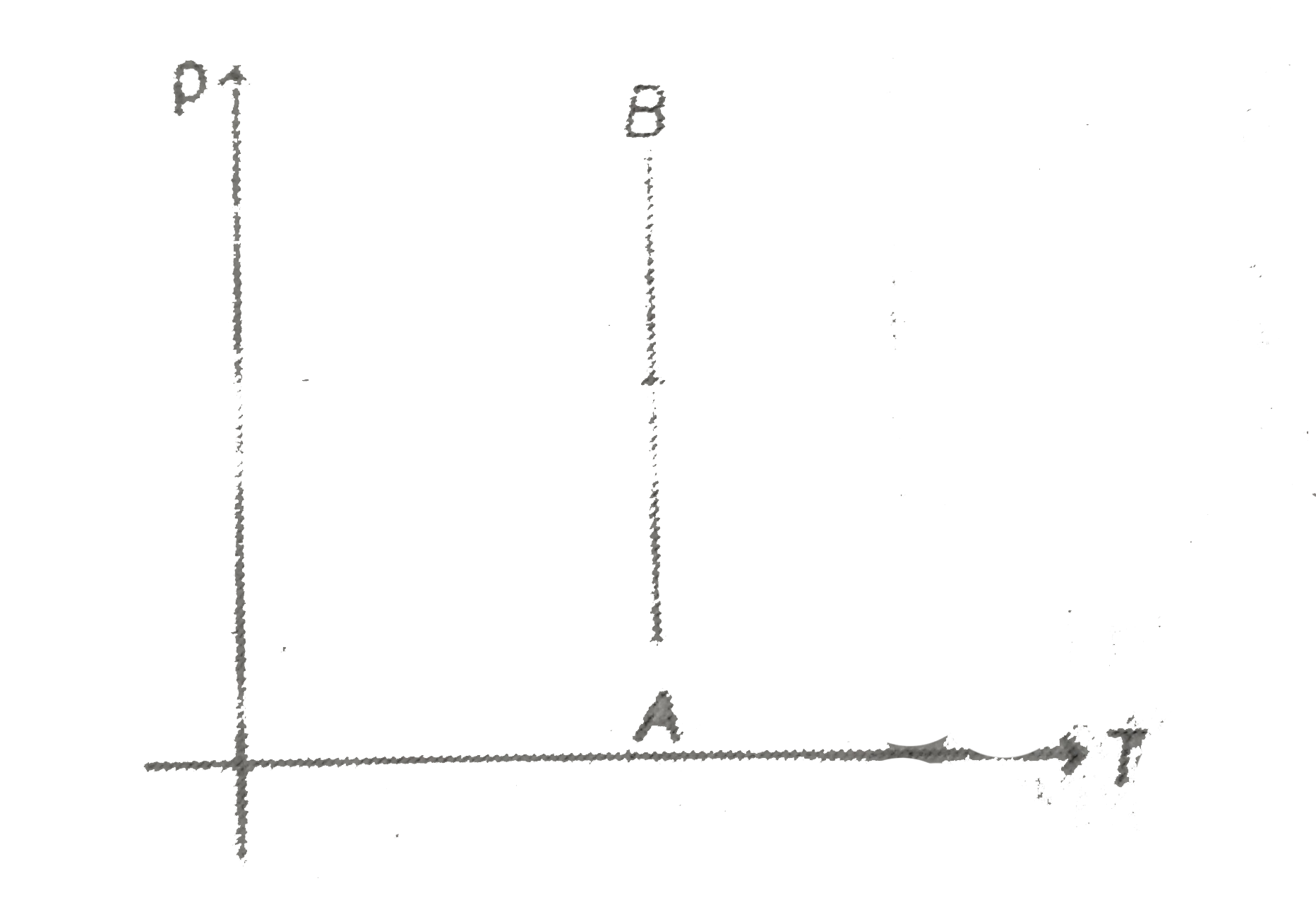
View SolutionA thin uniform tube is bent into a circle of radius r in the vertical plane. Equal volumes of two immiscible liquids, whose densities are ρ1 and ρ2(ρ1>ρ2) fill half the circle. The angle θ between the radius vector passing though the common interface and the vertical is :
View SolutionThe amount of heat supplied to decrease the volume of an ice water mixture by 1cm3 without any change in temperature, is equal to: (ρice=0.9,ρwater=80cal/gm)
View Solution
