In the figure , O is the centre and ∠ACB=20∘∠AOD is equal to
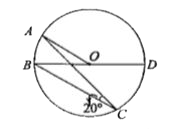
140∘
160∘
120∘
130∘
The correct Answer is:A
Related Playlists
- BOOK - CENGAGECHAPTER - CIRCLES AND CYCLIC QUADRILATERALS EXERCISE - TEST YOURSELF (MULTIPLE CHOICE QUESTIONS)16videos
CIRCLES AND CYCLIC QUADRILATERALS
- BOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - TEST YOURSELF (Multiple Choice Questions) OLYMPIAD AND NTSE LEVEL EXERCISES10videos
BINOMIAL THEOREM
- BOOK - CENGAGECHAPTER - DETERMINANTSEXERCISE - Puzziles and games5videos
DETERMINANTS
Similar Questions
In the figure O is the centre of the circle ∠AOE=150∘,∠DAO=51∘. Calculate the sizes of the angles CEB and OCE.
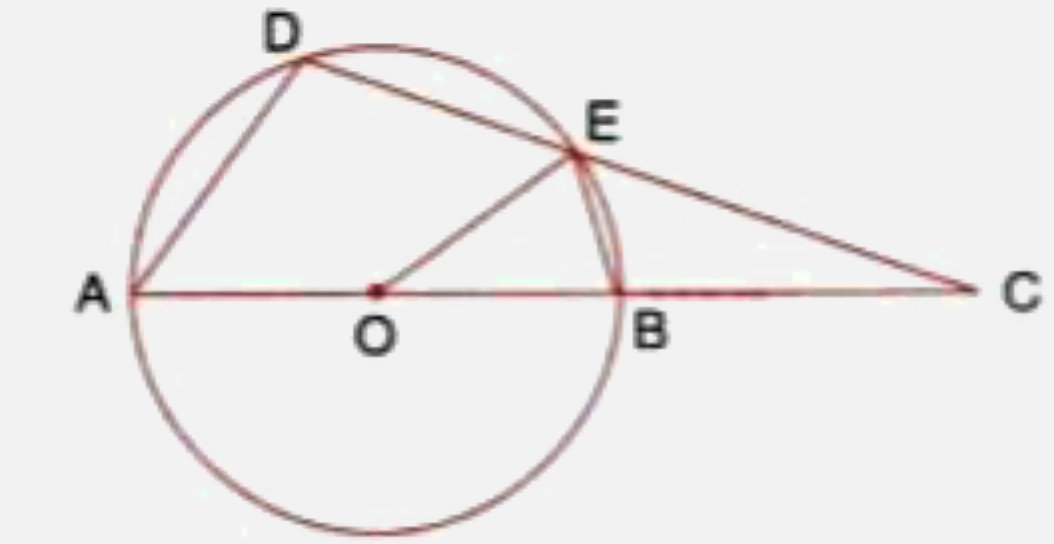 View Solution
View SolutionIn the given figure, O is the centre of the circle, ∠ACB=54∘ and BCF is a straight line. Find x.
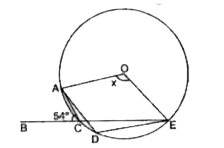 View Solution
View SolutionIn the figure, O is centre of a circle. If ∠BAC=20∘and∠ACB=30∘. Find ∠AOC
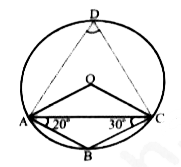 View Solution
View SolutionIn the figure , PQ = PR , O is the centre , ∠RQT=110∘. Then ∠OPQ equals
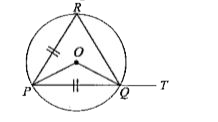 View Solution
View SolutionIn the given figure, PQ is a tangent at a point R of the circle with centre O. If ∠TRQ=30∘, then ∠PRS is equal to
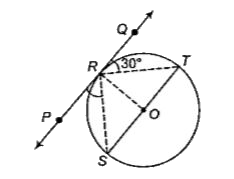 View Solution
View SolutionIn figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to
 View Solution
View SolutionIn figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to
 View Solution
View SolutionIn the given AB=BC=DCand∠AOB=50∘
∠AODView SolutionIn the given figure, O is the center of the circle. ∠DAB=110∘and∠BEC=100∘. What is the value (In degrees) of ∠OCB?
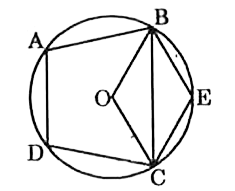 View Solution
View SolutionIn the figure, O is centre of a circle. If ∠BAC=20∘and∠ACB=30∘. Find ∠AOC
 View Solution
View Solution

