Question

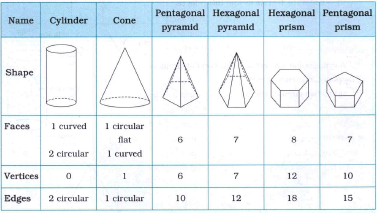
Faces : The flat surface of any solid is called face.
Edges : Line segments where two faces meet is called an edge.
Vertices : Corners of the solid are its vertices.
Find the number of faces, edges and vertices respectively of rectangular prism.

Options

A. 6, 12, 10
B. 6, 8, 10
C. 4, 6, 8
D. 6, 12, 8

Answer

D

Explanation

To find the number of faces, edges, and vertices of a rectangular prism, we can follow these steps:

1. Identify the Faces:
- A rectangular prism has flat surfaces called faces.
- For a rectangular prism, there are 6 faces:
- 2 faces are the top and bottom (the bases).
- 4 faces are the front, back, left, and right sides.
- Total Faces = 6.

2. Identify the Vertices:
- Vertices are the corners where the edges meet.
- A rectangular prism has 8 vertices:
- Each corner of the prism counts as a vertex.
- Total Vertices = 8.

3. Identify the Edges:
- Edges are the line segments where two faces meet.
- A rectangular prism has 12 edges:
- Each face contributes to the edges, and there are 4 edges on the top face, 4 on the bottom face, and 4 vertical edges connecting the top and bottom faces.
- Total Edges = 12.

4. Summarize the Results:
- Faces: 6
- Vertices: 8
- Edges: 12

Final Answer:
The number of faces, edges, and vertices of a rectangular prism is 6 faces, 12 edges, and 8 vertices.

---