A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground . Find the height of the pole, if the angle and by the rope with ground leave is 30∘
The correct Answer is:10 m
To find the height of the pole using the information provided, we can follow these steps:
1. Understanding the Problem:
We have a vertical pole and a rope that is tied from the top of the pole to the ground, forming a right triangle. The length of the rope (hypotenuse) is 20 m, and the angle between the rope and the ground is 30 degrees. We need to find the height of the pole (which is the opposite side of the triangle).
2. Drawing the Diagram:
Let's label the points:
- Let point A be the top of the pole.
- Let point B be the point on the ground directly below point A.
- Let point C be the point where the rope touches the ground.
- The length of the rope (AC) is 20 m.
- The angle ∠CAB is 30 degrees.
- The height of the pole (AB) is what we need to find, and we will denote it as h.
3. Using Trigonometric Ratios:
In triangle ABC, we can use the sine function:
sin(θ)=OppositeHypotenuse
Here, θ = 30 degrees, the opposite side is h (height of the pole), and the hypotenuse is AC (length of the rope).
4. Setting Up the Equation:
So, we can write:
sin(30∘)=h20
We know that:
sin(30∘)=12
Therefore, we can substitute this into our equation:
12=h20
5. Cross-Multiplying:
To solve for h, we can cross-multiply:
1⋅20=2⋅h
This simplifies to:
20=2h
6. Solving for h:
Now, divide both sides by 2:
h=202=10 m
7. Conclusion:
The height of the pole is 10 meters.
We have a vertical pole and a rope that is tied from the top of the pole to the ground, forming a right triangle. The length of the rope (hypotenuse) is 20 m, and the angle between the rope and the ground is 30 degrees. We need to find the height of the pole (which is the opposite side of the triangle).
Let's label the points:
- Let point A be the top of the pole.
- Let point B be the point on the ground directly below point A.
- Let point C be the point where the rope touches the ground.
- The length of the rope (AC) is 20 m.
- The angle ∠CAB is 30 degrees.
- The height of the pole (AB) is what we need to find, and we will denote it as h.
In triangle ABC, we can use the sine function:
sin(θ)=OppositeHypotenuse
Here, θ = 30 degrees, the opposite side is h (height of the pole), and the hypotenuse is AC (length of the rope).
So, we can write:
sin(30∘)=h20
We know that:
sin(30∘)=12
Therefore, we can substitute this into our equation:
12=h20
To solve for h, we can cross-multiply:
1⋅20=2⋅h
This simplifies to:
20=2h
Now, divide both sides by 2:
h=202=10 m
The height of the pole is 10 meters.
Topper's Solved these Questions
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Question Bank - 34
15 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Self Assessment Sheet - 33
10 Videos
- SIMULTANEOUS LINEAR EQUATIONSBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SIMULTANEOUS LINEAR EQUATIONSEXERCISE - SELF ASSESSMENT SHEET
10 Videos
- SQUARE ROOTS AND CUBE ROOTSBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - SQUARE ROOTS AND CUBE ROOTSEXERCISE - SELF ASSESSMENT SHEET-4
10 Videos
Similar Questions
A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30∘.
View SolutionA circus artists is climbing a 20m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole if the angle made by the rope with the ground level is 300.
View Solution
Knowledge Check
A pole of height 14m casts a 10m long shadow on the ground. At the same time, a tower casts a 70m long shadow on the ground. Find the height of the tower.
A50mB78mC90mD98mA vertical stick 15 cm long casts it shodow 10 cm long on the gound. At the same time a flag pole casts a shadow 60 cm long. Find the height of the flag pole :
A40 cmB45 cmC90 cmDnone of these
A circus artist is climbing a 20 m long rope ; which is tightly stretched and tied from the top of a vertical pole to the ground . Find the height of the pole if the angle made by the rope with the ground level is 30∘
View SolutionAnand is watching a circus artist climbing a 20m long rope which is tightly stretched and tied from the top of vertical pole to the ground. Find the height of the pole if the angle made by the rope with the ground level is 30∘.
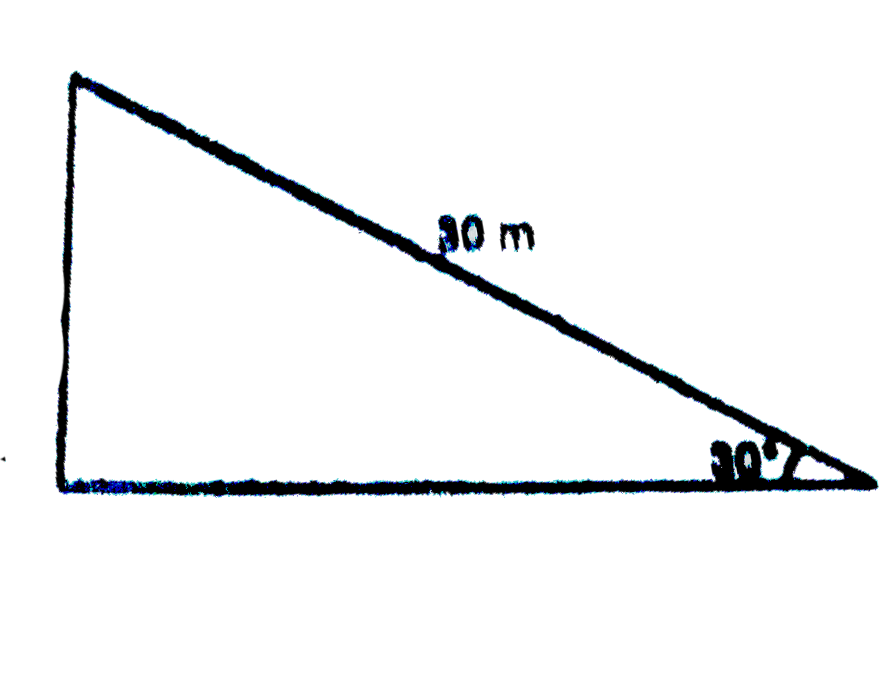
View SolutionA circus artist is climbing a 30 m rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole to the ground. Find the height of the angle made by the rope with the ground level is 30∘), as shown in the figure.
View SolutionA circus artist is climbing from the ground along a rope stretched from the top of a vertical pole and tied at the ground. The height of the pole is 12 m and the angle made by the rope with ground level is 30o . Calculate the distance covered by the artist in climbing to the top of the pole.
View SolutionA circus artist is climbing from the ground along a rope stretched from the top of a vertical pole and tied at the ground. The height of the pole is 10 m and angle made by the top with ground level is 60∘ .
(i) Calculate the distance covered by the artist in climbing to the top of the pole.
(ii) Which mathematical concept is used in this problem ?
(iii) What is its value ?
View SolutionThe angle of elevation of the top of a tower at a point on the ground 20 m from the foot of the tower is 30∘. What is the height of the tower?
View Solution
