ABC is a right-angled triangle, right angled at A and AD is the altitude on BC. If AB : AC= 3:4, what is the ratio BD:DC?
3:4
9:16
2:3
1:2
The correct Answer is:B
Step by step video, text & image solution for ABC is a right-angled triangle, right angled at A and AD is the altitude on BC. If AB : AC= 3:4, what is the ratio BD:DC? by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - TRIANGLES EXERCISE - Self Assessment Sheet
10 Videos
- TRIANGLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - TRIANGLES EXERCISE - Self Assessment Sheet
10 Videos
- TIME AND WORK BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - TIME AND WORK EXERCISE - Self Assessment Sheet - 20
10 Videos
- TRIGONOMETRICAL RATIOS BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - Self Assessment Sheet - 40
1 Videos
Similar Questions
Knowledge Check
△ABC is a right angled at A and AD is the altitude to BC. If AB= 7 cm and AC = 24 cm. Find the ratio of AD is to AM if M is the mid-point of BC.
A25:41B32:41C336/625D625/336△ABC is a right angled at A and AD is the altitude to BC. If AB= 7 cm and AC = 24 cm. Find the ratio of AD is to AM if M is the mid-point of BC.
A25:41B32:41C336625D625336
ΔABC is a right angle triangle (right angled at B), BD is perpendicular to AC. If AC=16cmBC=9cm, find the length of CD.
View Solution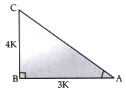
△ABC is a right triangle , right angle at B . Given the ratio of altitude and base tanA=43. Find the ratio of sin A
View Solution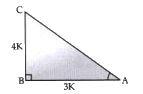
ΔABC is a right triangle , right angle at B . Given the ratio of altitude and base tanA=43 . Find the value of AC
View SolutionABC is a right triangle right angled at C and AC = √3BC . Prove that ∠ABC=60∘ .
View SolutionABC is a triangle right angled at A and AD is perpendicular to BC. If BD = 8 cm and DC =12.5 cm, then what is AD equal to ?
View SolutionABC is a right triangle right-angled at CandAC=√3BC . Prove that ∠ABC=600.
View Solution
