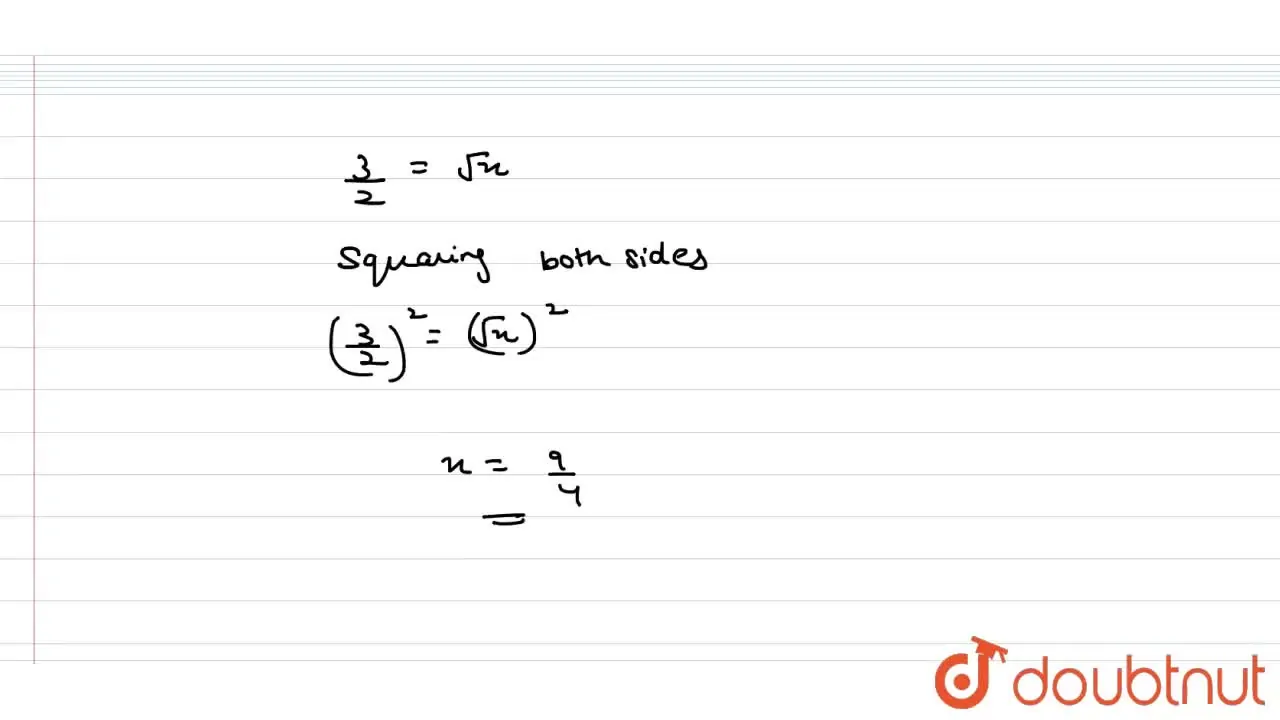
If xx√x=(x√x)x, then x is equal to:
A
32
B
29
C
94
D
49
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for If x^(xsqrt(x))=(xsqrt(x))^(x), then x is equal to: by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - EXPONENTS EXERCISE - SELF ASSESSMENT SHEET10videos
EXPONENTS
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - EXPONENTS EXERCISE - SELF ASSESSMENT SHEET10videos
EXPONENTS
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - DISTANCE, TIME AND SPEED EXERCISE - Unit Test-320videos
DISTANCE, TIME AND SPEED
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - FACTORIZATION OF ALGEBRAIC EXPRESSIONS EXERCISE - SELF ASSESSMENT SHEET-810videos
FACTORIZATION OF ALGEBRAIC EXPRESSIONS
Similar Questions
x√x+2
View SolutionIf xx(√x)=(x√x)x, then the value of x is:
View Solution∫1x√xdx
View Solution∫1x√1−x3dx is equal to
View Solution∫1+x2x√1+x4dx is equal to
View Solution∫dxx√x−1
View Solutionx√1+2x2
View SolutionIf y=√a+x−√a−x√a+x+√a−x,then dydxis equal to (a)ayx√a2−x2 (b) ay√a2−x2 (c)ayx√a2−x2 (d) none of these
View SolutionIf y=sin−1(x√1−x+√x√1−x2),thendydx=
View SolutionIf x√x=(x3/2)x, then find x.
View Solution