The mean, median and mode of given data of scores are 21, 23 and 22 respectively. If 3 is added to each score. What are the new values of mean, median and mode respectively.
21, 23, 24
24, 26, 25
24, 23, 22
23, 21, 24
The correct Answer is:B
To find the new values of mean, median, and mode after adding 3 to each score, we can follow these steps:
Step 1: Understand the given values
The original mean, median, and mode are given as:
- Mean (x̄) = 21
- Median = 23
- Mode = 22
Step 2: Calculate the new mean
When we add a constant (in this case, 3) to each score, the new mean can be calculated by simply adding that constant to the original mean.
New Mean (ȳ) = Original Mean + Constant
yˉ=21+3=24
Step 3: Calculate the new median
The median is the middle value of the ordered data. Since we are adding the same constant to each score, the order of the data does not change. Therefore, we can find the new median by adding the constant to the original median.
New Median = Original Median + Constant
New Median=23+3=26
Step 4: Calculate the new mode
The mode is the value that appears most frequently in the data set. Since we are adding the same constant to each score, the mode will also increase by that constant.
New Mode = Original Mode + Constant
New Mode=22+3=25
Final Results
After adding 3 to each score, the new values are:
- New Mean = 24
- New Median = 26
- New Mode = 25
Summary
Thus, the new values of mean, median, and mode are 24, 26, and 25, respectively.
---
The original mean, median, and mode are given as:
- Mean (x̄) = 21
- Median = 23
- Mode = 22
When we add a constant (in this case, 3) to each score, the new mean can be calculated by simply adding that constant to the original mean.
yˉ=21+3=24
The median is the middle value of the ordered data. Since we are adding the same constant to each score, the order of the data does not change. Therefore, we can find the new median by adding the constant to the original median.
New Median=23+3=26
The mode is the value that appears most frequently in the data set. Since we are adding the same constant to each score, the mode will also increase by that constant.
New Mode=22+3=25
After adding 3 to each score, the new values are:
- New Mean = 24
- New Median = 26
- New Mode = 25
Thus, the new values of mean, median, and mode are 24, 26, and 25, respectively.
Topper's Solved these Questions
- DATA HANDLING Book:S CHAND IIT JEE FOUNDATIONChapter:DATA HANDLING Exercise:Question Bank - 24 (b)
Explore 15 Videos
- CIRCUMFERENCE AND AREA OF A CIRCLE Book:S CHAND IIT JEE FOUNDATIONChapter:CIRCUMFERENCE AND AREA OF A CIRCLE Exercise:SELF ASSESSMENT SHEET - 22
Explore 10 Videos
- DECIMALS Book:S CHAND IIT JEE FOUNDATIONChapter:DECIMALS Exercise:SELF ASSESSMENT SHEET
Explore 10 Videos
Similar Questions
Knowledge Check
The mean mode and median of the given data
A39,15,17,B15,15,39C39,15,15D37,15,15Relation between mean, median and mode -
A¯¯¯X=M=ZB¯¯¯X>M>ZC¯¯¯X<M<ZDNone of theseThe median and mode of a frequency distribution are 26 and 29 respectively. Then , the mean is
A27.5B24.5C28.4D25.8
Find the mean, median and mode of the following data
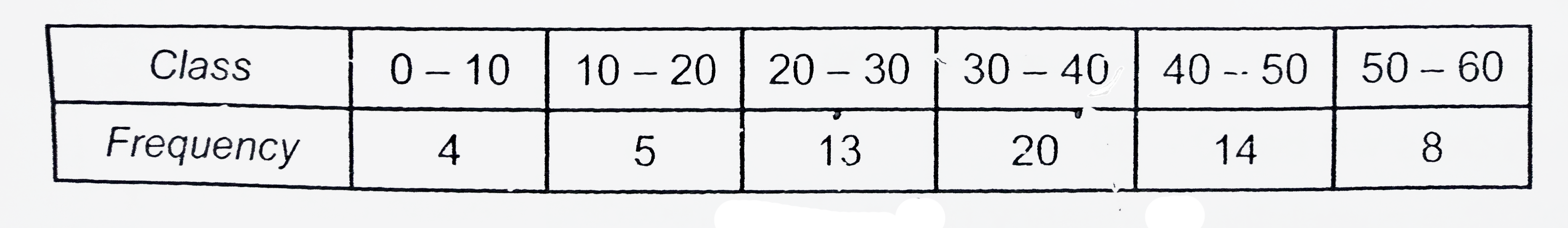
View SolutionFind the mean, median and mode of the following data.
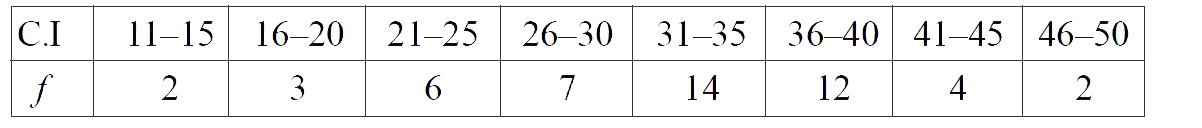
View SolutionFind the mean, median and mode of the following data:
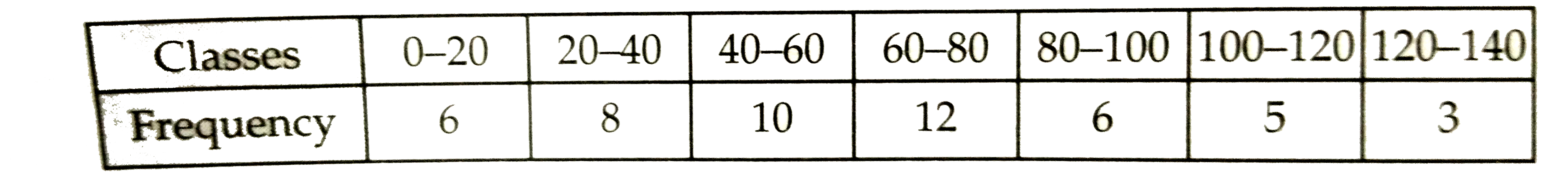
View SolutionThe mean and median of 5 observations are 9 and 8 respectively. If 1 is subtracted from each observation, then the new mean and the new median will respectively be
View SolutionIf the mean and median of a set of numbers are 8.2 and 8.4, respectively, then mode is
View Solution
