Let are circles defined by and . The length of the shortest line segment PQ that is tangent to at P and to at Q is Solution in Bengali
20
15
22
27
The correct Answer is:1
The centers are and
and the radii are and
Also, .
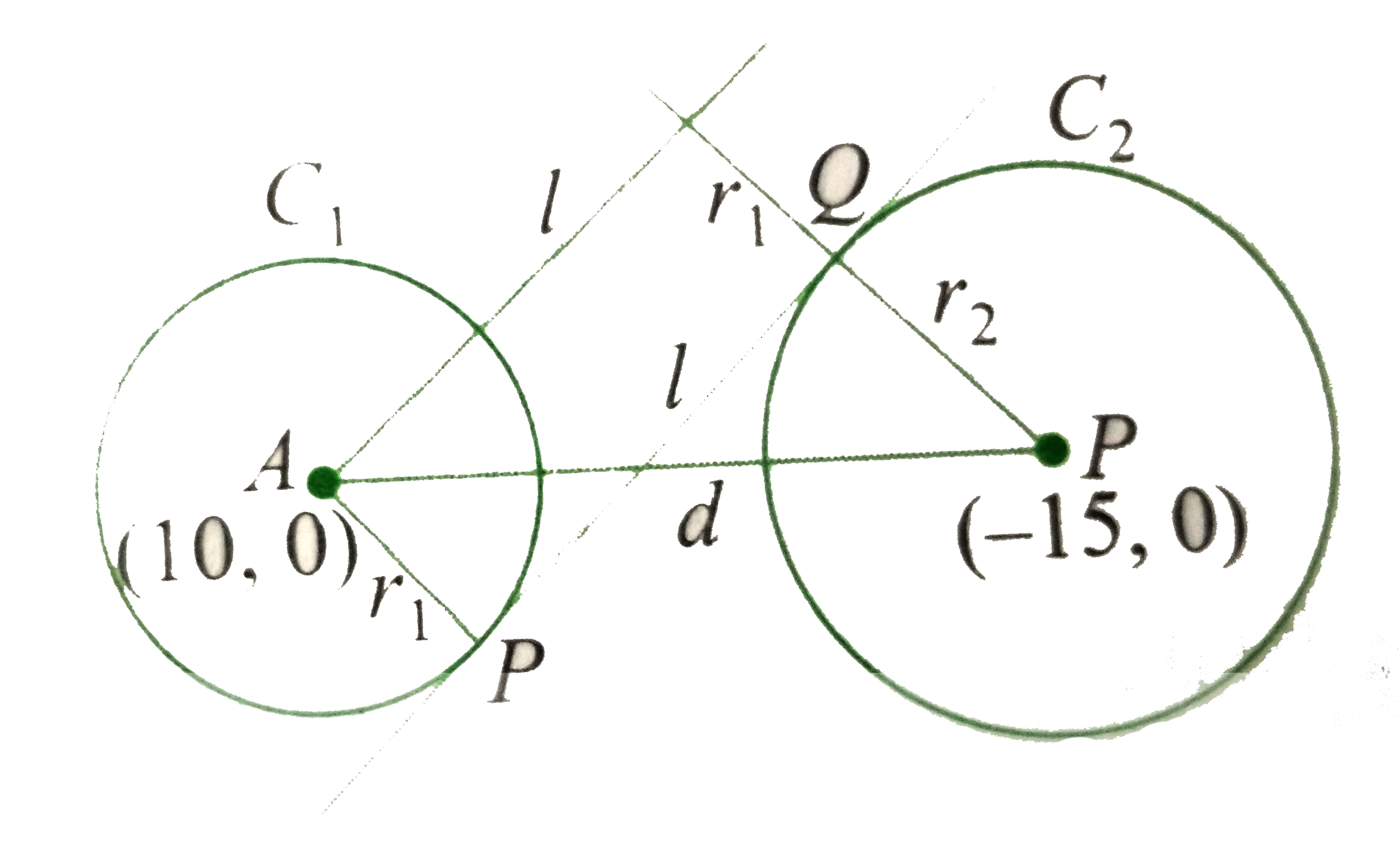
So, the circle are neither intersecting nor touching. Therefore,
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - Multiple Correct Anser Type
29 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - Linked Comprehension Type (For Problem 1-3)
3 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.17
2 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
about to only mathematics
View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
View SolutionThree concentric circles of which the biggest is x2+y2=1, have their radii in A.P If the line y=x+1 cuts all the circles in real and distinct points. The interval in which the common difference of the A.P will lie is:
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is
View SolutionLet C_1 and C_2 be parabolas x^2 = y - 1 and y^2 = x-1 respectively. Let P be any point on C_1 and Q be any point C_2. Let P_1 and Q_1 be the reflection of P and Q, respectively w.r.t the line y = x then prove that P_1 lies on C_2 and Q_1 lies on C_1 and PQ >= [P P_1, Q Q_1]. Hence or otherwise , determine points P_0 and Q_0 on the parabolas C_1 and C_2 respectively such that P_0 Q_0 <= PQ for all pairs of points (P,Q) with P on C_1 and Q on C_2
View SolutionLet C_(1) and C_(2) are circles defined by x^(2)+y^(2)-20x+64=0 and x^(2)+y^(2)+30x+144=0. The length of the shortest line segment PQ that is tangent to C_(1) at P and to C_(2) at Q is
View SolutionConsider the curves C1:y2andC2:x2+y2−6x+1=0
Assertion (A) The common tangents to the curves C1andC2 are othogonal.
Reason ( R ) x-y+1=0 and x+y+1=0 are the common tangents to the curves C1andC2 The correct answer is
View SolutionShow that the circle passing through the origin and cutting the circles x^2+y^2-2a_1x-2b_1y+c_1=0 and x^2+y^2-2a_2x -2b_2y+c_2=0 orthogonally is |(x^2+y^2,x,y),(c_1,a_1,b_1),(c_2,a_2,b_2)|=0
View SolutionTwo circles C1 and C2 intersect at two distinct points PandQ in a line passing through P meets circles C1andC2 at AandB , respectively. Let Y be the midpoint of AB and QY meets circles C1 andC2 at Xa n dZ respectively. Then prove that Y is the midpoint of XZ
View SolutionLet C1andC2 are circles defined by x2+y2−20x+64=0 and x2+y2+30x+144=0. The length of the shortest line segment PQ that is tangent to C1 at P and to C2 at Q is
View SolutionLet C1andC2 are circles defined by x2+y2−20x+64=0 and x2+y2+30x+144=0. The length of the shortest line segment PQ that is tangent to C1 at P and to C2 at Q is
View SolutionShow that the area of the triangle formed by the lines y=m1x+c1,y=m2x+c2 and x=0 is (c1−c2)22|m1−m2|
View SolutionShow that the area of the triangle formed by the lines y=m1x+c1,y=m2x+c2 and x=0 is (c1−c2)22|m1−m2|
View Solutionमाना C1:x2+y2−20x+64=0 तथा C2:x2+y2+30x+144=0 दो वृत है C1 को P पर एवं C2 को Q पर स्पर्श करने वाले सबसे छोटे रेखाखण्ड PQ की लम्बाई है
View Solutionवृतों C1:x2+y2−4x+6y+8=0 C2:x2+y2−10x−6y+14=0 पर विचार कीजिये ।
C1 एवं C2 के संदर्भ में निम्न में से कोनसा / कोनसे कथन सत्य है ?
View Solution
