If the circle x2+y2+2gx+2fy+c=0 bisects the circumference of the circle x2+y2+2g′x+2f′y+c′=0 then prove that 2g′(g−g′)+2f′(f−f′)=c−c'Solution in Bengali
Given circels are
S2≡x2+y2+2gx+2fy+c=0 (1)
S2≡x2+y2+2g'x+2f'y+c'=0 (2)
It is given that circle S1=0 bisects the circumference of the circle S2=0. So, the common chord of circles paseses through the centre of the circle S2=0.
Equation of common chord of the circles is 2x(g−g')+2y(f−f')+c−c'=0.
This chord passes through the centre (−g',−f') of second circle.
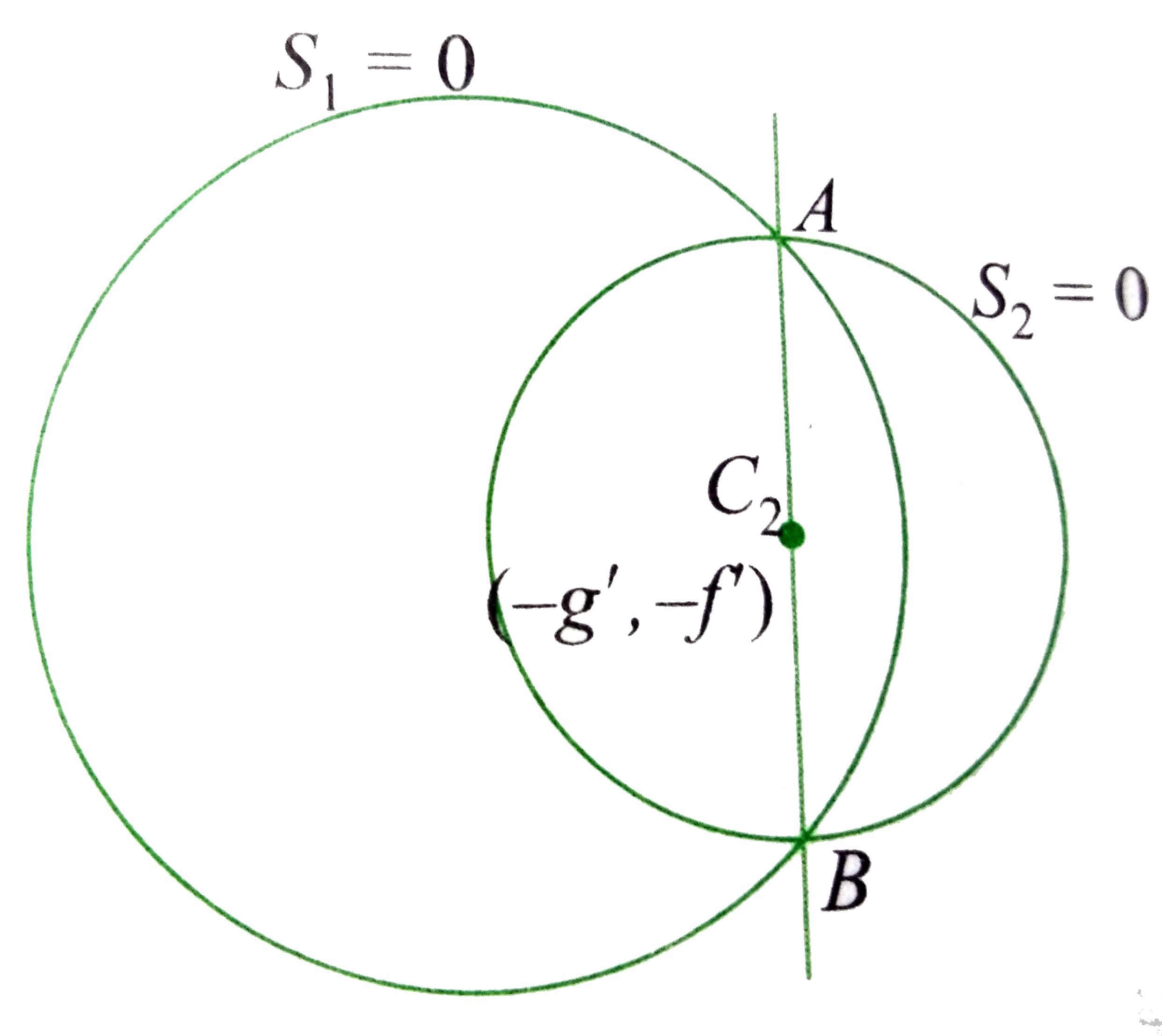
∴−2g'(g−g')−2f'(f−f')+c−c'=0
⇒2g'(g−g')+2f'(f−f')=c−c'
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - Examples
13 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.1
1 Video
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
The line Ax+By+C=0 cuts the circle x2+y2+ax+by+c=0 at PandQ . The line A′x+B′x+C′=0 cuts the circle x2+y2+a′x+b′y+c′=0 at RandS. If P,Q,R, and S are concyclic, then show that |a−a'b−b'c−c'ABCA'B'C'|=0
View SolutionIf a circle whose center is (1,−3) touches the line 3x−4y−5=0 , then find its radius.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is
View SolutionSuppose fandg are functions having second derivative f'' and g'' everywhere. If f(x).g(x)=1 for all xandf′andg' are never zero, then f''(x)f′(x)−g''(x)g′(x)equal (a)−2f′(x)f (b) 2g′(x)g(x) (c)−f′(x)f(x) (d) 2f′(x)f(x)
View SolutionIf a x^2+2h x y+b y^2+2gx+2f y+c=(l x+m y+n)(l prime x+m^(prime)y+n^(prime)) and Delta_1=|(l,l prime,0),(m,m prime,0),(n,n prime,0)|and Delta_2=|(l prime,l,0),(m prime,m,0),(n prime,n,0)|, then the product Delta_1 Delta_2 is
View SolutionIf (ax2+c)y+(a′x2+c′)=0 and x is a rational function of y and ac is negative, then
a. ac′+c′c=0
b. a/a'=c/c'
c. a2+c2=a'2+c'2
d. aa′+cc′=1
View SolutionIf (ax2+bx+c)y+(a′x2+b′x2+c′)=0 and x is a rational function of y, then prove that (ac′−a′c)2=(ab′−a′b)×(bc′−b′c).
View SolutionSuppose fand g are functions having second derivative f'' and g'' everywhere. If f(x).g(x)=1 for all x and f′ and g' are never zero, then f''(x)f′(x)−g''(x)g′(x) is equal (a)−2f′(x)f (b) 2g′(x)g(x) (c)−f′(x)f(x) (d) 2f′(x)f(x)
View SolutionThe line Ax+By+C=0 cuts the circle x2+y2+ax+by+c=0 at PandQ . The line A′x+B′x+C′=0 cuts the circle x2+y2+a′x+b′y+c′=0 at RandS. If P,Q,R, and S are concyclic, then show that |a−a'b−b'c−c'ABCA'B'C'|=0
View SolutionIf the circles x2+y2+2a′x+2b′y+c′=0 and 2x2+2y2+2ax+2by+c=0 intersect othrogonally, then prove that aa′+bb'=c+c′2.
View SolutionIf the circle x2+y2+2gx+2fy+c=0 bisects the circumference of the circle x2+y2+2g′x+2f′y+c′=0 then prove that 2g′(g−g′)+2f′(f−f′)=c−c'
View SolutionStatement 1 : If two circles x2+y2+2gx+2fy=0 and x2+y2+2g′x+2f′y=0 touch each other, then f′g=fg′. Statement 2 : Two circles touch other if the line joining their centers is perpendicular to all possible common tangents.
View SolutionIf the circle x2+y2+2gx+2fy+c=0 bisects the circumference of the circle x2+y2+2g′x+2f′y+c′=0 then prove that 2g′(g−g′)+2f′(f−f′)=c−c'
View SolutionIf f(x)=x+35x2+x−1 and g(x)=2x+3x220+2x−x2 such that f(x) and g(x) are differentiable functions in their domains, then which of the following is/are true (a) 2f′(2)+g′(1)=0 (b) 2f′(2)−g′(1)=0 (c) f′(1)+2g′(2)=0 (d) f′(1)−2g′(2)=0
View SolutionIf (ax2+c)y+(a′x2+c′)=0 and x is a rational function of y and ac is negative, then
a. ac′+c′c=0
b. a/a'=c/c'
c. a2+c2=a'2+c'2
d. aa′+cc′=1
View Solution