Two vertices of a triangle are (1, 3) and (4, 7). The orthocentre lies on the line $x + y = 3$ . The locus of the third vertex is Solution in Bengali

A

$x^{2} - 2 x y + 2 y^{2} - 3 x - 4 y + 36 = 0$

B

$2 x^{2} - 4 x y + 3 y^{2} - 4 x - y + 42 = 0$

C

$3 x^{2} + x y - 4 y^{2} - 2 x + 24 y - 40 = 0$

D

$x^{2} - 4 x y + 3 y^{2} - 2 x - y + 40 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

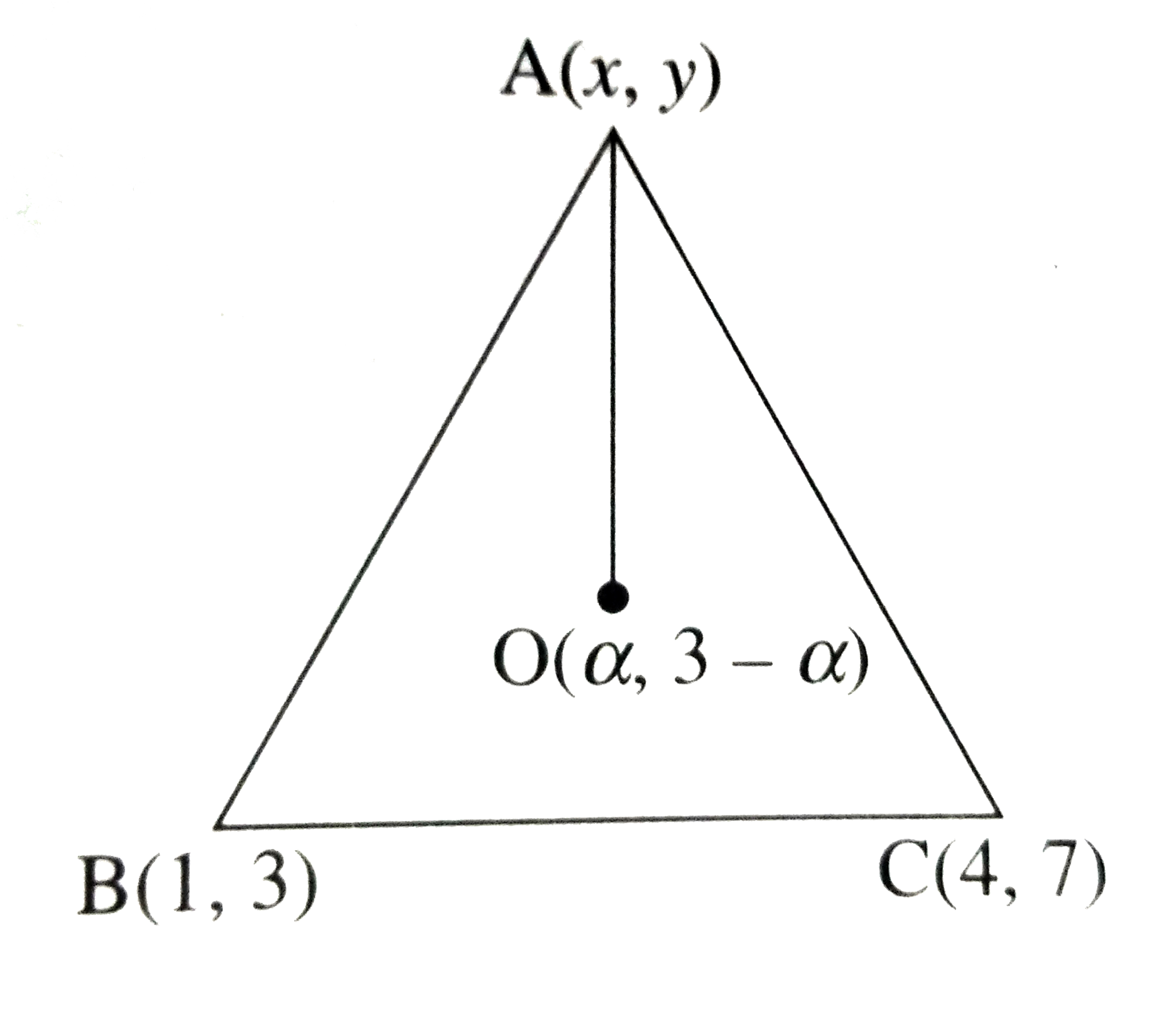
Orthocenter lies on the line $x + y = 2$
Let orthocenter be $O (α, 3 - α)$
$B O ⊥ A C$
$\Rightarrow \frac{y - 7}{x - 4} \frac{α}{1 - α} = - 1$
$\Rightarrow \frac{α - 1}{α} = \frac{y - 7}{x - 4}$
$\Rightarrow 1 - \frac{1}{α} = \frac{y - 7}{x - 4}$
$\Rightarrow \frac{1}{α} = 1 - \frac{y - 7}{x - 4}$
$\Rightarrow α = \frac{x - 4}{x - y + 3}$
Also, $A O ⊥ B C$
$\Rightarrow \frac{y - 3 + α}{x - α} \times \frac{4}{3} = - 1$
$\Rightarrow 4 y = 12 + 4 α = - 3 x + 3 α$
$\Rightarrow α = - 3 x - 4 y + 12$
$∴$ The locus is $\frac{x - 4}{x - y + 3} = - 3 x - 4 y + 12$

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-COORDINATE SYSTEM-Multiple Correct Answers Type

Home
Ask Doubt
Profile