The locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
Then the length of the arc described by the locus of P(z) isSolution in Bengali
10√2π
15π√2
5π√2
5√2π
The correct Answer is:B
(b) 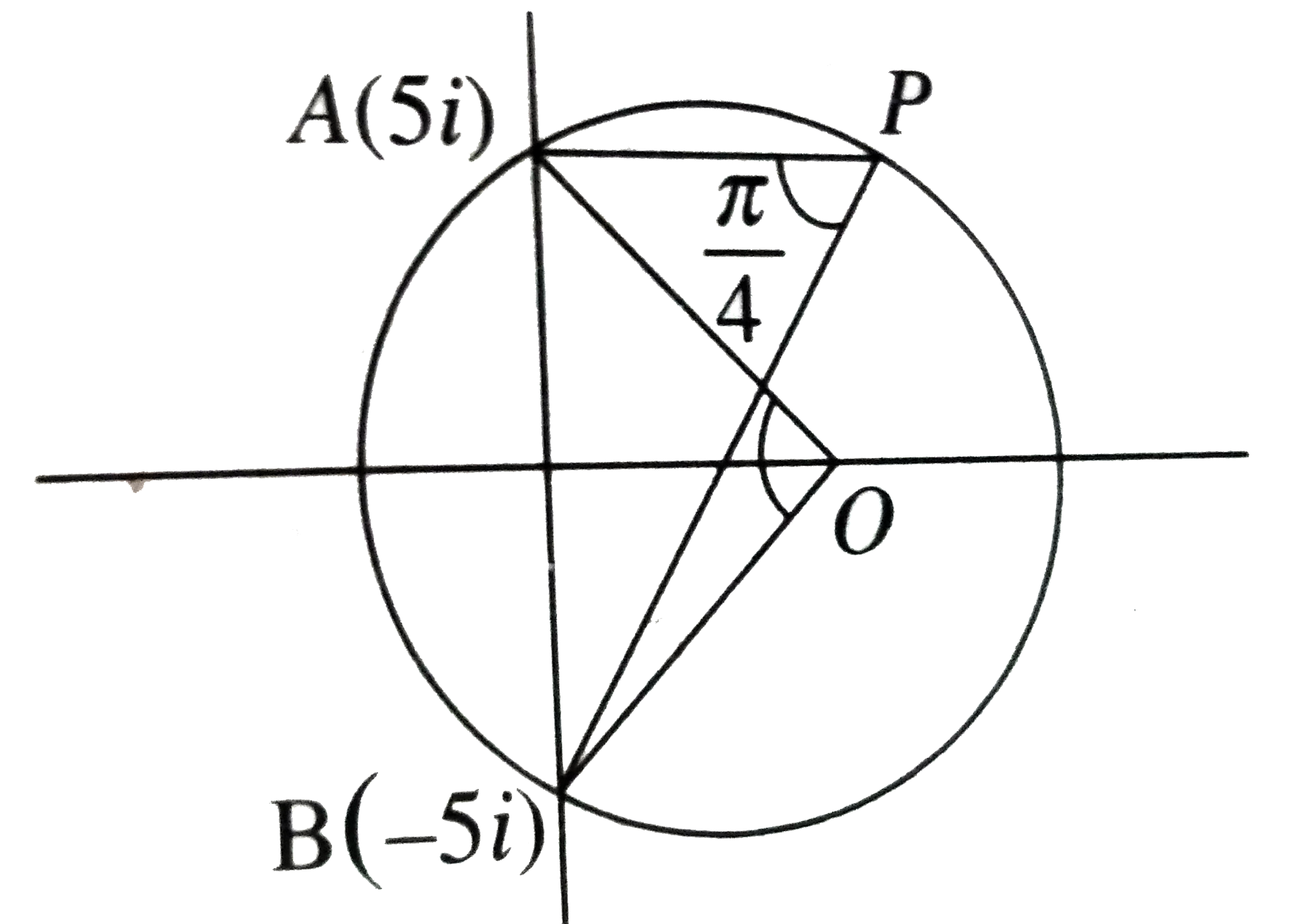
Given ∠APB=π/4⇒∠AOB=π/2
Let OA=OB=R= radius of the circle
⇒2R2=102⇒R=5√2
Length of the arc =5√2×3π2
Topper's Solved these Questions
- COMPLEX NUMBERSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - ILLUSTRATION
110 Videos
- COMPLEX NUMBERSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - SLOVED EXAMPLES
15 Videos
- COMPLEX NUMBERSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - Matching Column
1 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CONIC SECTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - CONIC SECTIONSEXERCISE - All Questions
102 Videos
Similar Questions
Evaluate :
(i) i135
(ii) i−47
(iii) (−√−1)4n+3,n∈N
(iv) √−25+3√−4+2√−9
View Solutionifωandω2 are the nonreal cube roots of unity and [1/(a+ω)]+[1/(b+ω)]+[1/(c+ω)]=2ω2 and [1/(a+ω)2]+[1/(b+ω)2]+[1/(c+ω)2]=2ω , then find the value of [1/(a+1)]+[1/(b+1)]+[1/(c+1)].
View SolutionEvaluate :
i−47
View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is
View SolutionGiven that A(1,1) and B(2,−3) are two points and D is a point on AB produced such that AD=3AB. Find the coordinates of D.
View SolutionIf |z−5i|=∣z+5i , then the locus of z.
View SolutionIf arg((z-3+4i)/(z+2-5i))=(5 pi)/(6), then locus of z represents .........
View SolutionThe locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
Then the length of the arc described by the locus of P(z) is
View SolutionThe locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
Total number of integral points inside the region bounded by the locus of P(z) and imaginery axis on the argand plane is
View SolutionThe locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
Area of the region bounded by the locus of a complex number Z satisfying arg(z+5iz−5i)=±π4
View SolutionThe point P represnets a complex number z in the Argand plane. If the amplitude of z is π4, determine the locus of P.
View SolutionIf Z=x+iy and if the point P in the Argand plane represent Z, then describe geometrically the locus of z satisfying the equation.
lmg z2=4
View SolutionLet the locus of any point P(z) in the argand plane is arg(z−5iz+5i)=π4. If O is the origin, then the value of max.(OP)+min.(OP)2 is
View SolutionThe locus of any point P (z) on argand plane is arg(z+5iz−5i)=π/4, then
Area of the region bounded by the locus of a complex number Z satisfying (z+5iz−5i)=±π4
View SolutionThe locus of z such that arg[(1−2i)z−2+5i]=π4 is a
View SolutionIf z=x+iy and if the point p in the argand plane represents z ,then the locus of z satisfying Im(z2)=4
View Solution
