The vertices of a parallelogram ABCD are A(3,1),B(13,6),C(13,21), and D(3,16). If a line passing through the origin divides the parallelogram into two congruent parts, then the slope of the line is (a) 1112 (b) 118 (c) 258 (d) 138Solution in Bengali
11/12
11/8
25/8
13/8
The correct Answer is:B
Slope of line thorugh origin is,
m=21−x13=x+13
or 63−3x=13x+13
or 16x=50
or x=258
Hence, m=(258+1)×13
=3324=118
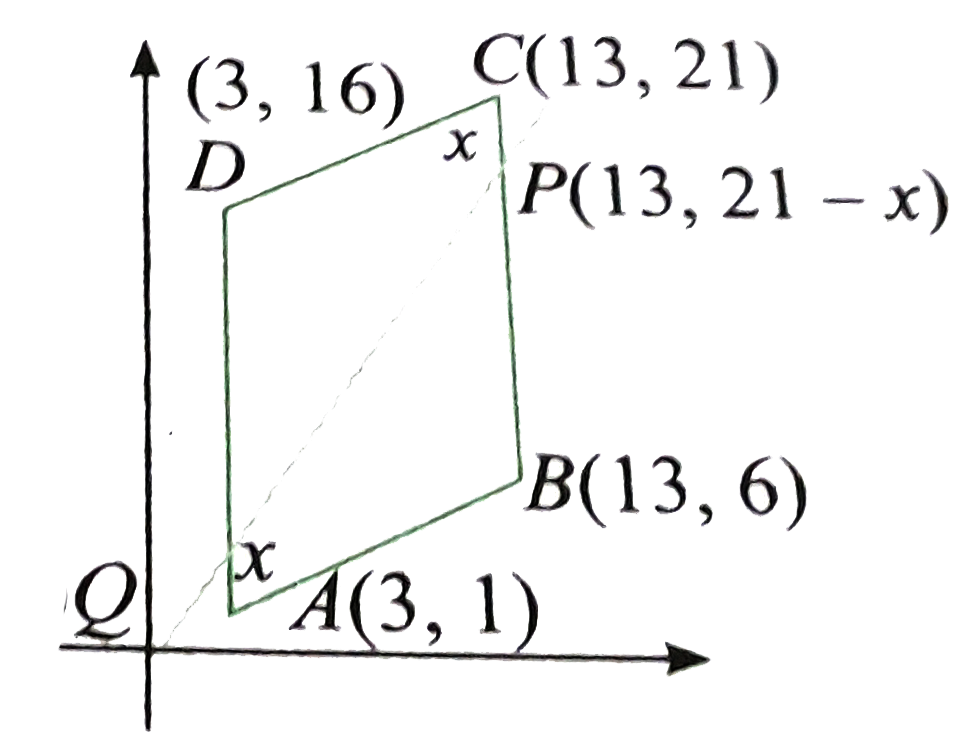
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Multiple correct
13 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Linked
10 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.6
9 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
If (-6,-4), (3,5),(-2,1) are the vertices of a parallelogram, then remaining vertex cannot be
View SolutionFor points P=(x1,y1)and Q=(x2,y2) of the co-ordinate plane a new distance d(P,Q)=|x1−x2|+|y1−y2| is defined .Let O(0,0)and A(3,2). The set of points in first quadrant which are equidistant from O and A is
View SolutionFind the locus of a point whose distance from (a, 0) is equal to its distance from the y-axis.
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionThe vertices of a parallelogram ABCD are A(3,1),B(13,6),C(13,21), and D(3,16). If a line passing through the origin divides the parallelogram into two congruent parts, then the slope of the line is 1112 (b) 118 (c) 258 (d) 138
View Solutiona+b=8,c-d=6,a+c=13,b+d=8 then a,b,c,dis:
View SolutionR is a relation from {11, 12, 13} to {8, 10, 12} defined by y=x−3 . Then, R−1 is (a) {(8, 11), (10, 13)} (b) {(11, 8), (13, 10)} (c) {(10, 13), (8, 11), (8, 10)} (d) none of these
View SolutionIn Fig. 21, ABCD is a parallelogram, DL⊥AB. If AB=20 cm , AD=13 cm and area of the parallelogram is 100 cm2 find AL.
View SolutionIf A={3,5,7,9,11},B={7,9,11,13},C={11,13,15}andD={15,17}, find
A∩C∩D
View SolutionIf A={3,5,7,9,11},B={7,9,11,13},C={11,13,15}andD={15,17}, find
A∩C∩D
View SolutionIf A={3,5,7,9,11},B={7,9,11,13},C={11,13,15}andD={15,17}, find
A∩C∩D
View SolutionIf A={3,5,7,9,11},B={7,9,11,13},C={11,13,15}andD={15,17}, find
A∩C∩D
View SolutionThe vertices of a parallelogram ABCD are A(3,1),B(13,6),C(13,21), and D(3,16). If a line passing through the origin divides the parallelogram into two congruent parts, then the slope of the line is (a) 1112 (b) 118 (c) 258 (d) 138
View SolutionThe vertices of a parallelogram ABCD are A(3,1),B(13,6),C(13,21), and D(3,16). If a line passing through the origin divides the parallelogram into two congruent parts, then the slope of the line is (a) 1112 (b) 118 (c) 258 (d) 138
View SolutionR is a relation from {11, 12, 13} to {8, 10, 12} defined by y=x−3 . Then, R−1 is (a) {(8, 11), (10, 13)} (b) {(11, 8), (13, 10)} (c) {(10, 13), (8, 11), (8, 10)} (d) none of these
View Solution
