The equation of an altitude of an equilateral triangle is √3x+y=2√3 and one of its vertices is (3,√3) then the possible number of triangles isSolution in Bengali
1
2
3
4
The correct Answer is:D

Let the triangle be ABC with C≡(3,√3) and the altitude drawn through the vertex (meeting BC at D) be √3x+y−2√3=0.If Bis(xb,yb),
then we have
xb−3√3=yb−√31=2(3√3+√3−2√3)4=−√3
or xb=0,yb=0
and the coordinates of D are (3/2,√3/2). Let the coordinates of vertex A be (xa,ya). Then,
xa−(3/2)−1/2=ya−(√3/2)√3/2=±3
or(xa,ya)≡(0,2√3) or (3,−√3)
Hence, the remaining vertices are (0,0) and (0,2√3) or (0,0) and (3,−√3). Also, the orthocenter is (1,√3) or (2,0).
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (NUMERICAL VALUE TYPE)
13 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWERS TYPE)
30 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
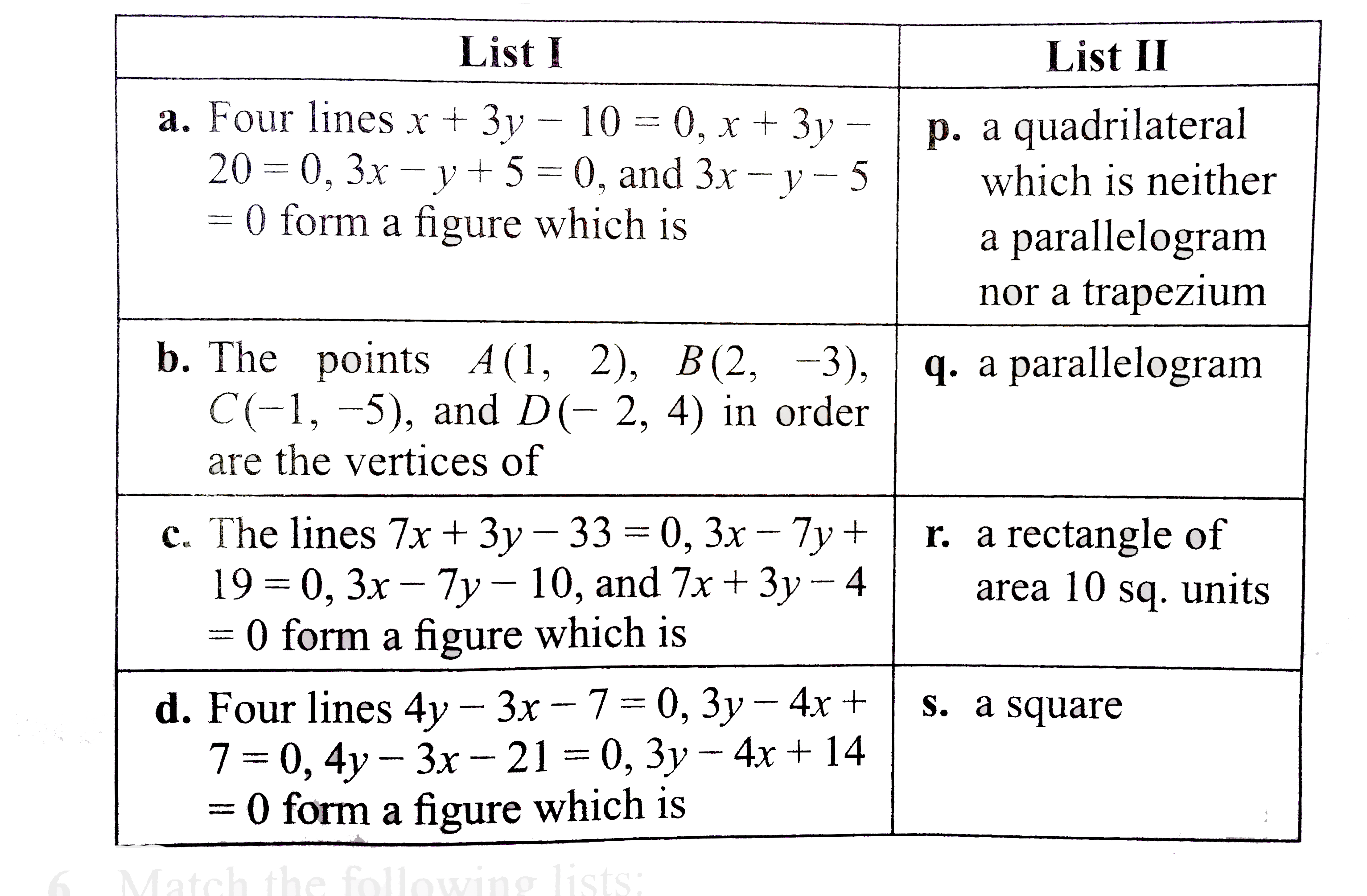
Match the following lists:
View SolutionFind the number of permutations of the set {1,2,3,4} in which no two adjacent positions are filled by consecutive integers ( increasing orders).
View SolutionIf P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionThe equation of an altitude of an equilateral triangle is sqrt(3)x+y=2sqrt(3) and one of its vertices is (3,sqrt(3)) then the possible number of triangles is
View SolutionNumber of equilateral triangle with y=sqrt3(x-1)+2;y=- sqrt3x as two of its sides is
View SolutionAn equilateral triangle, if its altitude is 3.2cm
View SolutionThe equation of two altitudes of an equilateral triangle are √3x−y+8−4√3=0 and √3x+y−12−4√3=0 . The equation of the third altitude is
View SolutionThe altitude of an equilateral triangle is √3cm. What is its perimeter?
View SolutionThe equation of an altitude of an equilateral triangle is √3x+y=2√3 and one of its vertices is (3,√3) then the possible number of triangles is a. 1 b. 2 c. 3 4. 4
View SolutionThe equation of an altitude of an equilateral triangle is √3x+y=2√3 and one of its vertices is (3,√3) then the possible number of triangles is
View SolutionThe equation to the base of an equilateral triangle is (√3+1)x+(√3−1)y+2√3=0 and opposite vertex is A(1,1) then the Area of the trangle is
View SolutionThe equation of an altitude of an equilateral triangle is √3x+y=2√3 and one of its vertices is (3,√3) then
The possible number of triangle (s) is
View SolutionThe equation of an altitude of an equilateral triangle is √3x+y=2√3 and one of its vertices is (3,√3) then
Which of the following can not be the vertex of the triangle
View SolutionThe equation of an altitude of an equilateral triangle is √3x+y=2√3 and one of its vertices is (3,√3) then
Which of the following can be the possible orthocentre of the triangle
View Solution
