Find the values of p for which the equation has
(a) Two distinct negative real roots
(b) Two real roots of opposite sign
(c) Four distinct real roots
(d) No real rootsSolution in Bengali
To examine the roots of the equation , let us first draw the graph of the function.
Also the leading coefficient is 1, when .
Sign scheme of is as follows.
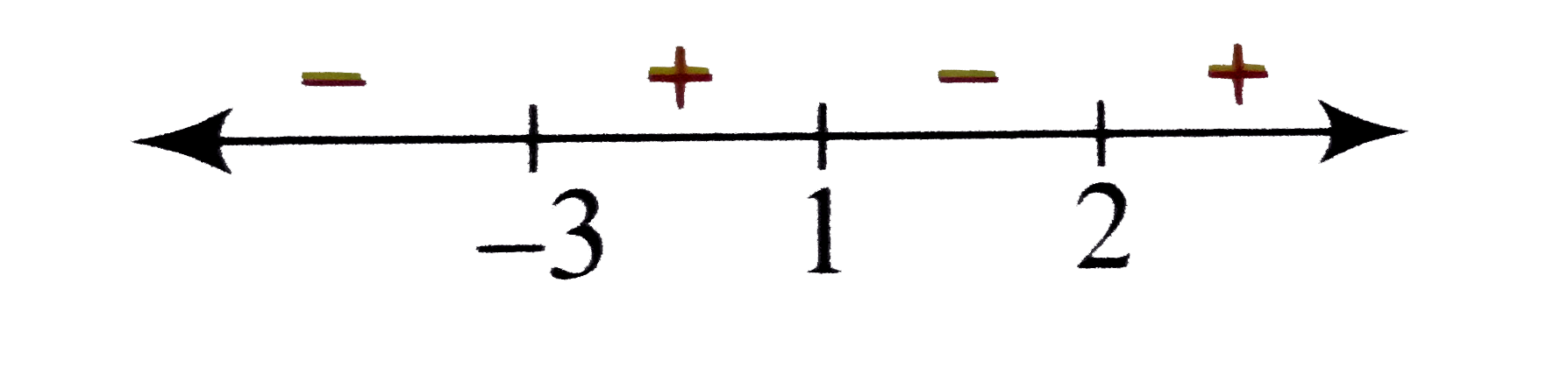
Clearly, from the sign scheme, x = -3 and x = 2 are the points of minima and x = 1 is the point of maxima.
and
Also
So the graph of is as shown in the following figure.

Now to examine the roots of the equation , we draw the straight line y = p and find the intersection of and .
Now for two distinct negative real roots of must cu t between point C and the x-axis. Hence the values of 'p' for two negative roots are (-120,0).
For two real roots of opposite sign, y = p must cut y = f(x) between points H and D. Hence the values of 'p' for two real roots of opposite sign are (-3,5).
For four distinct real roots, y=p must cut four times between points H and F. Hence the values of 'p' are (5,8).
For no real roots, must lie below point C. Hence the values of 'p' are .
Topper's Solved these Questions
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
17 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - ILLUSTRATION
78 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES
34 Videos
Similar Questions
Draw the graph of y=(x−1)(x2−x+1).
View SolutionPlot y=|x|,y+x−2∣,andy=|x|2∣
View SolutionDraw the graph of y=1(1/x).
View SolutionThe equation |x-a|-b|=c has four distinct real roots,then
View SolutionIf the equation ax^(2)+bx+c=0 has distinct real roots and ax^(2)+b{x}+C=0 also has two distinct real roots then
View SolutionIf p,q,rεR and are distinct the equation (x−p)5+(x−q)5+(x−r)5=0 has (A) four imaginary and one real root (B) two imaginary and three real roots (C) all the roots real (D) none of these
View SolutionSuppose a, b, c are real numbers, and each of the equations x2+2ax+b2=0 and x2+2bx+c2=0 has two distinct real roots. Then the equation x2+2cx+a2=0 has - (A) Two distinct positive real roots (B) Two equal roots (C) One positive and one negative root (D) No real roots
View SolutionThe quadratic equation 2x2−√5x+1=0 has
(a) two distinct real roots (b) two equal real roots
(c) no real roots (d) more than 2 real roots
View SolutionFind the values of p for which the equation x4−14x2+24x−3−p=0 has
(a) Two distinct negative real roots
(b) Two real roots of opposite sign
(c) Four distinct real roots
(d) No real roots
View SolutionThe quadratic equation p(x)=0 with real coefficients has purely imaginary roots. Then the equation p(p(x))=0 has A. only purely imaginary roots B. all real roots C. two real and purely imaginary roots D. neither real nor purely imaginary roots
View SolutionThe equation (xx+1)2+(xx−1)2=a(a−1) has
a. Four real roots if a>2
b.Four real roots if a<−1
c. Two real roots if 1<a<2
d . No real roots if a<−1
View SolutionFind the values of p for which the equation x4−14x2+24x−3−p=0 has
(a) Two distinct negative real roots
(b) Two real roots of opposite sign
(c) Four distinct real roots
(d) No real roots
View SolutionConsider function f(x)=x4−14x2+24x−3=p
Column - IColumn -II(A)two negative real rootsPforp>120(B)two real roots of opposite signfor−8≤p≤−5(C)four real rootsfor3<p≤<120(D)no real rootsforp<−8or−5<p<3
View SolutionIf a,b,c are real distinct numbers such that a3+b3+c3=3abc, then the quadratic equation ax2+bx+c=0 has (a) Real roots (b) At least one negative root (c) Both roots are negative (d) Non real roots
View SolutionThe quadratic equation 2x2−√5x+1=0 has
(a) two distinct real roots (b) two equal real roots
(c) no real roots (d) more than 2 real roots
View SolutionIf the two roots of the equation ax^2 + bx + c = 0' are distinct and real then c
View Solution
