Consider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
then the value of c is Solution in Bengali
-3
9/2
8/3
7/4
The correct Answer is:4
As the lines are perpendicular, Coefficient of x2+ Coefficient of y2=0
∴a−2=0
or a=2
Also , abc+2fgh−af2−bg2−ch2=0
∴c=−3
Hence , the given pair of lines is
2x2+3xy−2y2−5x+5y−3=0
Factorizing , we get lines
x+2y−3=0and2x−y+1=0
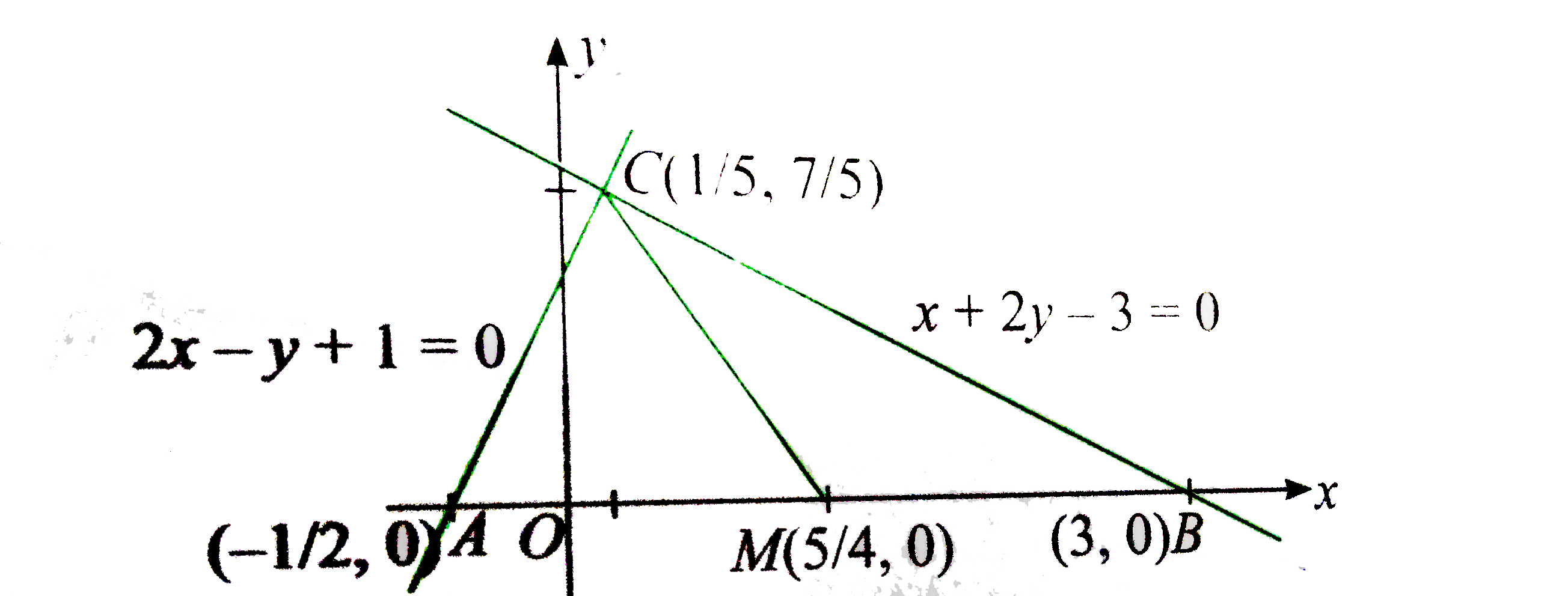
The point of intersection of the lines is C (1/5,7/5).
The points of intersection of the lines with the x- axis are A(3,0) and B (−1/2,0).
The orthocenter of triangle is C (1/5,7/5) and the circumcenter is the midpoint of AB which is M (5/4,0). Therefore,
CM√(54−15)2+4925=74
Topper's Solved these Questions
- PAIR OF STRAIGHT LINESBook:CENGAGE PUBLICATIONChapter:PAIR OF STRAIGHT LINESExercise:Numberical Value Type
Explore 5 Videos
- PAIR OF STRAIGHT LINESBook:CENGAGE PUBLICATIONChapter:PAIR OF STRAIGHT LINESExercise:Multiple Correct Answer Type
Explore 9 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBook:CENGAGE PUBLICATIONChapter:MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSExercise:Comprehension Type
Explore 6 Videos
- PARABOLABook:CENGAGE PUBLICATIONChapter:PARABOLAExercise:Matching Column Type
Explore 1 Video
Similar Questions
The distance between the lines (x+7y)2+4√2(x+7y)−42=0 is_____________
View SolutionThe equation x3+x2y−xy2=y3 represents
View SolutionLet f:R→R,y=f(x),f(0)=0,f'(x)>0andf''(x)>0. Three point A(α,f(α)),B(β,f(β)),C(γ,f(γ))ony=f(x) such that 0<α<β<γ.
Which of the following is false ?
View SolutionAB is a chord of y2=4x such that normals at A and B intersect at C(9,6) and the tengent at A and B at point T, find (CT)2/13
View SolutionConsider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
The value of a is
View SolutionConsider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
The value of c is
View SolutionConsider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
Distance between the orthocenter and the circumcenter of triangle ABC is
View SolutionStatement I . The equation 2x2−3xy−2y2+5x−5y+3=0 represents a pair of perpendicular straight lines.
Statement II A pair of lines given by ax2+2hxy+by2+2gx+2fy+c=0 are perpendicular if a+b=0
View SolutionConsider a pair of perpendicular straight lines 2x2+3xy+by2−11x+13y+c=0 The value fo c is
View SolutionConsider a pair of perpendicular straight lines 2x2+3xy+by−11x+13y+c=0
The value of |b+2c|is
View SolutionConsider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
The value of a is
View SolutionConsider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
The value of c is
View SolutionConsider a pair of perpendicular straight lines ax2+3xy−2y2−5x+5y+c=0.
Distance between the orthocenter and the circumcenter of triangle ABC is
View SolutionThe point of intersection of the perpendiecular lines ax2+3xy−2y2−5x+5y+c=0 is
View SolutionStatement I . The equation 2x2−3xy−2y2+5x−5y+3=0 represents a pair of perpendicular straight lines.
Statement II A pair of lines given by ax2+2hxy+by2+2gx+2fy+c=0 are perpendicular if a+b=0
View SolutionConsider a pair of perpendicular straight lines 2x2+3xy+by2−11x+13y+c=0 The value fo c is
View Solution