Find the area of the region bounded by the curve C : y=tan x ,tangent drawn to C at x=pi/4, and the x-axis.Solution in Bengali

Video Solution

Text Solution

Verified by Experts

The given curve is $y = \tan x (1)$
$When x = π / 4, y = 1$
i.e., co-ordinates of P are $(π / 4, 1)$
$∴ equation of tangent at P is y - 1 = (\sec^{2} \frac{π}{4}) (x - π / 4)$
$or y = 2 x + 1 - π / 2$
The graphs of (1) and (2) are as shown in the figure.

$Tangent (2) meets x-axis at L (\frac{π - 2}{4}, 0)$
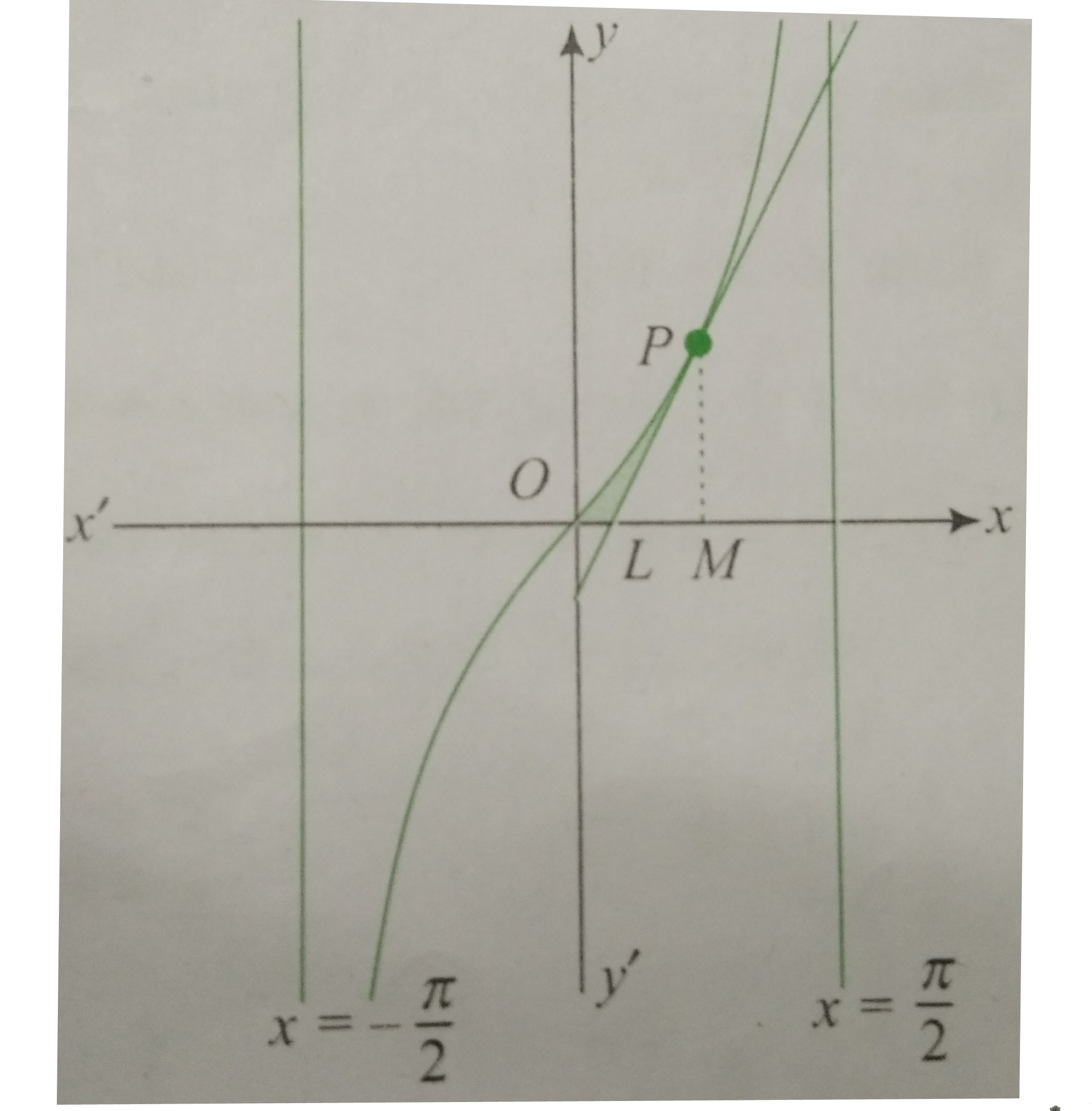
Now, Required area =Shaded area
$= Area O P M O - A r (Δ P L M)$
$= \int_{0}^{π / 4} \tan x d x - \frac{1}{2} (O M - O L) P M$
$= {[\log \sec x]}_{0}^{π / 4} - \frac{1}{2} \times {\frac{π}{2} - \frac{π - 2}{4}} \times 1$
=(1)/(2)[ log 2 -(1)/(2)]sq. units.

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-APPLICATION OF INTEGRALS-All Questions

Home
Ask Doubt
Profile