AB and CD are equal and parallel chords of a circle with centre O. Then AC passes through the centre O. [Agree / Disagree]
The correct Answer is:Disagree
Step by step video, text & image solution for AB and CD are equal and parallel chords of a circle with centre O. Then AC passes through the centre O. [Agree // Disagree] by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - TEST YOUR CONCEPTS ( SHORT ANSWER TYPE QUESTIONS)
15 Videos
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - TEST YOUR CONCEPTS (ESSAY TYPE QUESTIONS)
5 Videos
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Level 3
7 Videos
- FormulaeBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - FormulaeEXERCISE - Level 3
7 Videos
- INDICESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - INDICESEXERCISE - CONCEPT APPLICATION (Level 3)
7 Videos
Similar Questions
Knowledge Check
AB and AC are tangents to the circle with centre O. Then x equals.
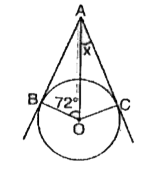 A22∘B18∘C20∘D36∘
A22∘B18∘C20∘D36∘AB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB =10cm , CD =24 cm and the distance between AB and CD is 17cm. Find the radius of the circle.
A13 cmB14 cmC15 cmD16 cmA chord that passes through the centre of a circle is___
ADiameterBSegmentCRadiusDCentre
AB and CD are two parallel chords of a circle whose diameter is AC . Prove that AB=CD
View SolutionAB and CD are diameters of a circle with centre O. If ∠OBD=50∘ then find ∠AOC
View SolutionABandCD are equal chords of a circle whose centre is O, when produced these chords meet at E,Prove that EB=ED.
View SolutionAB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB=10cm, CD=24cm and the distance between AB and CD is 17cm. Find the radius of the circle.
View SolutionIn the given figureAB and CD are two parallel chords of a circle with centre O and radius 5 cm. Also, AB = 8 cm andCD = 6cm. If OP perpendicular to AB andOQ perpendicular to CD,then determine the length of PQ
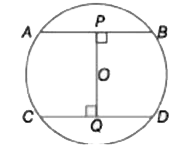
View Solution
