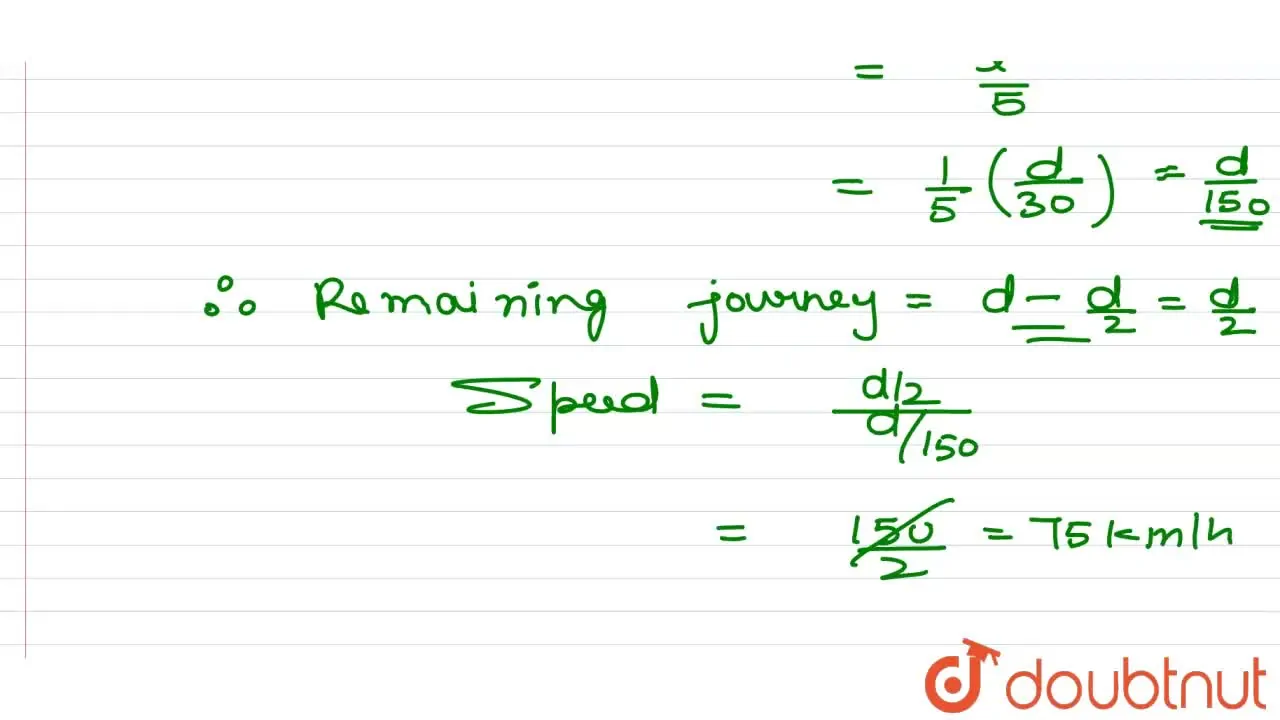
If a person travels in a car at a speed of 30 km/h then he would reach his destination on time . He covers half journey in 4/5 th time. What should be his speed for the remaining part of the journey so that he reaches his destination on time ?
The correct Answer is:75 kmph
Step by step video, text & image solution for If a person travels in a car at a speed of 30 km/h then he would reach his destination on time . He covers half journey in 4/5 th time. What should be his speed for the remaining part of the journey so that he reaches his destination on time ? by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Related Playlists
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TIME AND DISTANCE EXERCISE - Concept Application (Level 1 )30videos
TIME AND DISTANCE
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TIME AND DISTANCE EXERCISE - Concept Application (Level 2 )3videos
TIME AND DISTANCE
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TIME AND DISTANCE EXERCISE - Test Your Concepts ( Short Answer Type Questions)15videos
TIME AND DISTANCE
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - STATISTICS EXERCISE - CONCEPT APPLICATION (LEVEL II)29videos
STATISTICS
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TIME AND WORK PIPES AND CISTERNSEXERCISE - CONCEPT APPLICATION (LEVEL-3)7videos
TIME AND WORK PIPES AND CISTERNS
Similar Questions
Travelling at 60 km/h, a person reaches his destination in a certain time. He covers 60% of his journey in 2/5th of the time. At what speed (in km/h) should he travel to cover the remaining journey so that he reaches the destination right on time?
60 किमी प्रति घंटा की चाल से चलते हुए एक व्यक्ति अपने गंतव्य स्थल पर किसी निश्चित समय में पहुँचता है | वह अपनी 60% यात्रा 2/5 समय में कर लेता है | शेष यात्रा ( किमी/घंटा में ) उसे किस चाल से करनी चाहिए ताकि वह गंतव्य स्थल पर सही समय पर पहुंचे ?View SolutionTravelling at 60 km/h, a person reaches his destination in a certain time. He covers 60% of his journey in 2/5th of the time. At what speed (in km/h) should he travel to cover the remaining journey so that he reaches the destination right on time?
60 किमी प्रति घंटा की चाल से चलते हुए एक व्यक्ति अपने गंतव्य स्थल पर किसी निश्चित समय में पहुँचता है | वह अपनी 60% यात्रा 2/5 समय में कर लेता है | शेष यात्रा ( किमी/घंटा में ) उसे किस चाल से करनी चाहिए ताकि वह गंतव्य स्थल पर सही समय पर पहुंचे ?View SolutionTravelling at 60 km/h, a person reaches his destination in a certain time. He covers 60% of his journey in 2/5 th of the time. At what speed (in km/h) should be travel to cover the remaining journey so that he reaches the destination right on time?
काई व्यक्ति 60 किमी/घंटे की यात्रा कर एक निश्चित समय में गंतव्य पर पहुचता है। वह अपनी 60% यात्रा को 2/5 समय में तय करता है। बाकी यात्रा पूर्ण करने के लिए उसे किस गति (किमी/घंटे)से यात्रा करनी चाहिए ताकि वह अपने गंतव्य पर समय पर पहुच सके?View SolutionRaju has to cover a distance of 240 km in 4 hours. If he covers one-third of the journey in 27th time, what is his speed at the beginning of the journey?
View SolutionLaxman has to cover a distance of 6 km in 45 min if he covers one half of the distance in 2/3rd time what should be his speed to cover the remaining distance in the remaining time
View SolutionA person wishes to reach his destination 90 km away in three hours but for the first half of the journey his speed was 20 km/hr. His average speed for the rest of the journey should be
View SolutionVishal covers a distance of 20 km in 30 min. If he covers half of the distance in 18 min, what should be his speed to cover the remaining distance and completes the whole journey in 30 min.
View SolutionIf Abhi travels a certain distance at 6 km/h. he reaches his destination 12 minutes early, but if he travels at 4 km/h, he reaches his destination 10 minutes late. The speed (in km/h) at which he should travel to reach his destination on time is ........
View SolutionIf Ramu travelled 25% slower than his usual speed, then he would reach his destination 2 h late. Find the usual time taken by him to reach his destination (in hours )
View SolutionIf a train runs with the speed of 52km/h, it reaches its destination late by 15 minutes. However, if its speed is 65km/h, it is late by 5 minutes only. The right time for the train to cover its journey is:
यदि कोई ट्रेन 52 किमी/घंटा की चाल से चलती है, तो यह अपने गंतव्य स्थल पर 15 मिनट की देरी से पहुँचती है | लेकिन, जब इसकी चाल 65 किमी/घंटा होती है, तो यह केवल 5 मिनट देर करती है | ट्रेन को यात्रा पूर्ण करने में लगने वाला सही समय क्या है?View Solution