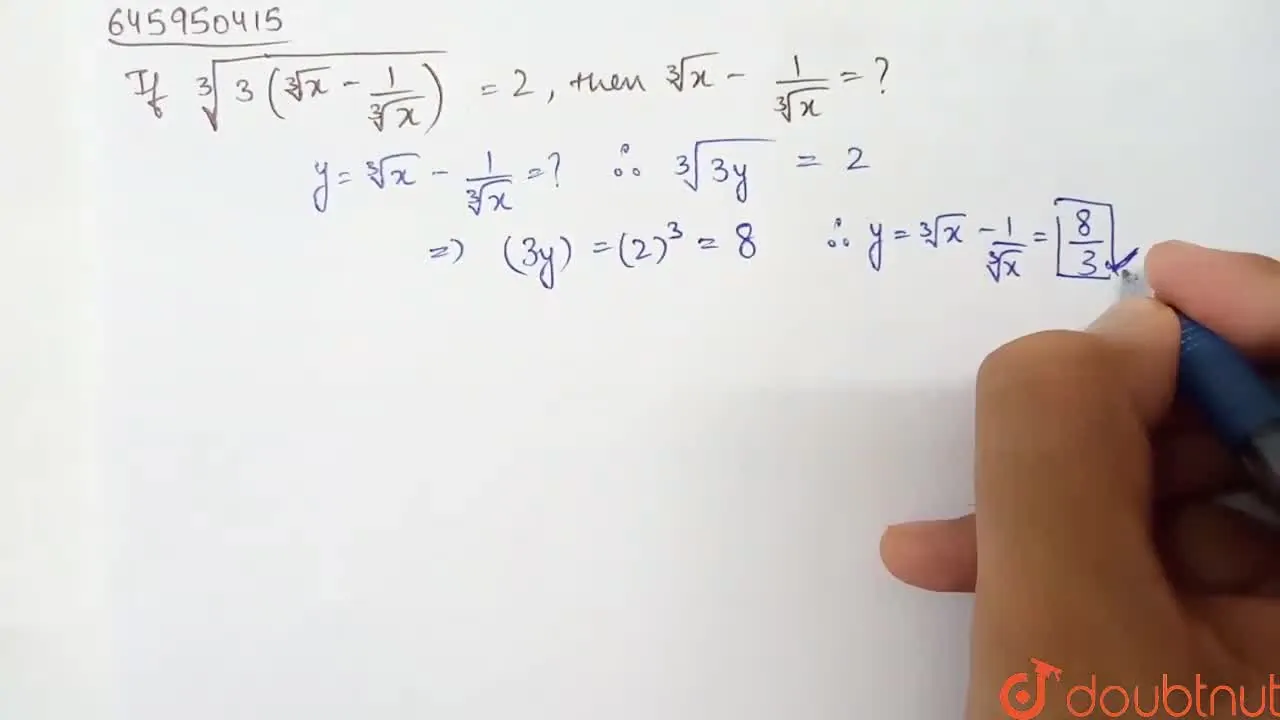If 3 ⎷3(3√x−13√x)=2 then 3√x−13√x=………..
Video Solution
|
Answer
Step by step video, text & image solution for If root3(3(root(3)(x)-1/(root3(x))))=2" then "root3(x)-1/(root3(x))=……….. by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023
Similar Questions
If 3 ⎷3(3√x−13√x)=2 then 3√x−13√x=………..
View SolutionIf 3 ⎷3(3√x−13√x)=2,then 3√x−13√x
View Solution3√x2+3√x2=3
View Solution3√sin2x
View Solution∫ (3√x)(5√1+3√x4)dx
View Solution(3√3+3√2)(3√9+3√4−3√6)=
View SolutionIf x=3√a−13√a then value of x3+3x is
View Solution3√1.728−3√0.2163√2.197−3√0.343=
View SolutionFor x>0 ,3√x×3√x×3√x=
View Solutionlimx→∞3√x(3√(x+1)2−3√(x−1)2)=
View Solution