Infinite number of images is formed when two plane mirrors placed
Step-by-Step Solution:
1. Understanding the Setup: We start with two plane mirrors. To create an infinite number of images, these mirrors must be placed parallel to each other.
2. Positioning the Mirrors: Assume we have two mirrors, Mirror 1 and Mirror 2, positioned parallel to each other.
3. Placing the Object: Let's place an object in front of these two mirrors. For example, we can place a candle or any other object.
4. First Reflection: The light from the object will hit Mirror 1 and reflect back. This reflection creates the first image (Image 1) behind Mirror 1.
5. Second Reflection: The light from the object will also hit Mirror 2 and reflect back, creating the second image (Image 2) behind Mirror 2.
6. Subsequent Reflections: Each of these images will act as new objects for the opposite mirror. For example, Image 1 will reflect back from Mirror 2, creating a new image (Image 2.1) behind Mirror 2. Similarly, Image 2 will reflect back from Mirror 1, creating another new image (Image 1.1) behind Mirror 1.
7. Continuing the Process: This process continues indefinitely. Each image created will reflect back and create another image in the opposite mirror, leading to an infinite series of images.
8. Conclusion: Therefore, when two plane mirrors are placed parallel to each other, they create an infinite number of images of the object placed between them.
---
Topper's Solved these Questions
- LIGHTBook:PEARSON IIT JEE FOUNDATIONChapter:LIGHTExercise:ASSESSMENT TEST
Explore 30 Videos
- Force and Pressure Book:PEARSON IIT JEE FOUNDATIONChapter:Force and Pressure Exercise:CROSSWORD
Explore 1 Video
- MEASUREMENTS AND MOTIONBook:PEARSON IIT JEE FOUNDATIONChapter:MEASUREMENTS AND MOTIONExercise:CROSSWORD
Explore 2 Videos
Similar Questions
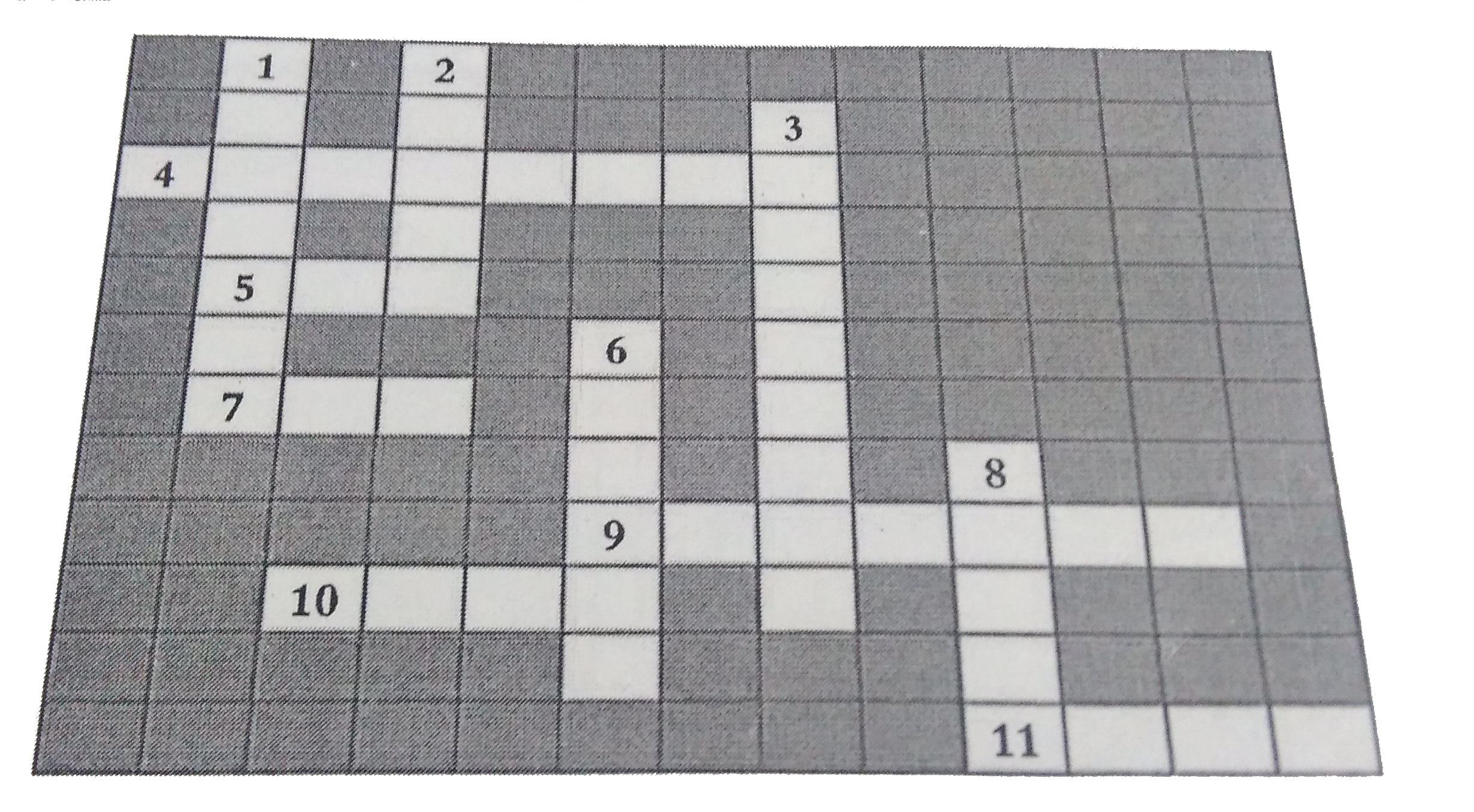
AcrossDown4.Infinite number of images in formed when two plane mirrors placed1.These are formed due to rectilinear propagation of light5.Magnification of cancave mirror when the object is placed at the centre of curvature2. This mirror forms laterally inverted image7.Example of hot source of light 3.Example of natural source of light 9.Convex mirrror can form this image6. This mirror can form a diminihed virtual image10.Number of images formed when an object placed between two plane mirrors inclined by60∘8.Eclipse formed when earth comes between moon and sun11.Image formed due to convergence of light
View SolutionFind the number of images formed when two plane mirrors are placed at E right angle to each other & locate the images by drawing ray diagram.
View Solution
Knowledge Check
The total number of images formed by two mirrors inclined at 120∘ to each other is ______.
A6B36C5D4The number of images formed by two plane mirrors inclined at 60∘ of an object placed symmetrically between mirror is
A6B7C5DinfiniteThe total number of images formed by two plane mirrors , inclined at an angle of 15∘ and an objectvitying symmetrically is
A24B23C22D25
Write the mathematical expression to calculate the number of image formed by two plne mirrors placed symmetrically at an angle 'θ'.
View SolutionNumber of images formed by two inclined plane mirrors
View SolutionHow many images are formed when two plane mirrors are placed parallel to each other?
View SolutionThe nature of the image formed by a plane mirror is
View SolutionThe total number of image formed by two plane mirrors, inclined at an angle of 112.5∘ and an object lying symmetrically is
View Solution