Consider the following reaction which proceeds in a closed vessel.
3X→2Y+Z
The rate of disappearance of X,−Δ[X]Δt is found to be 0.075 mol
L−s− calculate Δ[y]ΔtandΔ[Z]Δt
The correct Answer is:0.05molL−s−,0.025molL−s−1
To solve the problem, we need to analyze the stoichiometry of the given reaction and apply the relationship between the rates of disappearance and appearance of the reactants and products.
1. Write the Reaction:
The reaction is given as:
3X→2Y+Z
2. Identify the Rate of Disappearance of X:
We are given that the rate of disappearance of X is:
−Δ(X)Δt=0.075mol L−1s−1
3. Relate the Rate of Disappearance of X to the Rate of Appearance of Y and Z:
From the stoichiometry of the reaction, we can express the rates of change of concentrations as follows:
−13Δ(X)Δt=12Δ(Y)Δt=11Δ(Z)Δt
4. Calculate the Rate of Appearance of Y:
Using the relationship for Y:
Δ(Y)Δt=−23Δ(X)Δt
Substituting the value of −Δ(X)Δt:
Δ(Y)Δt=−23×(−0.075)=2×0.0753=0.153=0.05mol L−1s−1
5. Calculate the Rate of Appearance of Z:
Using the relationship for Z:
Δ(Z)Δt=−13Δ(X)Δt
Substituting the value of −Δ(X)Δt:
Δ(Z)Δt=−13×(−0.075)=0.0753=0.025mol L−1s−1
Final Answers:
- The rate of appearance of Y is:
Δ(Y)Δt=0.05mol L−1s−1
- The rate of appearance of Z is:
Δ(Z)Δt=0.025mol L−1s−1
The reaction is given as:
3X→2Y+Z
We are given that the rate of disappearance of X is:
−Δ(X)Δt=0.075mol L−1s−1
From the stoichiometry of the reaction, we can express the rates of change of concentrations as follows:
−13Δ(X)Δt=12Δ(Y)Δt=11Δ(Z)Δt
Using the relationship for Y:
Δ(Y)Δt=−23Δ(X)Δt
Substituting the value of −Δ(X)Δt:
Δ(Y)Δt=−23×(−0.075)=2×0.0753=0.153=0.05mol L−1s−1
Using the relationship for Z:
Δ(Z)Δt=−13Δ(X)Δt
Substituting the value of −Δ(X)Δt:
Δ(Z)Δt=−13×(−0.075)=0.0753=0.025mol L−1s−1
- The rate of appearance of Y is:
Δ(Y)Δt=0.05mol L−1s−1
- The rate of appearance of Z is:
Δ(Z)Δt=0.025mol L−1s−1
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - INTEXT QUESRTIONS
81 Videos
- CHEMICAL KINETICSBOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - EXERCISE (PART- I (OBJECTIVE QUESRTIONS)A.FILL IN THE BLANKS)
54 Videos
- CHEMICAL KINETICSBOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - ISC EXAMINATION QUESTIONS (NUMERICAL PROBLEMS )
57 Videos
- BIOMOLECULESBOOK - ICSECHAPTER - BIOMOLECULESEXERCISE - ISC EXAMINATION QUSTIONS (PART-I)(DESCRIPTIVE QUESTIONS)
21 Videos
- CHEMISTRY IN EVERYDAY LIFEBOOK - ICSECHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - EXERCISE (PART - II (DESCRIPTIVE QUESTIONS) (LONG ANSWER QUESTIONS))
19 Videos
Similar Questions
Comment on the spontaneity of a process when
ΔH>0,TΔS<0
View SolutionThe drift speed is defined as vd=(Δl/Δt)where (Δl)isthedistancedrifted∈alongtime(Delta t).Whydon'twedef∈ethedriftspeedasthelimitof(Delta l//Delta t)as (Delta t (rarr)0)?
View Solution1 mol each of the following compounds is dissolved in 1 L of
solution. Which will have the largest ΔTb value ?
View SolutionIf the equations of three sides of a triangle are x+y=1,3x+5y=2andx−y=0 then the orthocentre of the triangle lies on the line/lines
View SolutionCorrect relation for isothermal reversible expansion of an ideal gas is ΔU=0, ΔS=0,ΔG=−TΔS, ΔH=0
View Solution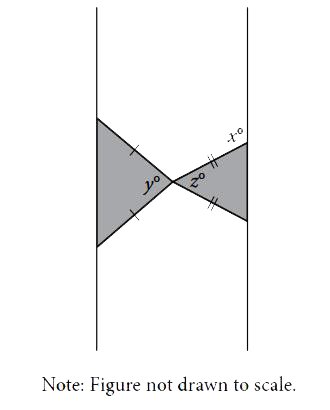
Two isosceles triangle are shown above. If 180-z=2y and y=75, what is the value of x?
View SolutionEnthalpy of formation of NH3 is -X kJ and ΔHH−H, ΔHN−H are respectively Y kJmol−1 and Z kjmol−1. The value of ΔHN=Nis
View SolutionThe equation of the sides of a triangle are x+2y+1=0,2x+y+2=0andpx+qy+1=0 and area of triangle is Δ .
View SolutionThe coordinates of the vertices of a triangle are (4, -3), (-5, 2) and (x, y). If the centroid of the triangle is at the origin, show that x=y=1.
View SolutionDraw the graphs of the following equations: 2x−3y+6=0, 2x+3y−18=0, y−2=0 Find the vertices of the triangle so obtained. Also, find the area of the triangle.
View Solution
