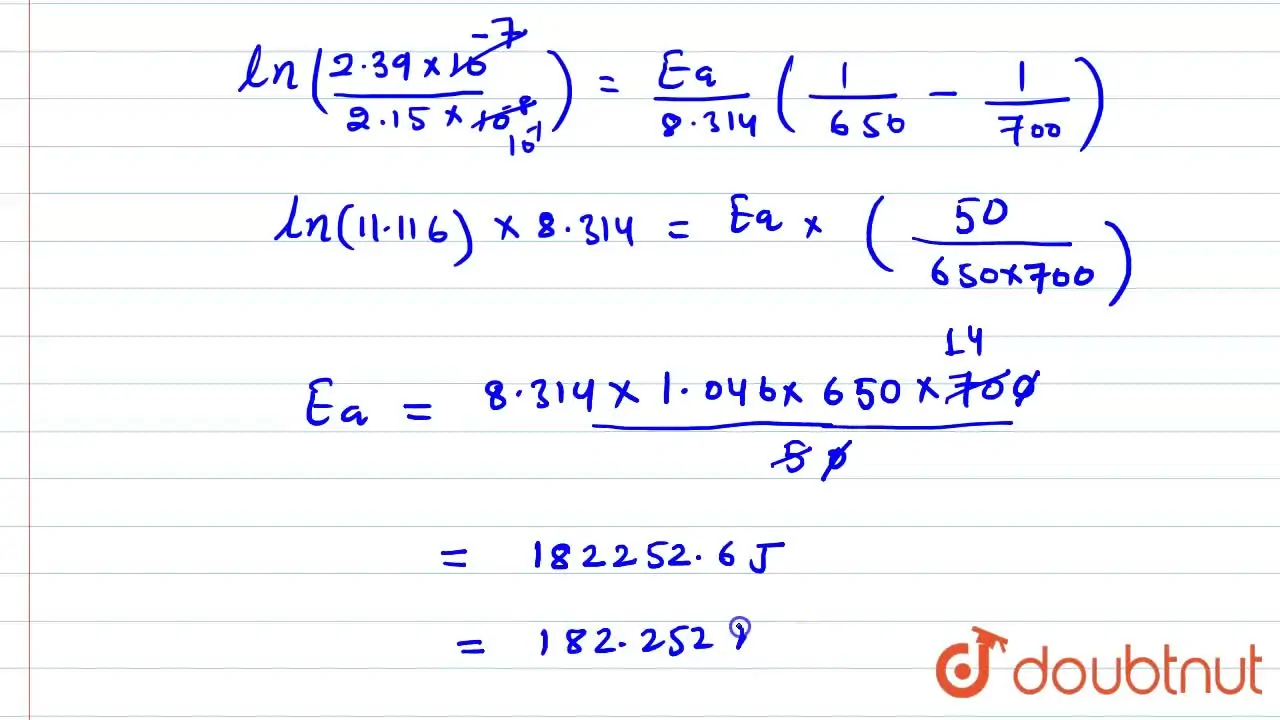
For a decomposition reaction, the values of rate constant k at two different temperatures are given below:
K1=2.15×10−8Lmol−1s−1at650K
K2=2.39×10−7Lmols−1at700K
Calculate the value of activation energy for this reaction. (R = 8.314JK−1mol−1)
Applying Arrhenius equation,
logk2k1=Ea2.303R[T2−T1]T1T2
Substituting the values
log(2.39×10−7)2.15×10−8=Ea2.303×8.314[400−650650×700]
orlog11.116=Ea19.147×50455000
or1.046=Ea19.147×50455000
or1.046=Ea19.147×50455000
orEa=1.046×19.147×45500050=182252.6J=182.25KJ
Related Playlists
- BOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - PROBLEM165videos
CHEMICAL KINETICS
- BOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - INTEXT QUESRTIONS81videos
CHEMICAL KINETICS
- BOOK - ICSECHAPTER - BIOMOLECULESEXERCISE - ISC EXAMINATION QUSTIONS (PART-I)(DESCRIPTIVE QUESTIONS)21videos
BIOMOLECULES
- BOOK - ICSECHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - EXERCISE (PART - II (DESCRIPTIVE QUESTIONS) (LONG ANSWER QUESTIONS))19videos
CHEMISTRY IN EVERYDAY LIFE
Similar Questions
For a decomposition reaction the values of rate constant k at two different temperatures are given below :
K1=2.15×10−8Lmol−1s−1at 650K
K2=2.39×10−7Lmol−1s−1at 700K
Calclate the value of activation energy for this reaction.
(R=8.314JK−1mol−1)View SolutionFor a decomposition reaction the values of rate constant k at two different temperatures are given below :
K1=2.15×10−8Lmol−1s−1at 650K
K2=2.39×10−7Lmol−1s−1at 700K
Calclate the value of activation energy for this reaction.
(R=8.314JK−1mol−1)View SolutionThe rate constant of a reaction is 1.5×107s−1 at 50∘C and 4.5×107s−1 at 100∘C. Calculate the value of activation energy for the reaction (R=8.314JK−1mol−1)
View SolutionRate constant of two reactions are given below. Identifying their order of reaction
k=5.3×10−2Lmol−1s−1
k=3.8×10−4s−1View SolutionRate constant of two reactions are given below. Indentifying their order of reaction.
(i) k=6.3×10−2Lmol−1s−1
(ii) k=2.8×10−4s−1View SolutionA first order reaction is 50% completed in 30 minutes at 300 K and in 10 minutes at 320 K, Calculate the activation energy of the reaction (R = 8.314 JK mol−1.)
View SolutionFor a reaction, K=1.958×10−4 at 400 K, what is the value of ΔG∘at this temepratuure ?(R=8.314JK−1mol−1).
View SolutionThe kinetic energy of two moles of N2at27∘Cis(R=8.314JK−1mol−1)
View SolutionFor a reaction, Kp=1.8×10−7at 300K. What is the value of ΔG∘ at this temeprature ?
(R=8.314JK−1mol−1)View SolutionThe value of rate constant for a second order reaction is 6.7×10−5mol−Ls− at 298 K and 1.64×10−4mol−Ls− at 313 K. Find the Arrhenius frequency factor A and activation energy of the reaction.
View Solution